Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Helminck, Aloysius G.
2000.
Computing Orbits of Minimal Parabolic k -subgroups Acting on Symmetric k -varieties.
Journal of Symbolic Computation,
Vol. 30,
Issue. 5,
p.
521.
Brown, Ronald
Ghani, Neil
Heyworth, Anne
and
Wensley, Christopher D.
2006.
String rewriting for double coset systems.
Journal of Symbolic Computation,
Vol. 41,
Issue. 5,
p.
573.
Gille, Philippe
and
Pianzola, Arturo
2007.
Galois cohomology and forms of algebras over Laurent polynomial rings.
Mathematische Annalen,
Vol. 338,
Issue. 2,
p.
497.
Nishiyama, Kyo
2009.
Resolution of null fiber and conormal bundles on the Lagrangian Grassmannian.
Geometriae Dedicata,
Vol. 143,
Issue. 1,
p.
19.
Beun, Stacy L.
and
Helminck, Aloysius G.
2009.
On the Classification of Orbits of Symmetric Subgroups Acting on Flag Varieties of SL(2,k).
Communications in Algebra,
Vol. 37,
Issue. 4,
p.
1334.
Helminck, A. G.
2010.
Symmetry and Spaces.
Vol. 278,
Issue. ,
p.
83.
Springer, T.A.
2011.
Decompositions related to symmetric varieties.
Journal of Algebra,
Vol. 329,
Issue. 1,
p.
260.
Huang, Huajun
and
He, Hongyu
2011.
Symmetric subgroup actions on isotropic Grassmannians.
Journal of Algebra,
Vol. 337,
Issue. 1,
p.
141.
Ciubotaru, Dan
Nishiyama, Kyo
and
Trapa, Peter E.
2012.
Representation Theory, Complex Analysis, and Integral Geometry.
p.
61.
HE, XUHUA
OCHIAI, HIROYUKI
NISHIYAMA, KYO
and
OSHIMA, YOSHIKI
2013.
On Orbits in Double Flag Varieties for Symmetric Pairs.
Transformation Groups,
Vol. 18,
Issue. 4,
p.
1091.
Colarusso, Mark
and
Evens, Sam
2014.
Symmetry: Representation Theory and Its Applications.
Vol. 257,
Issue. ,
p.
85.
KONDO, Kensuke
NISHIYAMA, Kyo
OCHIAI, Hiroyuki
and
TANIGUCHI, Kenji
2014.
CLOSED ORBITS ON PARTIAL FLAG VARIETIES AND DOUBLE FLAG VARIETY OF FINITE TYPE.
Kyushu Journal of Mathematics,
Vol. 68,
Issue. 1,
p.
113.
Colarusso, Mark
and
Evens, Sam
2015.
Eigenvalue coincidences and K-orbits, I.
Journal of Algebra,
Vol. 422,
Issue. ,
p.
611.
Buell, Catherine
Klima, Vicky
Schaefer, Jennifer
Wright, Carmen
and
Ziliak, Ellen
2018.
Advances in the Mathematical Sciences.
Vol. 15,
Issue. ,
p.
69.
Chen, Tsao-Hsien
Vilonen, Kari
and
Xue, Ting
2018.
Springer correspondence for the split symmetric pair in type .
Compositio Mathematica,
Vol. 154,
Issue. 11,
p.
2403.
Collins, J. B.
Haas, Ruth
Helminck, Aloysius G.
Lenarz, Jessie
Pelatt, Kristine Engel
Saccon, Silvia
and
Welz, Matthew
2020.
Extended symmetric spaces and θ-twisted involution graphs.
Communications in Algebra,
Vol. 48,
Issue. 6,
p.
2293.
Buell, C.
Helminck, A.
Klima, V.
Schaefer, J.
Wright, C.
and
Ziliak, E.
2020.
Classifying the orbits of the generalized symmetric spaces for SL2(Fq).
Communications in Algebra,
Vol. 48,
Issue. 4,
p.
1744.
Hunnell, Mark
2021.
Minimal parabolic k-subgroups acting on symmetric k-varieties corresponding to k-split groups.
Journal of Algebra and Its Applications,
Vol. 20,
Issue. 11,
Colarusso, Mark
and
Evens, Sam
2022.
B−1-bundles on the flag variety, I.
Journal of Algebra,
Vol. 596,
Issue. ,
p.
128.
Buell, Catherine
Helminck, Aloysius
Klima, Vicky
Schaefer, Jennifer
Wright, Carmen
and
Ziliak, Ellen
2024.
Fixed-point group conjugacy classes of unipotent elements in low-dimensional symmetric spaces of special linear groups over a finite field.
Journal of Algebra and Its Applications,
Vol. 23,
Issue. 09,
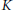 $K$-orbits in
$K$-orbits in 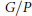 $G/P$ where
$G/P$ where 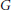 $G$ is a complex connected reductive group,
$G$ is a complex connected reductive group,  $P\,\subseteq \,G$ is a parabolic subgroup, and
$P\,\subseteq \,G$ is a parabolic subgroup, and 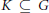 $K\,\subseteq \,G$ is the fixed point subgroup of an involutive automorphism
$K\,\subseteq \,G$ is the fixed point subgroup of an involutive automorphism 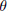 $\theta$. Generalizing work of Springer, we parametrize the (finite) orbit set
$\theta$. Generalizing work of Springer, we parametrize the (finite) orbit set 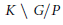 $K\,\backslash \,G/P$ and we determine the isotropy groups. As a consequence, we describe the closed (resp. affine) orbits in terms of
$K\,\backslash \,G/P$ and we determine the isotropy groups. As a consequence, we describe the closed (resp. affine) orbits in terms of 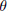 $\theta$-stable (resp.
$\theta$-stable (resp. 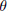 $\theta$-split) parabolic subgroups. We also describe the decomposition of any
$\theta$-split) parabolic subgroups. We also describe the decomposition of any 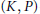 $(K,\,P)$-double coset in
$(K,\,P)$-double coset in 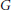 $G$ into
$G$ into 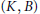 $(K,\,B)$-double cosets, where
$(K,\,B)$-double cosets, where  $B\,\subseteq \,P$ is a Borel subgroup. Finally, for certain
$B\,\subseteq \,P$ is a Borel subgroup. Finally, for certain 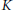 $K$-orbit closures
$K$-orbit closures 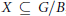 $X\,\subseteq \,G/B$, and for any homogeneous line bundle
$X\,\subseteq \,G/B$, and for any homogeneous line bundle 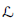 $\mathcal{L}$ on
$\mathcal{L}$ on 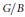 $G/B$ having nonzero global sections, we show that the restriction map
$G/B$ having nonzero global sections, we show that the restriction map 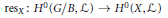 $\text{re}{{\text{s}}_{X}}\,:\,{{H}^{0}}\,\left( G\,/\,B,\,\mathcal{L} \right)\,\to \,{{H}^{0}}\,\left( X,\,\mathcal{L} \right)$ is surjective and that
$\text{re}{{\text{s}}_{X}}\,:\,{{H}^{0}}\,\left( G\,/\,B,\,\mathcal{L} \right)\,\to \,{{H}^{0}}\,\left( X,\,\mathcal{L} \right)$ is surjective and that 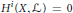 ${{H}^{i}}\,\left( X,\mathcal{L} \right)\,=\,0$ for
${{H}^{i}}\,\left( X,\mathcal{L} \right)\,=\,0$ for 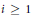 $i\,\ge \,1$. Moreover, we describe the
$i\,\ge \,1$. Moreover, we describe the 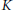 $K$-module
$K$-module 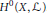 ${{H}^{0}}\left( X,L \right)$. This gives information on the restriction to
${{H}^{0}}\left( X,L \right)$. This gives information on the restriction to 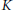 $K$ of the simple
$K$ of the simple 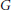 $G$-module
$G$-module 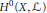 ${{H}^{0}}\,\left( G\,/\,B,\mathcal{L} \right)$. Our construction is a geometric analogue of Vogan and Sepanski’s approach to extremal
${{H}^{0}}\,\left( G\,/\,B,\mathcal{L} \right)$. Our construction is a geometric analogue of Vogan and Sepanski’s approach to extremal 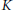 $K$-types.
$K$-types.

