Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-29T02:05:30.684Z Has data issue: false hasContentIssue false
  • English
  • Français

On Local L-Functions and Normalized Intertwining Operators

Published online by Cambridge University Press:  20 November 2018

Henry H. Kim
Henry H. Kim*
Affiliation:
Deptartment of Mathematics, University of Toronto, Toronto, ON M5S 3G3, e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this paper we make explicit all $L$-functions in the Langlands–Shahidi method which appear as normalizing factors of global intertwining operators in the constant term of the Eisenstein series. We prove, in many cases, the conjecture of Shahidi regarding the holomorphy of the local $L$-functions. We also prove that the normalized local intertwining operators are holomorphic and non-vaninishing for 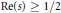$\operatorname{Re}\left( s \right)\,\ge \,1/2$ in many cases. These local results are essential in global applications such as Langlands functoriality, residual spectrum and determining poles of automorphic $L$-functions.

Keywords

11F7022E55
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 57 , Issue 3 , 01 June 2005 , pp. 535 - 597
Copyright
Copyright © Canadian Mathematical Society 2005

References

[A] Arthur, J. Intertwining operators and residues I. Weighted characters. J. Funct. Anal. 84(1989), 19–84.Google Scholar
[As] Asgari, M. Local L–functions for split spinor groups. Canad. J. Math. 54(2002), 673–693.Google Scholar
[Bo] Borel, A., Automorphic L–functions. In: Automorphic Forms and Automorphic Representations, Proc. Sympos. Pure Math. 33, American Mathematical Society, Providence, RI, 1979, pp. 27–61.Google Scholar
[Bou] Bourbaki, N., Groupes et algébres de Lie., Chap. 4-6, Paris: Hermann, 1968.Google Scholar
[Ca-Sh] Casselman, W. and Shahidi, F., On irreducibility of standard modules for generic representations. Ann. Sci. École Norm. Sup. 31(1998), 561–589.Google Scholar
[CKPSS] Cogdell, J., Kim, H., Piatetski-Shapiro, I. I., and Shahidi, F., On lifting from classical groups to GLN. Publ. Math. Inst. Hautes Études Sci. 93(2001), 5–30.Google Scholar
[G-O-V] Gorbatsevich, V. V., Onishchik, A. L. and Vinberg, E. B., Lie Groups and Lie Algebras III. In: Encyclopedia of Mathematical Sciences, Vol 41, Springer-Verlag, 1990.Google Scholar
[Ja1] Jantzen, C., Degenerate principal series for orthogonal groups. J. Reine Angew.Math.441(1993), 61–98.Google Scholar
[Ja2] Jantzen, C., On supports of induced representations for symplectic and odd-orthogonal groups. Amer. J. Math. 119(1997), 1213–1262 .Google Scholar
[Ja3] Jantzen, C., On square-integrable representations of classical p-adic groups. Can. J. Math. 52(2000), 539–581.Google Scholar
[Ki1] Kim, H., The residual spectrum of Sp4. Comp. Math. 99(1995), 129–151 Google Scholar
[Ki2] Kim, H., The residual spectrum of G2. Can. J. Math. 48(1996), 1245–1272.Google Scholar
[Ki3] Kim, H., Langlands-Shahidi method and poles of automorphic L-functions: application to exterior square L-functions. Can. J. Math. 51(1999), 835–849.Google Scholar
[Ki4] Kim, H., Langlands-Shahidi method and poles of automorphic L-functions II. Israel J. Math. 117(2000), 261–284.Google Scholar
[Ki5] Kim, H., Functoriality for the exterior square of GL4 and the symmetric fourth of GL2. J. Amer. Math. Soc. 16(2003), 139–183.Google Scholar
[Ki-Sh] Kim, H. and Shahidi, F., Functorial products for GL2 × GL3 and symmetric cube for GL2 . Ann. of Math. 155(2002), 837–893.Google Scholar
[Ki-Sh2] Kim, H. and Shahidi, F., Cuspidality of symmetric powers with applications. Duke Math. J. 112(2002), 177–197.Google Scholar
[Ko] Kottwitz, R. E., Stable trace formula: cuspidal tempered terms. Duke Math. J. 51(1984), 611–650.Google Scholar
[Ku] Kudla, S., The local Langlands correspondence: the non-archimedean case. In: Proceedings of Symposia in Pure Mathematics 55, American Mathematical Society, Providence, RI, 1994, pp. 365–391.Google Scholar
[La] Langlands, R. P., Euler Products. Yale University Press, New Haven, CN, 1971.Google Scholar
[La2] Langlands, R. P., On the Functional Equations Satisfied by Eisenstein Series. Lecture Notes in Mathematics vol. 544, Springer-Verlag, Berlin, 1976.Google Scholar
[M-W1] Moeglin, C. and Waldspurger, J. L., Spectral Decomposition and Eisenstein series, une paraphrase de l’Ecriture. Cambridge Tracts in Mathematics 113, Cambridge, Cambridge University Press, 1995.Google Scholar
[M-W2] Moeglin, C. and Waldspurger, J. L., Le spectre résiduel de GL(n). Ann. Scient. Éc. Norm. Sup. 22(1989), 605–674.Google Scholar
[M-Ta] Moeglin, C. and Tadic, M., Construction of discrete series for classical p-adic groups. J. Amer. Math. Soc. 15(2002), 715–786 .Google Scholar
[Mu1] Muić, G., Some results on square integrable representations; irreducibility of standard representations. Internat.Math. Res. Notices 14(1998), 705–726.Google Scholar
[Mu2] Muić, G., A proof of Casselman–Shahidi's conjecture for quasi-split classical groups. Canad. Math. Bull. 44(2001), 298–312.Google Scholar
[Ra] Ramakrishnan, D., Modularity of the Rankin-Selberg L-series, and multiplicity one for SL(2). Ann. of Math. 152(2000), 45–111.Google Scholar
[Sh1] Shahidi, F., A proof of Langlands conjecture on Plancherel measures; complementary series for p-adic groups. Annals of Math. 132(1990), 273–330.Google Scholar
[Sh2] Shahidi, F., On certain L-functions. Amer. J. Math. 103(1981), 297–355.Google Scholar
[Sh3] Shahidi, F., On the Ramanujan conjecture and finiteness of poles for certain L-functions. Ann. of Math. 127(1988), 547–584.Google Scholar
[Sh4] Shahidi, F., On non-vanishing of twisted symmetric and exterior square L-functions for GL(n). Pacific J. Math. Olga Taussky-Todd memorial issue (1997), 311–322.Google Scholar
[Sh5] Shahidi, F., Fourier transforms of intertwining operators and Plancherel measures for GL(n). Amer. J. Math. 106(1984), 67–111 .Google Scholar
[Sh6] Shahidi, F., On multiplicativity of local factors. In: Festschrift in honor of Piatetski-Shapiro, I. I., Part II, Israel Math. Conf. Proc. 3,Weizmann, Jerusalem, 1990, pp. 279–289.Google Scholar
[Sh7] Shahidi, F., Local coefficients as Artin factors for real groups. Duke Math. J. 52(1985), 973–1007.Google Scholar
[Ta] Tadic, M., Classification of unitary representations in irreducible representations of general linear group (non-Archimedean case. Ann. Sci. Ec. Norm. Sup. 19(1986), 335–382.Google Scholar
[V] Vogan, D., Gelfand-Kirillov dimension for Harish-Chandra modules. Invent.Math. 48(1978), 75–98.Google Scholar
[Ze] Zelevinsky, A.V., Induced representations of reductive p-adic groups II. On irreducible representations of GL(n). Ann. Sci. École Norm. Sup. 13(1980), 165–210.Google Scholar
[Zh] Zhang, Y., The holomorphy and nonvanishing of normalized local intertwining operators. Pacific J. Math. 180(1997), 385–398.Google Scholar