Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-27T16:17:05.419Z Has data issue: false hasContentIssue false
  • English
  • Français

On a Sumset Conjecture of Erdős

Published online by Cambridge University Press:  20 November 2018

Mauro Di Nasso ,
Isaac Goldbring ,
Renling Jin ,
Steven Leth ,
Martino Lupini  and
Karl Mahlburg
Mauro Di Nasso
Affiliation:
Dipartimento di Matematica, Universita' di Pisa, Largo Bruno Pontecorvo 5, Pisa 56127, Italy e-mail: [email protected]
Isaac Goldbring
Affiliation:
Department of Mathematics, Statistics, and Computer Science, University of Illinois at Chicago, Chicago, IL 60607-7045, USA e-mail: [email protected]
Renling Jin
Affiliation:
Department of Mathematics, College of Charleston, Charleston, SC 29424, USA e-mail: [email protected]
Steven Leth
Affiliation:
School of Mathematical Sciences, University of Northern Colorado, Greeley, CO 80639, USA e-mail: [email protected]
Martino Lupini
Affiliation:
Department of Mathematics and Statistics, York University, Toronto, ON M3J 1P3 e-mail: [email protected]
Karl Mahlburg
Affiliation:
Department of Mathematics, Louisiana State University, Baton Rouge, LA 70803, USA e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Erdős conjectured that for any set $A\,\subseteq \,\mathbb{N}$ with positive lower asymptotic density, there are infinite sets 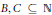$B,\,C\,\subseteq \,\mathbb{N}$ such that 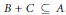$B\,+\,C\,\subseteq \,A$. We verify Erdős’ conjecture in the case where 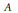$A$ has Banach density exceeding 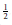$\frac{1}{2}$. As a consequence, we prove that, for $A\,\subseteq \,\mathbb{N}$ with positive Banach density (a much weaker assumption than positive lower density), we can find infinite 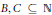$B,\,C\,\subseteq \,\mathbb{N}$ such that 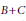$B\,+\,C$ is contained in the union of 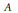$A$ and a translate of 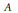$A$. Both of the aforementioned results are generalized to arbitrary countable amenable groups. We also provide a positive solution to Erdős’ conjecture for subsets of the natural numbers that are pseudorandom.

Keywords

11B0511B1311P7028D1537A45sumsets of integersasymptotic densityamenable groupsnonstandard analysis
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 67 , Issue 4 , 01 August 2015 , pp. 795 - 809
Copyright
Copyright © Canadian Mathematical Society 2015

References

[1] Beiglbock, M., Bergelson, V., and Fish, A., Sumset phenomenon in countable amenable groups. Adv. Math. 223(2010), no. 2, 416–432. http://dx.doi.org/10.1016/j.aim.2009.08.009 Google Scholar
[2] Bergelson, V., Sets of recurrence of ℤm-actions and properties of sets of differences in ℤm. J. London Math. Soc. (2) 31(1985), no. 2, 295–304.http://dx.doi.org/10.1112/jlms/s2-31.2.295 Google Scholar
[3] Di Nasso, M., Embeddability properties of difference sets. arxiv:1201.5865Google Scholar
[4] Di Nasso, M. and Lupini, M., Nonstandard analysis and the sumset phenomenon in arbitrary amenable. arxiv:1211.4208Google Scholar
[5] Erdös, P., Problems and results in combinatorial number theory. Astérisque 24–25(1975), 295–309.Google Scholar
[6] Erdös, P., Problems and results on combinatorial number theory. III. In; Number theory day (Proc. Conf., Rockefeller Univ., New York, 1976), Lecture Notes in Math., 626, Springer, Berlin, 1977, pp. 43–72.Google Scholar
[7] Erdös, P., A survey of problems in combinatorial number theory. Combinatorial mathematics, optimal designs and their applications (Proc. Sympos. Combin. Math. and Optimal Design, Colorado State Univ., Fort Collins, Colo., 1978). Ann. Discrete Math. 6(1980), 89–115.http://dx.doi.org/10.1016/S0167-5060(08)70697-6 Google Scholar
[8] Erdös, P. and Graham, R. L., Old and new problems and results in combinatorial number theory. Monographies de L’Enseignement Mathématique, 28. Universitè de Genve, L’Enseignement Mathématique, Geneva, 1980.Google Scholar
[9] Furstenberg, H., Ergodic behavior of diagonal measures and a theorem of Szemerédi on arithmetic progressions. J. Analyse Math. 31(1977), 204–256. http://dx.doi.org/10.1007/BF02813304 Google Scholar
[10] Hindman, N., Finite sums from sequences within cells of a partition of N. J. Combinatorial Theory Ser. A 17(1974), 1–11.http://dx.doi.org/10.1016/0097-3165(74)90023-5 Google Scholar
[11] Hindman, N., On density, translates, and pairwise sums of integers. J. Combin. Theory Ser. A 33(1982), no. 2, 147–157.http://dx.doi.org/10.1016/0097-3165(82)90003-6 Google Scholar
[12] Jin, R., An introduction of nonstandard methods for number theorists. Integers 8(2008), no. 2, A7.Google Scholar
[13] Krengel, U., Ergodic theorems. de Gruyter Studies in Mathematics, 6,Walter de Gruyter & Co., Berlin, 1985.Google Scholar
[14] Lindenstrauss, E., Pointwise theorems for amenable groups. Invent. Math. 146(2001), no. 2, 259–295.http://dx.doi.org/10.1007/s002220100162 Google Scholar
[15] Nathanson, M. B., Sumsets contained in infinite sets of integers. J. Combin. Theory Ser. A 28(1980), no. 2, 150–155.http://dx.doi.org/10.1016/0097-3165(80)90081-3 Google Scholar
[16] Pogorzelski, F. and Schwarzenberger, F., A Banach space-valued ergodic theorem for amenable groups and applications. arxiv:1205.3649Google Scholar
[17] Szemerédi, E., On sets of integers containing no k elements in arithmetic progression. Acta Arith. 27(1975), 199–245.Google Scholar
[18] van derWaerden, B. L., Beweis einer Baudetschen Vermutung. Nieuw Arch.Wisk. 15(1927), 212–216.Google Scholar