Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-12-02T23:53:42.825Z Has data issue: false hasContentIssue false
  • English
  • Français

A Note on Certain Spaces with Bases (mod K)

Published online by Cambridge University Press:  20 November 2018

Harold R. Bennett  and
Harold W. Martin
Harold R. Bennett
Affiliation:
Texas Tech University, Lubbock, Texas
Harold W. Martin
Affiliation:
Texas Tech University, Lubbock, Texas
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In this note all spaces are assumed to be regular T1 spaces and all undefined terms and notations may be found in [8], In particular let cl(A) denote the closure of the set A and let Z+ denote the set of natural numbers.

Definition 1. Let X be a topological space and a covering of X by compact sets. An open covering 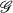of X is said to be a basis (mod K) if whenever 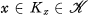and an open set V contains Kx, then there exists such that 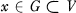. In such a case X is written as the ordered triple .

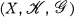Type
Research Article
Information
Canadian Journal of Mathematics , Volume 27 , Issue 2 , 01 April 1975 , pp. 469 - 474
Copyright
Copyright © Canadian Mathematical Society 1975

References

1. Arhangel'skiï, A. V., On a class of spaces containing all metric and all locally bicompact spaces, Sov. Math. Dokl. 4 (1963), 1051–1055.Google Scholar
2. Bennett, H. R. and Lutzer, D. J., A note on weak 0-refinability, General Topology and Appl. 2 (1972), 49–54.Google Scholar
3. Borges, C. J. R., On stratifiable spaces, Pacific J. Math. 17 (1966), 1–16.Google Scholar
4. Burke, D. K., On p-spaces and wA-spaces, Pacific J. Math. 35 (1970), 285–296.Google Scholar
5. Burke, D. K., A nondevelopable locally compact Hausdorff space with a G'diagonal, General Topology and Appl. 2 (1972), 287–292.Google Scholar
6. Céder, J. G., Some generalizations of metric spaces, Pacific J. Math. 11 (1961), 105–126.Google Scholar
7. Creede, G. D. D., Concerning semi-stratifiable spaces, Pacific J. Math. 32 (1970), 47–54.Google Scholar
8. Dugundji, J., Topology (Allyn & Bacon, Boston, Mass., 1966).Google Scholar
9. Heath, R. W., Arcwise connectedness in semi-metric spaces, Pacific J. Math. 12 (1962), 1301–1319.Google Scholar
10. Kelly, J. L., General topology (Van Nostrand Princeton, New Jersey, 1955).Google Scholar
11. Martin, H. W., Metrization and submetrization of topological spaces, Ph.D. Thesis, University of Pittsburgh, 1973.Google Scholar
12. Michael, E., On Nagamïs espaces and some related matters, Proc. Wash. S. Univ. Conf., 1970, 13–18.Google Scholar
13. Morita, K. and Hanai, S., Closed mappings and metric spaces, Proc. Jap. Acad. 32 (1956), 10–14.Google Scholar
14. Siwiec, F., On the theorem of Morita, Hanai and Stone (to appear).Google Scholar
15. Stone, A. H., Metrizability of decomposition spaces, Proc. Amer. Math. Soc. 7 (1956), 690–700.Google Scholar
16. Worrell, J. M. and Wicke, H. H., Characterizations of developable topological spaces, Can. J. Math. 17 (1965), 820–830.Google Scholar