Multi-linear forms, graphs, and 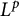$L^p$-improving measures in ${\Bbb F}_q^d$

Abstract

The purpose of this paper is to introduce and study the following graph-theoretic paradigm. Let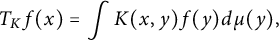

$$ \begin{align*}T_Kf(x)=\int K(x,y) f(y) d\mu(y),\end{align*} $$

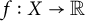where $f: X \to {\Bbb R}$, X a set, finite or infinite, and K and 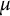$\mu $ denote a suitable kernel and a measure, respectively. Given a connected ordered graph G on n vertices, consider the multi-linear form

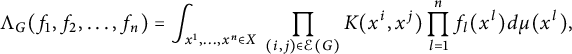$$ \begin{align*}\Lambda_G(f_1,f_2, \dots, f_n)=\int_{x^1, \dots, x^n \in X} \ \prod_{(i,j) \in {\mathcal E}(G)} K(x^i,x^j) \prod_{l=1}^n f_l(x^l) d\mu(x^l),\end{align*} $$

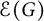where ${\mathcal E}(G)$ is the edge set of G. Define $\Lambda _G(p_1, \ldots , p_n)$ as the smallest constant 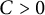$C>0$ such that the inequality (0.1)

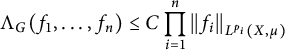$$ \begin{align} \Lambda_G(f_1, \dots, f_n) \leq C \prod_{i=1}^n {||f_i||}_{L^{p_i}(X, \mu)} \end{align} $$

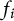holds for all nonnegative real-valued functions $f_i$, 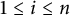$1\le i\le n$, on X. The basic question is, how does the structure of G and the mapping properties of the operator 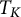$T_K$ influence the sharp exponents in (0.1). In this paper, this question is investigated mainly in the case 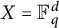$X={\Bbb F}_q^d$, the d-dimensional vector space over the field with q elements, 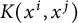$K(x^i,x^j)$ is the indicator function of the sphere evaluated at 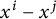$x^i-x^j$, and connected graphs G with at most four vertices.