No CrossRef data available.
Article contents
Monodromy Action on Unknotting Tunnelsin Fiber Surfaces
Published online by Cambridge University Press: 20 November 2018
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
In a 2012 paper, the second author showed that a tunnel of a tunnel number one, fibered link in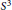 
${{S}^{3}}$ can be isotoped to lie as a properly embedded arc in the fiber surface of the link. In this paper we observe that this is true for fibered links in any 3-manifold, we analyze how the arc behaves under the monodromy action, and we show that the tunnel arc is nearly clean, with the possible exception of twisting around the boundary of the fiber.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2016
References
[1]
Baker, K. L., Johnson, J. E., and Klodginski, E. A., Tunnel number one, genus-onefibered knots.
Comm. Anal. Geom.
17(2009), no. 1, 1–16. http://dx.doi.org/10.4310/CAC.2009.v17.n1.a1
Google Scholar
[2]
Birman, J. S., A representation theorem forfibered knots and their monodromy maps.
In: Topology
of low-dimensional manifolds (Proc. Second Sussex Conf., Chelwood Gate, 1977), Lecture Notes
in Math., 722, Springer, Berlin, 1979, pp. 1–8.Google Scholar
[3]
Brittenham, M. and Wu, Y.-Q., The classification of exceptionalDehn surgeries on 2-bridge knots.
Comm. Anal. Geom. 9(2001), no. 1, 97–113. http://dx.doi.Org/10.4310/CAC.2001.v9.n1.a4
Google Scholar
[4]
Casson, A. J. and Bleiler, S. A., Automorphisms ofsurfaces after Nielsen and Thurston.
London
Mathematical Society Student Texts, 9, Cambridge University Press, Cambridge, 1988.
http://dx.doi.Org/10.1017/CBO9780511623912
Google Scholar
[5]
Cho, S. and McCullough, D., Cabling sequences oftunnels oftorus knots.
Algebr. Geom. Topol.
9(2009), no. 1, 1–20. http://dx.doi.Org/10.2140/agt.2009.9.1
Google Scholar
[6]
Cho, S., The tree ofknot tunnels.
Geom. Topol.
13(2009), no. 2, 769–815. http://dx.doi.org/10.2140/gt.2009.13.769
Google Scholar
[7]
Cho, S., Constructing knot tunnels usinggiant Steps.
Proc. Amer. Math. Soc.
138(2010), no. 1, 375–384. http://dx.doi.org/10.1090/S0002-9939-09-10069-2
Google Scholar
[8]
Coward, A. and Lackenby, M., Unknotting genus one knots.
Comment. Math. Helv.
86(2011), no. 2, 383–399. http://dx.doi.org/10.4171/CMH/227
Google Scholar
[9]
Futer, D., Kalfagianni, E., and Purcell, J. S., Cusp areas ofFarey manifolds and applications to knot theory.
Int. Math. Res. Not. IMRN 2010, no. 23, 4434–4497. http://dx.doi.Org/10.1093/imrn/rnqO37
Google Scholar
[10]
Futer, D. and Schleimer, S. , Cusp geometry of fibered 3-manifolds.
Amer. J. Math.
136(2014), no. 2, 309–356. http://dx.doi.Org/10.1353/ajm.2O14.0012
Google Scholar
[11]
Gabai, D., The Murasugi sum is a natural geometric Operation. II.
In: Combinatorial methods in topology and algebraic geometry (Rochester, N.Y., 1982), Contemp. Math., 44, American Mathematical Society, Providence, RI, 1985, pp.93–100. http://dx.doi.Org/10.1090/conm/044/813105
Google Scholar
[12]
Gabai, D., Detectingfibred links in S3.
Comment. Math. Helv.
61(1986), no. 4, 519–555. http://dx.doi.org/10.1007/BF02621931
Google Scholar
[13]
Gabai, D., Problems in foliations and laminations. In: Geometric topology (Athens, GA, 1993), AMS/IP Stud. Adv. Math., 2.2, American Mathematical Society, Providence, RI, 1997, pp. 1–33.Google Scholar
[14]
Gadre, V. and Tsai, C.-Y., Minimal pseudo-Anosov translation lengths on the complex of curves.
Geom. Topol.
15(2011), no. 3, 1297–1312. http://dx.doi.org/10.2140/gt.2011.15.1297
Google Scholar
[15]
Giroux, E. and Goodman, N., On the stable equivalence ofopen books in three-manifolds.
Geom.Topol.
10(2006), 97–114. http://dx.doi.org/10.2140/gt.2006.10.97
Google Scholar
[16]
Goda, H., Heegaard Splitting for sutured manifolds and Murasugi sum.
Osaka J. Math.
29(1992), no. 1, 21–40.Google Scholar
[17]
Gordon, C. McA. and Reid, A. W., Tangle decompositions oftunnel number one knots and links.
J. Knot Theory Ramifications
4(1995), no. 3, 389–409.http://dx.doi.org/10.1142/S0218216595000193
Google Scholar
[18]
Harer, J., How to construct all fibered knots and links.
Topology
21(1982), no. 3, 263–280. http://dx.doi.Org/10.1016/0040-9383(82)90009-X
Google Scholar
[19]
Hilden, H. M., Tejada, D. M., and Toro, M. M., Tunnel number one knots have palindrome
presentations.
J. Knot Theory Ramifications
11(2002), no. 5, 815–831. http://dx.doi.org/10.1142/S0218216502001998
Google Scholar
[20]
Johnson, J., Surface bundles with genus two Heegaard Splittings.
J. Topol.
1(2008), 671–692. http://dx.doi.Org/10.1112/jtopol/jtn018
Google Scholar
[21]
Kazez, W. H. and Roberts, R., Fractional Dehn twists in knot theory and contact topology.
Algebr.
Geom. Topol.
13(2013), no. 6, 3603–3637. http://dx.doi.org/10.2140/agt.2013.13.3603
Google Scholar
[22]
Kobayashi, T., Fibered links and unknotting operations.
Osaka J. Math.
26(1989), no. 4, 699–742.Google Scholar
[23]
Kobayashi, T., Classification of unknotting tunnels for two bridge knots.
In: Proceedings of the Kirbyfest (Berkeley, CA, 1998), Geom. Topol. Monogr., 2, Geom. Topol. Publ., Coventry, 1999, pp. 259–290.http://dx.doi.Org/10.2140/gtm.1999.2.259
Google Scholar
[24]
Morimoto, K., Sakuma, M., and Yokota, Y., Identifying tunnel number one knots.
J. Math. Soc.
Japan
48(1996), no. 4, 667–688. http://dx.doi.Org/1O.2969/jmsj7O484O667
Google Scholar
[25]
Nakamura, T., On canonical genus offibered knot.
J. Knot Theory Ramifications
11(2002), no. 3, 341–352. http://dx.doi.org/10.1142/S021821650200165
Google Scholar
[26]
Ni, Y., Knot Floer homology detectsfibred knots.
Invent. Math.
170(2007), no. 3, 577–608. http://dx.doi.org/10.1007/s00222-007-0075-9
Google Scholar
[27]
Otal, J.-P., Le théorème d'hyperbolisation pour les Variétés fibrées de dimension 3.
Astérisque, 235, 1996.Google Scholar
[28]
Otal, J.-P., The hyperboüzation theorem forfibered 3-manifolds.SMF/AMS Texts and Monographs, 7, American Mathematical Society, Providence, RI, 2001.Google Scholar
[29]
Rathbun, M., Tunnel one, fibered links.
Pacific J. Math.
259(2012), no. 2, 473–481.
http://dx.doi.org/10.2140/pjm.2012.259.473
Google Scholar
[30]
Sakuma, M., Murasugi decompositions ofcertain Seifert surfaces and unknotting operations.
In:
Proceedings of the 5th Korea-Japan School of knots and links, 1997, pp. 233–248.Google Scholar
[31]
Sakuma, M., Unknotting tunnels and canonical decompositions ofpunctured torus bundles over a circle. Analysis of discrete groups (Kyoto, 1995).
Sürikaisekikenkyüsho Kökyüroku, 967(1996), 58–70.Google Scholar
[32]
Sakuma, M., The topology, geometry and algebra of unknotting tunnels.
Chaos, Solitons and Fractals
9(1998), no. 4-5, 739–748. http://dx.doi.Org/10.1016/S0960-0779(97)00101-
Google Scholar
[33]
Scharlemann, M., Tunnel number one knots satisfy the Poenaru conjecture.
Topology Appl.
18(1984), no. 2-3, 235–258. http://dx.doi.Org/10.1016/0166-8641(84)90013-0
Google Scholar
[34]
Scharlemann, M. and Thompson, A., Unknotting tunnels and Seifert surfaces.
Proc. London Math.
Soc. (3) 87(2003), no. 2, 523–544. http://dx.doi.Org/10.1112/S0024611503014242
Google Scholar
[35]
Stallings, J. R., Constructions offibred knots and links.
In: Algebraic and geometric topology (Proc. Sympos. Pure Math., Stanford Univ., Stanford, Calif, 1976), Part 2, Proc. Sympos. Pure Math., 32, American Mathematical Society, Providence, RI, 1978, pp. 55–60.Google Scholar
[36]
Sullivan, D., Travaux de Thurston sur les groupes quasi-fuchsiens et les Varéités hyperboliques de dimension 3 fibrees surS1. In: Bourbaki Seminar, Vol.
1979/80, Lecture Notes in Math., 842, Springer, Berlin-New York, 1981, pp. 196–214.Google Scholar
[37]
Thurston, W. P., On the geometry and dynamics of diffeomorphisms of surfaces.
Bull. Amer. Math. Soc. (N.S.) 19(1988), no. 2, 417–431. http://dx.doi.org/10.1090/S0273-0979-1988-15685-6
Google Scholar
[38]
Yamamoto, R., Stallings twists which can be realized byplumbing and deplumbing Hopf bands.
J. Knot Theory Ramifications
12(2003), no. 6, 867–876. http://dx.doi.oriV10.1142/S0218216503002779
Google Scholar
You have
Access