Hostname: page-component-745bb68f8f-hvd4g Total loading time: 0 Render date: 2025-01-12T01:44:24.791Z Has data issue: false hasContentIssue false
  • English
  • Français

The MOD 2 K-Homology of Ω3S3X

Published online by Cambridge University Press:  20 November 2018

J. G. Mayorquin
J. G. Mayorquin*
Affiliation:
Memorial University, St. John's, Newfoundland
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In order to compute the group K*(Ω3S3X; Z/2) when X is a finite, torsion free CW-complex we apply the techniques developed by Snaith in [38], [39], [40], [41] which were used in [42] to determine the Atiyah-Hirzebruch spectral sequence ( [11], [1, Part III])

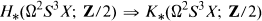
for X as above. Roughly speaking the method consists in defining certain classes in K*(Ω3S3X; Z/2) via the π-equivariant mod 2 K-homology of S2 × Y2,

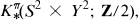
([35]), π the cyclic group of order 2 (acting antipodally on S2, by permuting factors in Y2, and diagonally on S2 × Y2), Y a finite subcomplex of Ω3S3X, and then showing that the classes so produced map under the edge homomorphism to cycles (in the E1-term of the Atiyah-Hirzebruch spectral sequence for

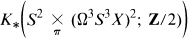
which determine certain homology classes of H*(Ω3S3X; Z/2), thus exhibiting these as infinite cycles of the spectral sequence

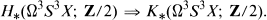
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 40 , Issue 1 , 01 February 1988 , pp. 142 - 196
Copyright
Copyright © Canadian Mathematical Society 1988

References

1. Adams, J. F., Lectures on generalized homology, Mathematics Lecture Notes, University of Chicago (1970).Google Scholar
2. Adem, J., The relations on Steenrodpowers of cohomology classes, Algebraic Geometry and Topology (Princeton University Press, 1957), 191–238.CrossRefGoogle Scholar
3. Anderson, D. W., Universal coefficient theorems for K-theory (preprint).Google Scholar
4. Anderson, D. W. and Hodgkin, L., The K-theory of Eilenberg-Mac Lane complexes, Topology. 7 (1968), 317–29.Google Scholar
5. Araki, S., Hodgkin's theorem, Ann. Math. 85 (1957), 508–525.Google Scholar
6. Araki, S. and Kudo, T., Topology of Hn-spaces and H-squaring operations, Mem. Fac. Kyusyu Univ., Ser. A, 10 (1956), 85–120.Google Scholar
7. Araki, S. and Toda, H., Multiplicative structures in mod q cohomology theories I, II, Osaka J. Math.. 2 (1965), 71–115 and 3 (1966), 81–120.Google Scholar
8. Araki, S. and Yosimura, Z., Differential Hopf algebras modelled on K-theory mod p I, Osaka J. Math.. 8 (1971), 151–206.Google Scholar
9. Atiyah, M. F., Characters and cohomology of finite groups, Pub. Math.. 9 (IHES, Paris).Google Scholar
10. Atiyah, M. F., K-theory (Benjamin Press, 1968).Google Scholar
11. Atiyah, M. F. and Hirzebruch, F., Vector bundles and homogeneous spaces, Differential Geometry, Proc. of Symp. in Pure Math.. 3 (Amer. Math. Soc, 1961).Google Scholar
12. Atiyah, M. F. and Segal, G. B., Equivariant K-theory and completion, J. Diff. Geom.. 3 (1969), 1–18.Google Scholar
13. Boardman, J. M. and Vogt, R. M., Homotopy-everything H-spaces, Bull. Amer. Math. Soc.. 74 (1968), 1117–1122.Google Scholar
14. Browder, W., Homology operations and loop spaces, Illinois J. Math.. 4 (1960), 347–357.Google Scholar
15. Brown, E. H. and Peterson, F. P., On the stable decomposition of Ω2Sr+2 , Trans. Am. Math. Soc.. 243(1978), 287–298.Google Scholar
16. Cartan, H. and Eilenberg, S., Homological algebra (Princeton University Press, 1956).Google Scholar
17. Caruso, J., Cohen, F. R., May, J. P. and Taylor, L. R., James maps, Segal maps and the Kahn-Priddy theorem, Trans. AMS. 1, 281.Google Scholar
18. Cohen, J. M., Stable homotopy, Lecture Notes in Math. 165 (Springer-Verlag).Google Scholar
19. Cohen, F. R., Lada, T. J. and May, J. P., The homology of iterated loop spaces, Lecture Notes in Math.. 533 (1976).CrossRefGoogle Scholar
20. Cohen, F. R., Mahowald, M. and Milgram, R. J., The stable decomposition of the double loop space of a sphere, AMS Proc. Symp. Pure. Math.. 32 (1978), part 2 (1978), 225–228.Google Scholar
21. Cohen, F. R., May, J. P. and Taylor, L. R., Splitting of certain spaces CX, Math. Proc. Camb. Phil. Soc. 84 (1978), 465–496.Google Scholar
22. Dyer, E. and Lashof, R. K., Homology of iterated loop spaces. Amer. J. Math. 84 (1962), 35–88.Google Scholar
23. Eilenberg, S. and Moore, J. C., Homology and fibrations I, Com. Mat. Helv.. 40 (1966), 199–236.Google Scholar
24. Hodgkin, L., A Kunneth formula in equivariant K-theory, Warwick Univ. preprint (1968).Google Scholar
25. Hodgkin, L., The K-theory of Lie groups, Topology. 6 (1967), 1–36.Google Scholar
26. Hodgkin, L., Dyer-Lashof operations in K-theory, Proc. Oxford Symposium in Algebraic Topology (1972), London Mathematical Society Lecture Note Series 11.Google Scholar
27. May, J. P., Categories of spectra and infinite loop spaces, Lecture Notes in Math. 99 (Springer-Verlag).Google Scholar
28. May, J. P., A general algebraic approach to Steenrod operations, Lecture Notes in Math. 168 (Springer-Verlag).Google Scholar
29. May, J. P., Homology operations on infinite loop spaces, Proc. Symp. Pure Math.. 22 (Amer. Math. Soc. 1971).Google Scholar
30. May, J. P., The geometry of iterated loop spaces, Lecture Notes in Math. 271 (Springer-Verlag).Google Scholar
31. May, J. P., E∞ ring spaces and E∞ ring spectra, Lecture Notes in Math. 577 (Springer-Verlag).Google Scholar
32. Massey, W. S., Exact couples in algebraic topology I, II, Ann. Math.. 56 and 57.Google Scholar
33. Milnor, J. W., On axiomatic homology theory, Pacific J. Math.. 12 (1962), 337–341.Google Scholar
34. Rothenberg, M. and Steenrod, N. E., The cohomology of classifying spaces of H-spaces, Bull. Amer. Math. Soc.. 71 (1961).Google Scholar
35. Segal, G. B., Equivariant K-theory, Pub. Math.. 34 (IHES Paris, 1968).Google Scholar
36. Segal, G. B., Classifying spaces and spectral sequences, Publ. Math. Inst, des Hautes Etudes Scient. (Paris) 34 (1968).Google Scholar
37. Snaith, V. P., A stable decomposition of EnSnX, J. London Math. Soc.. 7 (1974), 577–583.Google Scholar
38. Snaith, V. P., On the K-theory of homogeneous spaces and conjugate bundles of Lie groups, Proc. L. M. Soc. (3) 22 (1971), 562–584.Google Scholar
39. Snaith, V. P., On cyclic maps, Proc. Camb. Phil. Soc. (1972), 449–456.Google Scholar
40. Snaith, V. P., Massey products in K-theory I, II, Proc. Camb. Phil. Soc.. 68 (1970), 303–320 and 69 (1971), 259–289.Google Scholar
41. Snaith, V. P., Dyer-Lashof operations in K-theory, Lecture Notes in Math.. 496 (1975).Google Scholar
42. Snaith, V. P., On K*(Ω2X; Z/2), Quart. J. Math. Oxford (3), 26 (1975), 421–436.Google Scholar
43. Miller, Haines and Snaith, V. P., On K*(Ω2X; Z/2), Canadian Math. Soc. Conference Proc.. 2, Part 1 (1982).Google Scholar
44. Steenrod, N. E., The cohomology algebra of a space, L'enseignement Math. II Série Tome. 7 (1961), 153–178.Google Scholar
45. Steenrod, N. E. and Epstein, D. B. A., Cohomology operations, Annals of Math. Study 50 (Princeton Press, 1962).Google Scholar
46. Whitehead, G. W., Generalized homology theory, Trans. Am. Math Soc.. 102 (1962), 227–283.Google Scholar