Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-26T18:34:49.396Z Has data issue: false hasContentIssue false
  • English
  • Français

Majorization-Subordination Theorems for Locally Univalent Functions, II

Published online by Cambridge University Press:  20 November 2018

Douglas Michael Campbell
Douglas Michael Campbell*
Affiliation:
Brigham Young University, Provo, Utah
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

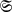Let denote the set of all normalized analytic univalent functions in the open unit disc D. Let f(z), F(z) and φ(z) be analytic in |z| < r. We say that f(z) is majorized by F(z) in 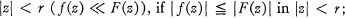we say that f(z) is subordinate to F(z) in 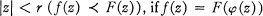where .

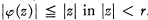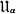Let be the set of all locally univalent (f’(z) ≠ 0) analytic functions in D with order ≦α which are of the form f(z) = z +… . The family 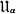is known as the universal linear invariant family of order α [6]. A concise summary of and introduction to properties of linear invariant families which relate to the following material is contained in [1]. The present paper contains the proofs of some of the results announced in [1]

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 25 , Issue 2 , April 1973 , pp. 420 - 425
Copyright
Copyright © Canadian Mathematical Society 1973

References

1. Campbell, D. M., Majorization-subordination theorems for locally univalent functions, Bull. Amer. Math. Soc. 78 (1972), 535–538.Google Scholar
2. Lewandowski, Z., Sur les majorants des fonctions holomorphes dans le cercle \z\ < 1, Ann. Univ. Mariae Curie-Sklodowska Sect A 15 (1961), 5–11.Google Scholar
3. Littlewood, J. E., Lectures on the theory of functions (Oxford University Press, Oxford, 1944).Google Scholar
4. MacGregor, T. H., Majorization by univalent functions, Duke Math. J. 34 (1967), 95–102.Google Scholar
5. Nehari, Z., Conformai mapping (McGraw-Hill, New York, 1952).Google Scholar
6. Pommerenke, Ch., Linear-invariante Familien Analytischer Funktionen. J, Math. Ann. 155 (1964), 108–154.Google Scholar