No CrossRef data available.
Article contents
Localizations of Linked Quaternionic Mappings
Published online by Cambridge University Press: 20 November 2018
Extract
Let G and B be abelian groups with G having exponent 2 and a distinguished element –1. In [7] we defined a linked quaternionic mapping to be a map q : G × G → B satisfying the following properties:
(A) q is symmetric and bilinear
(B) q(a, a) = q(a, – 1) for every a ∈ G, and
(L) q(a, b) = q(c, d) implies there exists x ∈ G such that q(a, b) = q(a, x) and q(c, d) = q(c, x).
A form (of dimension n over q) is a symbol φ = 〈a1, …, an〉 with a1, …, an ∈ G. The determinant and Hasse invariant of such a form φ are
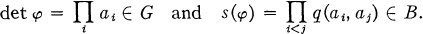
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1982


