Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-28T03:14:34.545Z Has data issue: false hasContentIssue false
  • English
  • Français

Integral Points on Elliptic Curves and Explicit Valuations of Division Polynomials

Published online by Cambridge University Press:  20 November 2018

Katherine E. Stange
Katherine E. Stange*
Affiliation:
Department of Mathematics, University of Colorado Boulder, Campus Box 395, Boulder, CO, 80309, USA e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Assuming Lang's conjectured lower bound on the heights of non-torsion points on an elliptic curve, we show that there exists an absolute constant 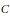$C$ such that for any elliptic curve 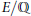$E/\mathbb{Q}$ and non-torsion point 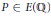$P\,\in \,E\left( \mathbb{Q} \right)$, there is at most one integral multiple 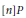$\left[ n \right]P$ such that 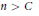$n\,>\,C$. The proof is a modification of a proof of Ingram giving an unconditional, but not uniform, bound. The new ingredient is a collection of explicit formulæ for the sequence 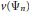$v\left( {{\Psi }_{n}} \right)$ of valuations of the division polynomials. For 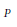$P$ of non-singular reduction, such sequences are already well described in most cases, but for 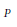$P$ of singular reduction, we are led to define a new class of sequences called elliptic troublemaker sequences, which measure the failure of the Néron local height to be quadratic. As a corollary in the spirit of a conjecture of Lang and Hall, we obtain a uniform upper bound on $\widehat{h}\left( P \right)/h\left( E \right)$ for integer points having two large integral multiples.

Keywords

11G0511G0711D2511B3711B3911Y5511G5011H52elliptic divisibility sequenceLang's conjectureheight functions
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 68 , Issue 5 , 01 October 2016 , pp. 1120 - 1158
Copyright
Copyright © Canadian Mathematical Society 2016

References

[1] Ayad, M., Points S-entiers des courbes elliptiques. Manuscripta Math. 76( 1992), no. 3-4, 305–324.http://dx.doi.org/10.1007/BF02567763 Google Scholar
[2] Baker, A., The Diophantine equation y2 = ax3 + bx2 + cx+ d. J. London Math. Soc. 43(1968), 1–9.http://dx.doi.Org/10.1112/jlms/s1-43.1.1 Google Scholar
[3] Cheon, J. and Hahn, S., Explicit valuations of division polynomials of an elliptic curve. Manuscripta Math. 97(1998), no. 3, 319–328.http://dx.doi.org/10.1007/s002290050104 Google Scholar
[4] Cheon, J., The orders of the reductions of a point in the Mordell-Weil group of an elliptic curve. Acta Arith. 88(1999), no. 3, 219–222.Google Scholar
[5] Cornelissen, G. and Zahidi, K., Elliptic divisibility sequences and undecidableproblems about rational points. J. Reine Angew. Math. 613(2007), 1–33,http://dx.doi.Org/10.1515/CRELLE.2007.089 Google Scholar
[6] David, S., Minorations déformes linéaires de logarithmes elliptiques. Mém. Soc. Math. France (N.S.) 62(1995).Google Scholar
[7] Einsiedler, M., Everest, G., Ward, T. , Primes in elliptic divisibility sequences. LMS J. Comput. Math. 4(2001), 1–13.http://dx.doi.Org/10.1112/S1461157000000772 Google Scholar
[8] Ekedahl, T. , One semester of elliptic curves. EMS Series of Lectures in Mathematics, European Mathematical Society (EMS), Zürich, 2006.http://dx.doi.Org/10.4171/015 Google Scholar
[9] Everest, G. and Ward, T., The canonical height of an algebraic point on an elliptic curve. New York J. Math. 6(2000), 331–342.Google Scholar
[10] Everest, G., Primes in divisibility sequences. Cubo Mat. Educ. 3(2001), no. 2, 245–259.Google Scholar
[11] Everest, G. and King, H., Prime powers in elliptic divisibility sequences. Math. Comp. 74(2005), 2061–2071. http://dx.doi.org/10.1090/S0025-5718-05-01737-0 Google Scholar
[12] Everest, G., Mclaren, G., and Ward, T., Primitive divisors of elliptic divisibility sequences. J. Number Theory 118(2006), no. 1,71–89.http://dx.doi.Org/10.1016/j.jnt.2005.08.002 Google Scholar
[13] Gezer, B. and Bizim, O., Elliptic divisibility sequences associated to elliptic curves with torsion points. 2011, arxiv:1101.3839 Google Scholar
[14] Everest, G., Squares in elliptic divisibility sequences. Acta Arith. 144(2010), no. 2, 125–134. http://dx.doi.Org/10.4064/aa144-2-2 http://dx.doi.Org/10.4064/aa1 44-2-2 Google Scholar
[15] Hall, M. Jr., The Diophantine equation x3 — y2 = k. In: Computers in number theory (Proc. Sci. Res. Council Atlas Sympos. No. 2, Oxford, 1969), Academic Press, London, 1971, pp. 173–198.Google Scholar
[16] Hindry, M. and Silverman, J. H., The canonical height and integral points on elliptic curves. Invent. Math. 93(1988), no. 2, 419–450.http://dx.doi.org/10.1007/BF01394340 Google Scholar
[17] Ingram, P., Multiples of integral points on elliptic curves. J. Number Theory 129(2009), no. 1, YEAR = 2009, 182–208.http://dx.doi.Org/10.101 6/j.jnt.2008.08.001 http://dx.doi.Org/10.101 6/j.jnt.2008.08.001 Google Scholar
[18] Ingram, P. and Silverman, J. H., Uniform estimates for primitive divisors in elliptic divisibility sequences. In: Number theory, Analysis and Geometry (In memory of Serge Lang), Springer-Verlag, Berlin, pp. 233–263.Google Scholar
[19] Lang, S., Elliptic curves: Diophantine analysis. Grundlehren der Mathematischen Wissenschaften, 231, Springer-Verlag, Berlin, 1978.Google Scholar
[20] Lang, S., Conjectured Diophantine estimates on elliptic curves. In: Arithmetic and geometry, I, Progr. Math., 35, Birkhâuser Boston, Boston, MA, 1983, pp. 155–171.Google Scholar
[21] Mahé, V., Primepower terms in elliptic divisibility sequences. Math. Comp. 83(2014), no. 288, 1951–1991.http://dx.doi.org/10.1090/S0025-5718-2013-02790-1 http://dx.doi.org/10.1090/S0025-5718-2013-02790-1 Google Scholar
[22] Pinter, A., On the magnitude of integer points on elliptic curves. Bull. Austral. Math. Soc. 52(1995), no. 2, pp. 195–199. http://dx.doi.org/10.1017/S000497270001460X http://dx.doi.Org/10.1017/S0004972 70001460X Google Scholar
[23] Schmidt, W. M., Integer points on curves of genus. Compositio Math. 81(1992), no. 1, 33–59.Google Scholar
[24] Shipsey, R., Elliptic divisibility sequences.Ph.D. thesis, Goldsmith's College (University of London), 2000.Google Scholar
[25] Silverman, J. H., Lower bound for the canonical height on elliptic curves. Duke Math. J. 48(1981), no. 3, 633–648. http://dx.doi.org/10.1215/S0012-7094-81-04834-1 Google Scholar
[26] Silverman, J. H., A quantitative version ofSiegel's theorem: integral points on elliptic curves and Catalan curves. J. Reine Angew. Math. 378( 1987), 60–100.Google Scholar
[27] Silverman, J. H., The difference between the Weil height and the canonical height on elliptic curves. Math. Comp. 55(1990), no. 192, 723–743.http://dx.doi.org/10.1090/S0025-5718-1990-1035944-5 Google Scholar
[28] Silverman, J. H., Advanced topics in the arithmetic of elliptic curves. Graduate Texts in Mathematics, 151, Springer-Verlag, New York, 1994.Google Scholar
[29] Silverman, J. H., The arithmetic of elliptic curves. Graduate Texts in Mathematics, 106, Springer, Dordrecht, 2009.Google Scholar
[30] Silverman, J. H. and Stange, K. E., Terms in elliptic divisibility sequences divisible by their indices. Acta Arith. 146(2011), no. 4, 355–378.http://dx.doi.org/10.4064/aa146-4-4 http://dx.doi.Org/10.4064/aa146-4-4 Google Scholar
[31] Silverman, J. H. and Stephens, N., The sign of an elliptic divisibility sequence. J. Ramanujan Math. Soc. 21(2006), no. 1, 1–17.Google Scholar
[32] Sprindzuk, V. G., Classical Diophantine equations. Lecture Notes in Mathematics, 1559, Springer-Verlag, Berlin, 1993.Google Scholar
[33] Stange, K., Elliptic nets and elliptic curves. Algebra Number Theory 5 (2011), no. 2, 197–229. http://dx.doi.org/10.2140/ant.2011.5.197 http://dx.doi.org/10.2140/ant.2011.5.197 Google Scholar
[34] Stark, H. M., Effective estimates of solutions of some Diophantine equations. Acta Arith. 24( 1973), 251–259.Google Scholar
[35] Stein, W. A., et. al., The Sage Development Team, Sage Mathematics Software (Version 4.6.2). 2011.http://www.sagemath.org Google Scholar
[36] Streng, M.,Divisibility sequences for elliptic curves with complex multiplication. Algebra Number Theory 2(2008), 183–208. http://dx.doi.Org/10.2140/ant.2008.2.183. http://dx.doi.Org/10.2140/ant.2008.2.183 Google Scholar
[37] Ward, M., Memoir on elliptic divisibility sequences. Amer. J. Math. 70(1948), 31–74.http://dx.doi.Org/10.2307/2371930 Google Scholar
[38] Yabuta, M., Primitive divisors of certain elliptic divisibility sequences. Experiment. Math. 18(2009), no. 3, 303–310.http://dx.doi.ore/10.1080/10586458.2009.10129047 Google Scholar