Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ishiguro, Kenshi
1996.
Classifying spaces and homotopy sets of axes of pairings.
Proceedings of the American Mathematical Society,
Vol. 124,
Issue. 12,
p.
3897.
Lee, Kee
and
Woo, Moo
1996.
Generalized evaluation subgroups of product spaces relative to a factor.
Proceedings of the American Mathematical Society,
Vol. 124,
Issue. 7,
p.
2255.
Lee, Kee Young
and
Woo, Moo Ha
2001.
Cocyclic morphisms and dual G-sequences.
Topology and its Applications,
Vol. 116,
Issue. 1,
p.
123.
Yoon, Yeon Soo
2001.
The generalized dual Gottlieb sets.
Topology and its Applications,
Vol. 109,
Issue. 2,
p.
173.
Hardie, K.A.
Vermeulen, J.J.C.
and
Witbooi, P.J.
2002.
A nontrivial pairing of finite T0 spaces.
Topology and its Applications,
Vol. 125,
Issue. 3,
p.
533.
Hardie, K.A.
Salbany, S.
Vermeulen, J.J.C.
and
Witbooi, P.J.
2003.
A non-Hausdorff quaternion multiplication.
Theoretical Computer Science,
Vol. 305,
Issue. 1-3,
p.
135.
Kim, Ji-Yean
and
Lee, Kee-Young
2010.
GOTTLIEB SUBSETS WITH RESPECT TO A MORPHISM IN THE CATEGORY OF PAIRS.
Bulletin of the Korean Mathematical Society,
Vol. 47,
Issue. 6,
p.
1311.
Iwase, Norio
Mimura, Mamoru
Oda, Nobuyuki
and
Yoon, Yeon Soo
2012.
The Milnor–Stasheff Filtration on Spaces and Generalized Cyclic Maps.
Canadian Mathematical Bulletin,
Vol. 55,
Issue. 3,
p.
523.
Kim, Jae-Ryong
and
Oda, Nobuyuki
2013.
The set of cyclic-element preserving maps.
Topology and its Applications,
Vol. 160,
Issue. 6,
p.
794.
Kim, Jae-Ryong
and
Oda, Nobuyuki
2015.
Cocyclic element preserving pair maps and fibrations.
Topology and its Applications,
Vol. 191,
Issue. ,
p.
82.
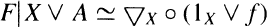 for the folding map ∇X: X ∨ X → X. He defined the generalized Gottlieb set G(A, X) of the homotopy classes of the cyclic maps F: A → X and studied the fundamental properties of G(A, X) If A is a co-Hopf space, then the Varadarajan set G(A, X) has a group structure [13]. The group G(A,X) is a generalization of G(X) and Gn(X) of Gottlieb [2,3]. Some authors studied the properties of the Varadarajan set, its dual and related topics [4, 5, 6, 7,12,15,16,17].
for the folding map ∇X: X ∨ X → X. He defined the generalized Gottlieb set G(A, X) of the homotopy classes of the cyclic maps F: A → X and studied the fundamental properties of G(A, X) If A is a co-Hopf space, then the Varadarajan set G(A, X) has a group structure [13]. The group G(A,X) is a generalization of G(X) and Gn(X) of Gottlieb [2,3]. Some authors studied the properties of the Varadarajan set, its dual and related topics [4, 5, 6, 7,12,15,16,17].

