The Homology of Abelian Covers of Knotted Graphs

Abstract

Let 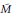$\tilde{M}$ be a regular branched cover of a homology 3-sphere 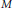$M$ with deck group 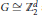$G\cong \mathbb{Z}_{2}^{d}$ and branch set a trivalent graph $\Gamma $; such a cover is determined by a coloring of the edges of $\Gamma $ with elements of $G$. For each index-2 subgroup 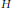$H$ of 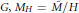$G,\,{{M}_{H}}=\tilde{M}/H$ is a double branched cover of 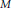$M$. Sakuma has proved that 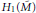${{H}_{1}}\left( {\tilde{M}} \right)$ is isomorphic, modulo 2-torsion, to 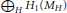${{\oplus }_{H}}{{H}_{1}}\left( {{M}_{H}} \right)$, and has shown that 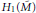${{H}_{1}}\left( {\tilde{M}} \right)$ is determined up to isomorphism by 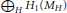${{\oplus }_{H}}{{H}_{1}}\left( {{M}_{H}} \right)$ in certain cases; specifically, when $d=2$ and the coloring is such that the branch set of each cover 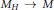${{M}_{H}}\to M$ is connected, and when $d=3$ and $\Gamma $ is the complete graph 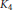${{K}_{4}}$. We prove this for a larger class of coverings: when $d=2$, for any coloring of a connected graph; when 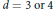$d=3\,\text{or}\,\text{4}$, for an infinite class of colored graphs; and when 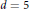$d=5$, for a single coloring of the Petersen graph.