Hostname: page-component-586b7cd67f-rcrh6 Total loading time: 0 Render date: 2024-11-28T00:57:13.706Z Has data issue: false hasContentIssue false
  • English
  • Français

A Hilbert Scheme in Computer Vision

Published online by Cambridge University Press:  20 November 2018

Chris Aholt ,
Bernd Sturmfels  and
Rekha Thomas
Chris Aholt
Affiliation:
Mathematics, University of Washington, Seattle, WA 98195, USA, e-mail: [email protected], [email protected]
Bernd Sturmfels
Affiliation:
Mathematics, University of California, Berkeley, CA 94720, USA, e-mail: [email protected]
Rekha Thomas
Affiliation:
Mathematics, University of Washington, Seattle, WA 98195, USA, e-mail: [email protected], [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Multiview geometry is the study of two-dimensional images of three-dimensional scenes, a foundational subject in computer vision. We determine a universal Gröbner basis for the multiview ideal of $n$ generic cameras. As the cameras move, the multiview varieties vary in a family of dimension 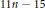$11n\,-\,15$. This family is the distinguished component of a multigraded Hilbert scheme with a unique Borel-fixed point. We present a combinatorial study of ideals lying on that Hilbert scheme.

Keywords

14N14Q68multigraded Hilbert Schemecomputer visionmonomial idealGroebner basisgeneric initial ideal
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 65 , Issue 5 , 01 October 2013 , pp. 961 - 988
Copyright
Copyright © Canadian Mathematical Society 2013

References

[1] Alzati, A. and Tortora, A., A geometric approach to the trifocal tensor. J. Math. Imaging Vision 38(2010), no. 3, 159–170. http://dx.doi.org/10.1007/s10851-010-0216-4 Google Scholar
[2] Cartwright, D., Häbich, M., Sturmfels, B., and Werner, A., Mustafin varieties. Selecta Math. 17(2011), no. 4, 757–793. http://dx.doi.org/10.1007/s00029-011-0075-x Google Scholar
[3] Cartwright, D. and Sturmfels, B., The Hilbert scheme of the diagonal in a product of projective spaces. Int. Math. Res. Not. 2010, no. 9, 1741–1771.Google Scholar
[4] Conca, A., Linear spaces, transversal polymatroids and ASL domains. J. Algebraic Combin. 25(2007), no. 1, 25–41. http://dx.doi.org/10.1007/s10801-006-0026-3 Google Scholar
[5] Cox, D., Little, J., O’Shea, and D., Ideals, varieties, and algorithms. An introduction to computational algebraic geometry and commutative algebra. Third ed. Undergraduate Texts in Mathematics, Springer, New York, 2007.Google Scholar
[6] Eisenbud, D., Commutative algebra with a view toward algebraic geometry. Graduate Texts in Mathematics, 150, Springer-Verlag, New York, 1995.Google Scholar
[7] Faugeras, O. and Luong, Q-T., The geometry of multiple images. The laws that govern the formation of multiple images of a scene and some of their applications. MIT Press, Cambridge, MA, 2001.Google Scholar
[8] Grayson, D. R. and Stillman, M. E., Macaulay2, a software system for research in algebraic geometry. http://www.math.uiuc.edu/Macaulay2/. Google Scholar
[9] Grosshans, F. D., On the equations relating a three-dimensional object and its two-dimensional images. Adv.in Appl. Math. 34(2005), no. 2, 366–392. http://dx.doi.org/10.1016/j.aam.2004.07.005 Google Scholar
[10] Haiman, M. and Sturmfels, B., Multigraded Hilbert schemes. J. Algebraic Geom. 13(2004), no. 4, 725–769. http://dx.doi.org/10.1090/S1056-3911-04-00373-X Google Scholar
[11] Hartley, R. and Zisserman, A, Multiple view geometry in computer vision. Second ed. Cambridge University Press, Cambridge, 2003.Google Scholar
[12] Heyden, A. and A°ström, K., Algebraic properties of multilinear constraints. Math. Methods Appl. Sci. 20(1997), no. 13, 1135–1162. http://dx.doi.org/10.1002/(SICI)1099-1476(19970910)20:13h1135::AID-MMA908i3.0.CO;2-9 Google Scholar
[13] Heyden, A., Tensorial properties of multiple view constraints. Math. Methods Appl. Sci. 23(2000), no. 2, 169–202. http://dx.doi.org/10.1002/(SICI)1099-1476(20000125)23:2h169::AID-MMA110i3.0.CO;2-Y Google Scholar
[14] Jensen, A., CaTS, a software system for toric state polytopes. http://www.soopadoopa.dk/anders/cats/cats.html. Google Scholar
[15] Jensen, A., Gfan, a software system for Gröbner fans and tropical varieties. http://www.math.tu-berlin.de/_jensen/software/gfan/gfan.html. Google Scholar
[16] D. Maclagan, and Sturmfels, B., Introduction to tropical geometry. http://www.warwick.ac.uk/staff/D.Maclagan/papers/papers.html. Google Scholar
[17] Miller, E. and Sturmfels, B., Combinatorial commutative algebra. Graduate Texts in Mathematics, 227, Springer, New York, 2005.Google Scholar
[18] Stanley, R., Combinatorics and commutative algebra. Second ed., Progress in Mathematics, 41, Birkhäuser Boston Inc., Boston, 1996.Google Scholar
[19] Stein, W. et. al, Sage Mathematics Software (Version 4.7). The Sage Development Team, 2011, http://www.sagemath.org. Google Scholar
[20] Sturmfels, B., Gröbner bases and convex polytopes. University Lecture Series, 8, American Mathematical Society, Providence, RI, 1996.Google Scholar
[21] Sturmfels, B. and Zelevinsky, A., Maximal minors and their leading terms. Adv. Math. 98(1993), no. 1, 65–112. http://dx.doi.org/10.1006/aima.1993.1013 Google Scholar