No CrossRef data available.
Article contents
Hardy Spaces of Conjugate Systems of Temperatures
Published online by Cambridge University Press: 20 November 2018
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
We define Hardy spaces of conjugate systems of temperature functions on 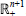 $\mathbb{R}_{+}^{n+1}$. We show that their boundary distributions are the same as the boundary distributions of the usual Hardy spaces of conjugate systems of harmonic functions.
$\mathbb{R}_{+}^{n+1}$. We show that their boundary distributions are the same as the boundary distributions of the usual Hardy spaces of conjugate systems of harmonic functions.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1998
References
1.
Baransky, F. and Musialek, J., On the Green functions for the heat equation over the m-dimensional cuboid. Demonstratio Math. (2) XIV (1981), 371–382.Google Scholar
2.
Bear, H.S., Hardy spaces of heat functions. Trans. Amer.Math. Soc. (2)
301(1987), 831–844.Google Scholar
3.
Cannon, J.R., The One-dimensional Heat Equation. Encyclopedia of Math. and its Appl.
23, Addison- Wesley, 1984.Google Scholar
4.
Fefferman, C. and Stein, E.M., Hp spaces of several variables. Acta Math.
129(1972), 137–193.Google Scholar
5.
Flett, T.M., Temperatures, Bessel potentials and Lipschitz spaces. Proc. LondonMath. Soc. (3)
22(1971), 385–451.Google Scholar
6.
García-Cuerva, J. and Rubio, J.L. de Francia,Weighted norm inequalities and related topics. NotasMat.
116, North Holland, Amsterdam, 1985.Google Scholar
7.
Guzmán-Partida, M., Hardy spaces of conjugate temperatures. Studia Math. (2)
122(1997), 153–165.Google Scholar
8.
Hirschman, I.I. and Widder, D.V., The Convolution Transform. Princeton Univ. Press, 1955.Google Scholar
9.
Kochneff, E. and Sagher, Y., Conjugate Temperatures. J. Approx. Theory (1)
70(1992), 39–49.Google Scholar
10.
Pérez-Esteva, S.,Hardy spaces of vector-valued heat functions. Houston J.Math. (1)
19(1993), 127–134.Google Scholar
11.
Riesz, M., L’integrale de Riemann-Liouville et le problem de Cauchy. Acta Math.
81(1949), 1–223. 12.
Stein, E.M., Harmonic Analysis. Real-variableMethods,Orthogonality and Oscillatory Integrals. Princeton Univ. Press, 1993.Google Scholar
13.
Stein, E.M. and Weiss, G., On the theory of harmonic functions of several variables. I. The theory of Hp spaces. Acta Math.
103(1960), 25–62.Google Scholar


