No CrossRef data available.
Published online by Cambridge University Press: 27 October 2023
Let 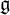 ${\mathfrak g}$ be a complex simple Lie algebra and
${\mathfrak g}$ be a complex simple Lie algebra and 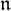 ${\mathfrak n}$ the nilradical of a parabolic subalgebra of
${\mathfrak n}$ the nilradical of a parabolic subalgebra of 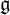 ${\mathfrak g}$. We consider some properties of the coadjoint representation of
${\mathfrak g}$. We consider some properties of the coadjoint representation of 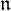 ${\mathfrak n}$ and related algebras of invariants. This includes (i) the problem of existence of generic stabilizers, (ii) a description of the Frobenius semiradical of
${\mathfrak n}$ and related algebras of invariants. This includes (i) the problem of existence of generic stabilizers, (ii) a description of the Frobenius semiradical of 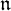 ${\mathfrak n}$ and the Poisson center
${\mathfrak n}$ and the Poisson center 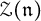 of the symmetric algebra
of the symmetric algebra  , (iii) the structure of
, (iii) the structure of 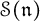 as
as  -module, and (iv) the description of square integrable (= quasi-reductive) nilradicals. Our main technical tools are the Kostant cascade in the set of positive roots of
-module, and (iv) the description of square integrable (= quasi-reductive) nilradicals. Our main technical tools are the Kostant cascade in the set of positive roots of 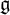 ${\mathfrak g}$ and the notion of optimization of
${\mathfrak g}$ and the notion of optimization of 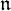 ${\mathfrak n}$.
${\mathfrak n}$.