Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-25T06:53:20.517Z Has data issue: false hasContentIssue false
  • English
  • Français

Fat Points in ℙ1 × ℙ1 and Their Hilbert Functions

Published online by Cambridge University Press:  20 November 2018

Elena Guardo  and
Adam Van Tuyl
Elena Guardo
Affiliation:
Dipartimento di Matematica e Informatica, Viale A. Doria 6 - 95100 – Catania, Italy e-mail: [email protected]
Adam Van Tuyl
Affiliation:
Department of Mathematics, Lakehead University, Thunder Bay, ON, P7B 5E1 e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We study the Hilbert functions of fat points in ${{\mathbb{P}}^{1\,}}\times \,{{\mathbb{P}}^{1}}$. If 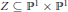$Z\,\subseteq \,{{\mathbb{P}}^{1\,}}\times \,{{\mathbb{P}}^{1}}$ is an arbitrary fat point scheme, then it can be shown that for every 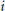$i$ and 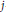$j$ the values of the Hilbert function 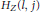${{H}_{Z}}(l,\,j)$ and ${{H}_{Z}}(i,\,l)$ eventually become constant for 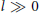$l\,\gg \,0$. We show how to determine these eventual values by using only the multiplicities of the points, and the relative positions of the points in ${{\mathbb{P}}^{1\,}}\times \,{{\mathbb{P}}^{1}}$. This enables us to compute all but a finite number values of 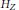${{H}_{Z}}$ without using the coordinates of points. We also characterize the 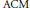$\text{ACM}$ fat point schemes using our description of the eventual behaviour. In fact, in the case that 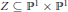$Z\,\subseteq \,{{\mathbb{P}}^{1\,}}\times \,{{\mathbb{P}}^{1}}$ is 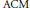$\text{ACM}$, then the entire Hilbert function and its minimal free resolution depend solely on knowing the eventual values of the Hilbert function.

Keywords

13D4013D0213H1014A15Hilbert functionpointsfat pointsCohen-Macaulay multi-projective space
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 56 , Issue 4 , 01 August 2004 , pp. 716 - 741
Copyright
Copyright © Canadian Mathematical Society 2004

References

[1] Capani, A., Niesi, G., Robbiano, L., CoCoA, a system for doing Computations in Commutative Algebra, Available via anonymous ftp from: cocoa.dima.unige.itGoogle Scholar
[2] Catalisano, M. V., Geramita, A. V., Gimigliano, A., Ranks of tensors, secant varieties of Segre Varieties and fat points. Linear Algebra Appl. 355(2002), 263–285.Google Scholar
[3] Giuffrida, S., Maggioni, R., Ragusa, A., On the postulation of 0-dimensional subschemes on a smooth quadric. Pacific J. Math. 155(1992), 251–282.Google Scholar
[4] Guardo, E., Schemi di “Fat Points”. Ph.D. Thesis, Universit à di Messina, 2000.Google Scholar
[5] Guardo, E., Fat point schemes on a smooth quadric. J. Pure Appl. Algebra 162(2001), 183–208.Google Scholar
[6] Harbourne, B., Problems and progress: a survey on fat points in ℙ2 . In: Zero-dimensional schemes and applications, Queen's Papers in Pure and Applied Mathematics, 123, 2002, pp. 85–132.Google Scholar
[7] Ryser, H. J., Combinatorial mathematics. The Carus Mathematical Monographs, No. 14. Mathematical Association of America, Providence, RI, 1963.Google Scholar
[8] Van Tuyl, A., The border of the Hilbert function of a set of points in ℙ n1 × · · · × ℙ nk . J. Pure Appl. Algebra 176(2002), 223–247.Google Scholar
[9] Van Tuyl, A., The Hilbert functions of ACM sets of points in ℙ n1 × · · · × ℙ nk . J. Algebra 264(2003), 420–441.Google Scholar