Hostname: page-component-745bb68f8f-cphqk Total loading time: 0 Render date: 2025-01-23T22:19:18.173Z Has data issue: false hasContentIssue false
  • English
  • Français

The Elliptic Integrals of the Third Kind

Published online by Cambridge University Press:  20 November 2018

E. H. Neville
E. H. Neville*
Affiliation:
Sonning-on- Thames, England
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

This paper develops a case for adopting as the standard elliptic integrals of the third kind the function IIs(u, a) defined by

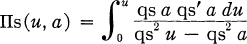
and the three functions lIs (u, a + Kc), Πs(u, a + Kn), Πss(u, a+ Kn) where KC, Kn, Kn are the three quarter-periods of the Jacobian system. The function Πs(u, a) is the same function whether qs u is cs u, ns u, or ds u.

The origin of the paper was a wish to understand how it has come about that the integrals commonly accepted as standard are not related symmetrically to the theta functions in terms of which they are expressed.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 11 , 1959 , pp. 175 - 194
Copyright
Copyright © Canadian Mathematical Society 1959

References

1. A. Briot, C. A. et Bouquet, J. C., Théorie des Fonctions elliptiques, (Paris, 1875).Google Scholar
2. Enneper, A., Elliptische Funktionen, Theorie und Geschichte (Halle, 1876).Google Scholar
3. Hermite, C., Note sur la théorie des fonctions elliptiques in Serret, Cours de calcul différentiel et intégral (4th ed, Paris, 1894) 737–904.Google Scholar
4. Jacobi, C. G.J., Fundamenta nova theoriae functionum ellipticarum (Königsburg, 1829).Google Scholar
5. Legendre, A. M., Exercices de calcul intégral (Paris, 1811).Google Scholar
6. Legendre, A. M. Théorie des fonctions elliptiques, t.l (Paris, 1825).Google Scholar
7. Lenz, H., Uber die elliptischen Funktionen von Jacobi, Math. Zeit., 67 (1957), 153–175.Google Scholar
8. Neville, E. H., Jacobian Elliptic Functions (2nd ed., Cambridge, 1951).Google Scholar