Hostname: page-component-586b7cd67f-l7hp2 Total loading time: 0 Render date: 2024-11-24T07:44:57.542Z Has data issue: false hasContentIssue false
  • English
  • Français

Eigenvalue Optimisation on Flat Tori and Lattice Points in Anisotropically Expanding Domains

Part of: Geometry of numbers Discrete geometry Spectral theory and eigenvalue problems

Published online by Cambridge University Press:  07 March 2019

Jean Lagacé
Jean Lagacé*
Affiliation:
Department of Mathematics, University College London, Gower Street, London, WC1E 6BT, United Kingdom Email: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper is concerned with the maximisation of the 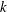$k$-th eigenvalue of the Laplacian amongst flat tori of unit volume in dimension 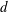$d$ as 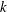$k$ goes to infinity. We show that in any dimension maximisers exist for any given 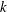$k$, but that any sequence of maximisers degenerates as 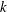$k$ goes to infinity when the dimension is at most 10. Furthermore, we obtain specific upper and lower bounds for the injectivity radius of any sequence of maximisers. We also prove that flat Klein bottles maximising the 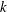$k$-th eigenvalue of the Laplacian exhibit the same behaviour. These results contrast with those obtained recently by Gittins and Larson, stating that sequences of optimal cuboids for either Dirichlet or Neumann boundary conditions converge to the cube no matter the dimension. We obtain these results via Weyl asymptotics with explicit control of the remainder in terms of the injectivity radius. We reduce the problem at hand to counting lattice points inside anisotropically expanding domains, where we generalise methods of Yu. Kordyukov and A. Yakovlev by considering domains that expand at different rates in various directions.

Keywords

spectral optimisationLaplacianeigenvalueasymptotics

MSC classification

Primary: 35P20: Asymptotic distribution of eigenvalues and eigenfunctions
Secondary: 11H06: Lattices and convex bodies 52C07: Lattices and convex bodies in $n$ dimensions
Type
Article
Information
Canadian Journal of Mathematics , Volume 72 , Issue 4 , August 2020 , pp. 967 - 987
Check for updates
Copyright
© Canadian Mathematical Society 2019

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The research of the author was supported by NSERC’s Alexander-Graham-Bell doctoral scholarship.

References

Antunes, P. R. S. and Freitas, P., Numerical optimization of low eigenvalues of the Dirichlet and Neumann Laplacians. J. Optim. Theory Appl. 154(2012), 235–257. https://doi.org/10.1007/s10957-011-9983-3Google Scholar
Antunes, P. R. S. and Freitas, P., Optimal spectral rectangles and lattice ellipses. Proc. R. Soc. Lond. Ser. A Math. Phys. Eng. Sci. 469(2013), no. 2015, 20120492. https://doi.org/10.1098/rspa.2012.0492Google Scholar
Banaszczyk, W., New bounds in some transference theorems in the geometry of numbers. Math. Ann. 296(1993), 625–635. https://doi.org/10.1007/BF01445125Google Scholar
van den Berg, M., Bucur, D., and Gittins, K., Maximising Neumann eigenvalues on rectangles. Bull. Lond. Math. Soc. 48(2016), 877–894. https://doi.org/10.1112/blms/bdw049Google Scholar
van den Berg, M. and Gittins, K., Minimizing Dirichlet eigenvalues on cuboids of unit measure. Mathematika 63(2017), 469–482. https://doi.org/10.1112/S0025579316000413Google Scholar
Berger, A., The eigenvalues of the Laplacian with Dirichlet boundary condition in ℝ2 are almost never minimized by disks. Ann. Global Anal. Geom. 47(2015), 285–304. https://doi.org/10.1007/s10455-014-9446-9Google Scholar
Berger, M., Gauduchon, P., and Mazet, E., Le spectre d’une variété riemannienne. Lecture Notes in Mathematics, 194, Springer-Verlag, Berlin-New York, 1971.Google Scholar
Buser, P., A note on the isoperimetric constant. Ann. Sci. École Norm. Sup. (4) 15(1982), 213–230.Google Scholar
Cassels, J. W. S., An introduction to the geometry of numbers. Die Grundlehren der mathematischen Wissenschaften, Band 99, Springer-Verlag, Berlin-New York, 1971.Google Scholar
Colbois, B. and Dodziuk, J., Riemannian metrics with large 𝜆1. Proc. Amer. Math. Soc. 122(1994), 905–906. https://doi.org/10.2307/2160770Google Scholar
Duistermaat, J. J. and Guillemin, V. W., The spectrum of positive elliptic operators and periodic bicharacteristics. Invent. Math. 29(1975), 39–79. https://doi.org/10.1007/BF01405172Google Scholar
Faber, G., Beweis, dass unter allen homogenen Membranen von gleicher Fläche und gleicher Spannung die kreisförmige den tiefsten Grundton gibt. Sitzungsber. Bayer. Akad. Wiss. München, Math.-Phys. Kl., 1923, pp. 169–172.Google Scholar
Gittins, K. and Larson, S., Asymptotic behaviour of cuboids optimising Laplacian eigenvalues. Integral Equations Operator Theory 89(2017), 607–629. https://doi.org/10.1007/s00020-017-2407-5Google Scholar
Hassannezhad, A., Kokarev, G., and Polterovich, I., Eigenvalue inequalities on Riemannian manifolds with a lower Ricci curvature bound. J. Spectr. Theory 6(2016), 807–835. https://doi.org/10.4171/JST/143Google Scholar
Hersch, J., Quatre propriétés isopérimétriques de membranes sphériques homogènes. C. R. Acad. Sci. Paris Sér. A-B 270(1970), A1645–A1648.Google Scholar
Iosevich, A. and Liflyand, E., Decay of the Fourier transform, analytic and geometric aspects. Birkhäuser/Springer, Basel, 2014. https://doi.org/10.1007/978-3-0348-0625-1Google Scholar
Kao, C.-Y., Lai, R., and Osting, B., Maximization of Laplace-Beltrami eigenvalues on closed Riemannian surfaces. ESAIM Control Optim. Calc. Var. 23(2017), 685–720. https://doi.org/10.1051/cocv/2016008Google Scholar
Karpukhin, M., Nadirashvili, N., Penskoi, A. V., and Polterovich, I., An isoperimetric inequality for Laplace eigenvalues on the sphere. J. Diff. Geom., to appear.Google Scholar
Kordyukov, Yu. A. and Yakovlev, A. A., Lattice points in domains and adiabatic limits. (Russian) Algebra i Analiz 23(2011), 80–95. https://doi.org/10.1090/S1061-0022-2012-01225-2Google Scholar
Kordyukov, Yu. A. and Yakovlev, A. A., The problem of the number of integer points in families of anisotropically expanding domains, with applications to spectral theory. Mat. Zametki 92(2012); trans. in Math. Notes 92(2012), no. 3–4, 574–576. https://doi.org/10.1134/S0001434612090295Google Scholar
Kordyukov, Yu. A. and Yakovlev, A. A., The number of integer points in a family of anisotropically expanding domains. Monatsh. Math. 178(2015), 97–111. https://doi.org/10.1007/s00605-015-0787-7Google Scholar
Kordyukov, Yu. A. and Yakovlev, A. A., On a problem in geometry of numbers arising in spectral theory. Russ. J. Math. Phys. 22(2015), 473–482. https://doi.org/10.1134/S106192081504007XGoogle Scholar
Krahn, E., Über eine von Rayleigh formulierte Minimaleigenschaft des Kreises. Math. Ann. 94(1925), 97–100. https://doi.org/10.1007/BF01208645Google Scholar
Krahn, E., Über Minimaleigenschaften der Kugel in drei und mehr Dimensionen. Acta Comm. Univ. Tartu (Dorpat) A9(1926), 1–44.Google Scholar
Lagacé, J. and Parnovski, L., A generalised Gauss circle problem and integrated density of states. J. Spectr. Theory 6(2016), 859–879. https://doi.org/10.4171/JST/145Google Scholar
Nadirashvili, N., Berger’s isoperimetric problem and minimal immersions of surfaces. Geom. Funct. Anal. 6(1996), 877–897. https://doi.org/10.1007/BF02246788Google Scholar
Szegö, G., Inequalities for certain eigenvalues of a membrane of given area. J. Rational Mech. Anal. 3(1954), 343–356. https://doi.org/10.1512/iumj.1954.3.53017Google Scholar
Weinberger, H. F., An isoperimetric inequality for the N-dimensional free membrane problem. J. Rational Mech. Anal. 5(1956), 633–636. https://doi.org/10.1512/iumj.1956.5.55021Google Scholar