Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-27T20:58:51.491Z Has data issue: false hasContentIssue false
  • English
  • Français

Divisibility of torsion subgroups of abelian surfaces over number fields

Part of: Linear algebraic groups and related topics Arithmetic algebraic geometry

Published online by Cambridge University Press:  28 October 2020

John Cullinan  and
Jeffrey Yelton
John Cullinan*
Affiliation:
Department of Mathematics, Bard College, Annandale-On-Hudson, NY12401, USA
Jeffrey Yelton
Affiliation:
Department of Mathematics and Computer Science, Emory University, Atlanta, GA 30322, USA e-mail: [email protected]
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let A be a two-dimensional abelian variety defined over a number field K. Fix a prime number 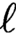$\ell $ and suppose 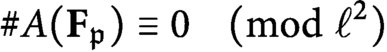$\#A({\mathbf {F}_{\mathfrak {p}}}) \equiv 0 \pmod {\ell ^2}$ for a set of primes 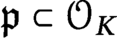${\mathfrak {p}} \subset {\mathcal {O}_{K}}$ of density 1. When $\ell =2$ Serre has shown that there does not necessarily exist a K-isogenous $A'$ such that 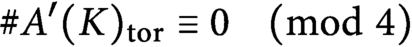$\#A'(K)_{{tor}} \equiv 0 \pmod {4}$ . We extend those results to all odd 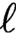$\ell $ and classify the abelian varieties that fail this divisibility principle for torsion in terms of the image of the mod-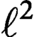 $\ell ^2$ representation.

Keywords

Abelian varietytorsion pointsℓ-adic representation

MSC classification

Primary: 11G10: Abelian varieties of dimension $> 1$
Secondary: 20G25: Linear algebraic groups over local fields and their integers
Type
Article
Information
Canadian Journal of Mathematics , Volume 74 , Issue 1 , February 2022 , pp. 266 - 298
Check for updates
Copyright
© Canadian Mathematical Society 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Booker, A., Sijsling, J., Sutherland, A., Voight, J., and Yasaki, D., A database of genus-2 curves over the rational numbers. LMS J. Comput. Math. 19(2016), suppl. A, 235–254.CrossRefGoogle Scholar
Cullinan, J., A computational approach to the 2-torsion structure of abelian threefolds. Math. Comput. 78(2009), no. 267, 1825–1836.CrossRefGoogle Scholar
Katz, N., Galois properties of torsion points on abelian varieties. Invent. Math. 62(1981), 481–502.CrossRefGoogle Scholar
Landesman, A., Swaminathan, A., Tao, J., and Xu, Y., Lifting subgroups of symplectic groups over $\boldsymbol{Z}/ \ell Z$ . Res. Number Theory 3(2017), no. 14, 12 pp.CrossRefGoogle Scholar
Landesman, A., Swaminathan, A., Tao, J., and Xu, Y., Hyperelliptic curves with maximal Galois action on the torsion points of their Jacobians. Preprint, 2017. https://arxiv.org/pdf/1705.08777.pdf Google Scholar
Lang, S., Algebra . Revised 3rd ed., Graduate Texts in Mathematics, 211, Springer-Verlag, New York, NY, 2002.CrossRefGoogle Scholar
The LMFDB Collaboration, The L-functions and modular forms database. 2019. http://www.lmfdb.org Google Scholar
Milne, J. S., Abelian varieties . In: Arithmetic geometry (Storrs, Conn., 1984), Springer, New York, NY, 1986, pp. 103–150.Google Scholar
O’Meara, O. T., Symplectic groups, mathematical surveys . Vol. 16. Amer. Math. Soc., Providence, RI, 1978.CrossRefGoogle Scholar
Serre, J.-P., Abelian l-adic representations and elliptic curves . Research Notes in Mathematics, 7, A K Peters, Ltd., Wellesley, MA, 1998.Google Scholar
Serre, J.-P., Letter to N. Katz. Unpublished.Google Scholar
Testerman, D., A1-type overgroups of elements of order $p$ in semisimple algebraic groups and the associated finite groups. J. Algebra 177(1995), no. 1, 34–76.CrossRefGoogle Scholar