Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-25T06:18:03.234Z Has data issue: false hasContentIssue false
  • English
  • Français

Distance Sets of Urysohn Metric Spaces

Published online by Cambridge University Press:  20 November 2018

N.W. Sauer
N.W. Sauer*
Affiliation:
Department of Mathematics and Statistics, University of Calgary, Calgary, AB, e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

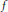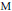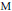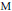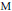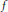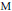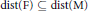A metric space $\text{M=}\left( M;\text{d} \right)$ is homogeneous if for every isometry $f$ of a finite subspace of $\text{M}$ to a subspace of $\text{M}$ there exists an isometry of $\text{M}$ onto $\text{M}$ extending $f$. The space $\text{M}$ is universal if it isometrically embeds every finite metric space $\text{F}$ with $\text{dist}\left( \text{F} \right)\subseteq \text{dist}\left( \text{M} \right)$ (with $\text{dist}\left( \text{M} \right)$ being the set of distances between points in 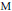$\text{M}$).

A metric space 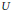$U$ is a Urysohn metric space if it is homogeneous, universal, separable, and complete. (We deduce as a corollary that a Urysohn metric space 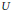$U$ isometrically embeds every separable metric space 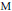$\text{M}$ with 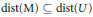$\text{dist}\left( \text{M} \right)\subseteq \text{dist}\left( U \right)$.)

The main results are: (1) A characterization of the sets 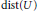$\text{dist}\left( U \right)$ for Urysohn metric spaces 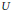$U$. (2) If $R$ is the distance set of a Urysohn metric space and 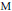$\text{M}$ and 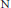$\text{N}$ are two metric spaces, of any cardinality with distances in $R$, then they amalgamate disjointly to a metric space with distances in $R$. (3) The completion of every homogeneous, universal, separable metric space 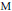$\text{M}$ is homogeneous.

Keywords

03E0222F0505C5505D1022A0551F99partitions of metric spacesRamsey theorymetric geometryUrysohn metric spaceoscillation stability
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 65 , Issue 1 , 01 February 2013 , pp. 222 - 240
Copyright
Copyright © Canadian Mathematical Society 2013

References

[1] Barbina, S. and Zambella, D., A viewpoint on amalgamation classes. Comment. Math. Univ. Carolin. 51(2010), no. 4, 681–691.Google Scholar
[2] Clemens, J., The set of distances in a Polish metric space..Fundamenta Math., to appear, http://www.math.psu.edu/clemens/Papers/distances.pdf Google Scholar
[3]Delhommé, C., Laflamme, C., Pouzet, M., and Sauer, N. Divisibility of countable metric spaces. European J. Combin. 28(2007), no. 6, 1746–1769. http://dx.doi.org/10.1016/j.ejc.2006.06.024 Google Scholar
[4] Fraϊssé, R., Theory of relations. Studies in Logic and the Foundations of Mathematics, 145, North-Holland Publishing Co., Amsterdam, 2000.Google Scholar
[5]Katětov, M., On universal metric spaces. In: General topology and its relations to modern analysis and algebra, VI (Prague, 1986), Res. Exp. Math., 16, Heldermann, Berlin, 1988.Google Scholar
[6]Kechris, A. S., Pestov, V. G., and Todorcevic, S., Fraϊssé limits, Ramsey theory, and topological dynamics of automorphism groups. Geom. Funct. Anal. 15(2005), no. 1, 106–189. http://dx.doi.org/10.1007/s00039-005-0503-1 Google Scholar
[7] Jόnsson, B., Homogeneous universal relational systems. Math. Scand. 8(1960), 137–142.Google Scholar
[8] Lopez-Abad, J. and Nguyen Van, L. Thě, The oscillation stability problem for the Urysohn sphere: A combinatorial approach. Topology Appl. 155(2008), no. 14, 1516–1530.http://dx.doi.org/10.1016/j.topol.2008.03.011 Google Scholar
[9] Mbombo, B. and Pestov, V., Subgroups of isometries of Urysohn-Katĕtov metric spaces of uncountable density..arxiv:1012.1056v6 Google Scholar
[10] Melleray, J., Topology of the isometry group of the Urysohn space. Fund. Math. 207(2010), no. 3, 273–287. http://dx.doi.org/10.4064/fm207-3-4 Google Scholar
[11]Milman, V. D., A new proof of A. Dvoretzky's theorem on cross-sections of convex bodies. (Russian) Funkcional. Anal. i Priložen. 5(1971), no. 4, 28–37.Google Scholar
[12] Nguyen, L. Van Thé, Structural Ramsey theory of metric spaces and topological dynamics of isometry groups. Mem. Amer. Math. Soc. 206(2010), no. 968.Google Scholar
[13] Nguyen, L., Théorie de Ramsey structurale des espaces m´etriques et dynamique topologique des groupes d’isom´etries. Ph.D. Thesis, Université Paris 7, 2006 (available in English).Google Scholar
[14] Nguyen, L. Van Thé and Sauer, N.W., The Urysohn sphere is oscillation stable. Goem. Funct. Anal. 19(2009), no. 2, 536–557. http://dx.doi.org/10.1007/s00039-009-0007-5 Google Scholar
[15] Nguyen, L., Some weak indivisibility results in ultrahomogeneous metric spaces. European J. Combin. 31(2010), no. 5, 1464–1483. http://dx.doi.org/10.1016/j.ejc.2010.01.003 Google Scholar
[16] Odell, E. and Schlumprecht, T., The distortion problem. Acta Math. 173(1994), no. 2, 259–281. http://dx.doi.org/10.1007/BF02398436 Google Scholar
[17]Pestov, V., Dynamics of infinite-dimensional groups. The Ramsey-Dvoretzky-Milman phenomenon. Revised edition of Dynamics of infinite-dimensional groups and Ramsey-type phenomena [Inst. Mat. Pura. Apl. (IMPA), Rio de Janeiro, 2005]. University Lecture Series, 40, American Mathematical Society, Providence, RI, 2006.Google Scholar
[18] Sauer, N.W., Vertex partitions of metric spaces with finite distance sets. Discrete Math. 312(2012), no. 1, 119–128. http://dx.doi.org/10.1016/j.disc.2011.06.002 Google Scholar
[19] Urysohn, P. S., Sur un espace métrique universel. C. R. Acad. Sci. Paris 180 (1925), 803–806.Google Scholar
[20] Uspenskij, V. V., On the group of isometries of the Urysohn universal metric space. Comment. Math. Univ. Carolin. 31(1990), no. 1, 181–182.Google Scholar
[21] Uspenskij, V. V., On subgroups of minimal topological groups. Topology Appl. 155(2008), no. 14, 1580–1606. http://dx.doi.org/10.1016/j.topol.2008.03.001 Google Scholar