Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-26T17:48:42.810Z Has data issue: false hasContentIssue false
  • English
  • Français

Discrete Convex Functions and Proof of the Six Circle Conjecture of Fejes Tóth

Published online by Cambridge University Press:  20 November 2018

Imre Bárány ,
Zoltán Füredi  and
János Pach
Imre Bárány
Affiliation:
Hungarian Academy of Sciences, Budapest, Hungary
Zoltán Füredi
Affiliation:
Hungarian Academy of Sciences, Budapest, Hungary
János Pach
Affiliation:
Hungarian Academy of Sciences, Budapest, Hungary
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

A system 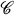of openly disjoint discs in the plane is said to form a 6-neighboured circle packing if every 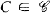is tangent to at least 6 other elements of 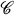(It is evident that such a system consists of infinitely many discs.) The simplest example is the regular circle packing all of whose circles are of the same size and have exactly 6 neighbours. L. Fejes Tóth conjectured that the regular circle packing has the interesting extremal property that, if we slightly “perturb” it, then there will necessarily occur either arbitrarily small or arbitrarily large circles. More precisely, he asked whether or not the following “zero or one law” (cf. [3], [6]) is valid: If 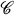is a 6-neighboured circle packing, then

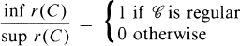
where r(C) denotes the radius of circle C, inf and sup are taken over all C ∊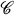

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 36 , Issue 3 , 01 June 1984 , pp. 569 - 576
Copyright
Copyright © Canadian Mathematical Society 1984

References

1. Beckenbach, E. F. and Bellman, R., Inequalities (Springer, Berlin-Göttingen-Heidelberg, 1961).CrossRefGoogle Scholar
2. Dynkin, E. B. and Juschkewitsch, A. A., Sdtze unci Aufgaben uber Markoffsche Prozesse (Springer, Berlin-Heidelberg-New York, 1969).CrossRefGoogle Scholar
3. Tóth, L. Fejes, Research problem, Periodica Math. Hung. 8 (1977), 103–104.Google Scholar
4. Tóth, L. Fejes, Compact packing of circles, Studia Sci. Math Hungar. (to appear).Google Scholar
5. Karamata, J., Sur une inégalité relative aux fonctions convexes, Publ. Math. Univ. Belgrade 1 (1932), 145–148.Google Scholar
6. Rybnikov, K. A., Problems of combinatorial analysis (in Russian) (MIR Publ. Co., Moscow, 1980).Google Scholar