Article contents
A Difference-Differential Basis Theorem
Published online by Cambridge University Press: 20 November 2018
Extract
Our aim in this paper is to extend to difference-differential rings the beautiful theorem of Kolchin [5, Theorem 3] for the differential case. The necessity portion of Kolchin's result is not obtained.
What might well be called the Ritt basis theorem states that if a commutative ring R with identity is finitely generated over a subring R0, then the ascending chain condition for radical ideals of R0 implies the ascending chain condition for radical ideals of R. (This is indeed a basis theorem. If we define a basis for a radical ideal A to be a finite set B such that 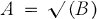 then every radical ideal of a ring R has a basis if and only if the ascending chain condition for radical ideals holds in R.) It is the Ritt basis theorem rather than the Hilbert basis theorem which has appropriate generalizations in differential and difference algebra, where in fact it originated.
then every radical ideal of a ring R has a basis if and only if the ascending chain condition for radical ideals holds in R.) It is the Ritt basis theorem rather than the Hilbert basis theorem which has appropriate generalizations in differential and difference algebra, where in fact it originated.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1970
References
- 10
- Cited by


