Article contents
Défaut de semi-stabilité des courbes elliptiques dans le cas non ramifié
Published online by Cambridge University Press: 20 November 2018
Abstract
Let 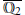 $\overline{{{\mathbb{Q}}_{2}}}$ be an algebraic closure of
$\overline{{{\mathbb{Q}}_{2}}}$ be an algebraic closure of 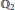 ${{\mathbb{Q}}_{2}}$ and
${{\mathbb{Q}}_{2}}$ and 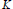 $K$ be an unramified finite extension of
$K$ be an unramified finite extension of 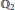 ${{\mathbb{Q}}_{2}}$ included in
${{\mathbb{Q}}_{2}}$ included in 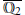 $\overline{{{\mathbb{Q}}_{2}}}$. Let
$\overline{{{\mathbb{Q}}_{2}}}$. Let  $E$ be an elliptic curve defined over
$E$ be an elliptic curve defined over 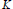 $K$ with additive reduction over
$K$ with additive reduction over 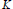 $K$, and having an integral modular invariant. Let us denote by
$K$, and having an integral modular invariant. Let us denote by 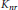 ${{K}_{nr}}$ the maximal unramified extension of
${{K}_{nr}}$ the maximal unramified extension of 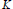 $K$ contained in
$K$ contained in 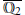 $\overline{{{\mathbb{Q}}_{2}}}$. There exists a smallest finite extension
$\overline{{{\mathbb{Q}}_{2}}}$. There exists a smallest finite extension  $L$ of
$L$ of 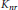 ${{K}_{nr}}$ over which
${{K}_{nr}}$ over which  $E$ has good reduction. We determine in this paper the degree of the extension
$E$ has good reduction. We determine in this paper the degree of the extension 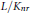 $L/{{K}_{nr}}$.
$L/{{K}_{nr}}$.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2004
References
Références
- 1
- Cited by


