Article contents
Convexité, complête monotonie et inégalités sur les fonctions zêta et gamma, sur les fonctions des opérateurs de Baskakov et sur des fonctions arithmétiques
Published online by Cambridge University Press: 20 November 2018
Résumé
Nous prouvons un encadrement optimal pour la quantité 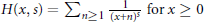 $H\left( x,\,s \right)\,=\,\sum{_{n\ge 1}\,\frac{1}{{{\left( x+n \right)}^{s}}}}$ pour
$H\left( x,\,s \right)\,=\,\sum{_{n\ge 1}\,\frac{1}{{{\left( x+n \right)}^{s}}}}$ pour 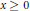 $x\,\ge \,0$ et
$x\,\ge \,0$ et 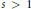 $s\,>\,1$, qui améliore l'encadrement standard par des intégrales. Cet encadrement entraîne des inégalités sur la fonction
$s\,>\,1$, qui améliore l'encadrement standard par des intégrales. Cet encadrement entraîne des inégalités sur la fonction  $\zeta $ de Riemann, et amène à conjecturer la monotonie de la fonction
$\zeta $ de Riemann, et amène à conjecturer la monotonie de la fonction 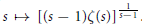 $s\,\mapsto \,{{[(s\,-\,1)\text{ }\!\!\zeta\!\!\text{ (}s\text{)}]}^{\frac{1}{S-1}}}$. On donne des applications à l'étude de la convexité de fonctions liées à la fonction
$s\,\mapsto \,{{[(s\,-\,1)\text{ }\!\!\zeta\!\!\text{ (}s\text{)}]}^{\frac{1}{S-1}}}$. On donne des applications à l'étude de la convexité de fonctions liées à la fonction  $\Gamma $ d'Euler et à la majoration optimale des fonctions élémentaires intervenant dans les opérateurs de Baskakov. Puis, nous étendons aux fonctions complètement monotones sur ]
$\Gamma $ d'Euler et à la majoration optimale des fonctions élémentaires intervenant dans les opérateurs de Baskakov. Puis, nous étendons aux fonctions complètement monotones sur ] $0,\,+\infty $[ les résultats établis pour la fonction
$0,\,+\infty $[ les résultats établis pour la fonction 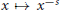 $x\,\mapsto \,{{x}^{-s}}$, et nous en déduisons des preuves élémentaires du comportement, quand
$x\,\mapsto \,{{x}^{-s}}$, et nous en déduisons des preuves élémentaires du comportement, quand  $z$ tend vers 1, des séries génératrices de certaines fonctions arithmétiques. Enfin, nous prouvons qu'une partie du résultat se généralise à une classe de fonctions convexes positives décroissantes.
$z$ tend vers 1, des séries génératrices de certaines fonctions arithmétiques. Enfin, nous prouvons qu'une partie du résultat se généralise à une classe de fonctions convexes positives décroissantes.
Abstract
We give optimal upper and lower bounds for the function 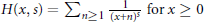 $H\left( x,\,s \right)\,=\,\sum{_{n\ge 1}\,\frac{1}{{{\left( x+n \right)}^{s}}}}$ for
$H\left( x,\,s \right)\,=\,\sum{_{n\ge 1}\,\frac{1}{{{\left( x+n \right)}^{s}}}}$ for 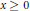 $x\,\ge \,0$ and
$x\,\ge \,0$ and 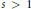 $s\,>\,1$. These bounds improve the standard inequalities with integrals. We deduce from them inequalities about Riemann's
$s\,>\,1$. These bounds improve the standard inequalities with integrals. We deduce from them inequalities about Riemann's  $\zeta $ function, and we give a conjecture about the monotonicity of the function
$\zeta $ function, and we give a conjecture about the monotonicity of the function 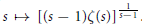 $s\,\mapsto \,{{[(s\,-\,1)\text{ }\!\!\zeta\!\!\text{ (}s\text{)}]}^{\frac{1}{S-1}}}$. Some applications concern the convexity of functions related to Euler's
$s\,\mapsto \,{{[(s\,-\,1)\text{ }\!\!\zeta\!\!\text{ (}s\text{)}]}^{\frac{1}{S-1}}}$. Some applications concern the convexity of functions related to Euler's  $\Gamma $ function and optimal majorization of elementary functions of Baskakov's operators. Then, the result proved for the function
$\Gamma $ function and optimal majorization of elementary functions of Baskakov's operators. Then, the result proved for the function 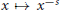 $x\,\mapsto \,{{x}^{-s}}$ is extended to completely monotonic functions. This leads to easy evaluation of the order of the generating series of some arithmetical functions when
$x\,\mapsto \,{{x}^{-s}}$ is extended to completely monotonic functions. This leads to easy evaluation of the order of the generating series of some arithmetical functions when  $z$ tends to 1. The last part is concerned with the class of non negative decreasing convex functions on ]
$z$ tends to 1. The last part is concerned with the class of non negative decreasing convex functions on ] $0,\,+\infty $[, integrable at infinity.
$0,\,+\infty $[, integrable at infinity.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 2002
References
Références
- 18
- Cited by


