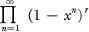Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Newman, Morris
1960.
Periodicity modulo 𝑚 and divisibility properties of the partition function.
Transactions of the American Mathematical Society,
Vol. 97,
Issue. 2,
p.
225.
Gandhi, J. M.
1963.
Congruences for Þor(n) and Ramanujan's τ Function.
The American Mathematical Monthly,
Vol. 70,
Issue. 3,
p.
265.
Yaqub, Adil
Brauer, George
Khandekar, P. R.
Newman, Morris
Carlitz, L.
Bilodeau, G. G.
and
Burton, David M.
1964.
Mathematical Notes.
The American Mathematical Monthly,
Vol. 71,
Issue. 9,
p.
1010.
Arkin, Joseph
1966.
An Extension of a Theorem of Ramanujan.
The American Mathematical Monthly,
Vol. 73,
Issue. 10,
p.
1087.
Rankin, R. A.
1977.
Modular Functions of one Variable V.
Vol. 601,
Issue. ,
p.
3.
Ramanathan, K G
1980.
Ramanujan and the congruence properties of partitions.
Proceedings of the Indian Academy of Sciences - Section A,
Vol. 89,
Issue. 3,
Fomenko, O. M.
1980.
Applications of the theory of modular forms to number theory.
Journal of Soviet Mathematics,
Vol. 14,
Issue. 4,
p.
1307.
MacLean, D. W.
1980.
Residue classes of the partition function.
Mathematics of Computation,
Vol. 34,
Issue. 149,
p.
313.
Berndt, Bruce C.
and
Ono, Ken
2001.
The Andrews Festschrift.
p.
39.
Ono, Ken
2010.
Parity of the partition function.
Advances in Mathematics,
Vol. 225,
Issue. 1,
p.
349.
Ono, Ken
2011.
The partition function and Hecke operators.
Advances in Mathematics,
Vol. 228,
Issue. 1,
p.
527.
BARUAH, NAYANDEEP DEKA
and
OJAH, KANAN KUMARI
2011.
SOME CONGRUENCES DEDUCIBLE FROM RAMANUJAN'S CUBIC CONTINUED FRACTION.
International Journal of Number Theory,
Vol. 07,
Issue. 05,
p.
1331.
Narkiewicz, Władysław
2012.
Rational Number Theory in the 20th Century.
p.
13.
Andrews, George E.
and
Berndt, Bruce C.
2012.
Ramanujan's Lost Notebook.
p.
181.
Berndt, Bruce C.
Gugg, Chadwick
and
Kim, Sun
2012.
Partitions, q-Series, and Modular Forms.
Vol. 23,
Issue. ,
p.
13.
Andrews, George E.
and
Berndt, Bruce C.
2012.
Ramanujan's Lost Notebook.
p.
89.
Folsom, Amanda
Kent, Zachary A.
and
Ono, Ken
2012.
ℓ-Adic properties of the partition function.
Advances in Mathematics,
Vol. 229,
Issue. 3,
p.
1586.
Baruah, Nayandeep Deka
and
Sarmah, Bipul Kumar
2013.
Identities and congruences for the general partition and Ramanujan’s tau functions.
Indian Journal of Pure and Applied Mathematics,
Vol. 44,
Issue. 5,
p.
643.
Choi, Dohoon
2016.
Congruences involving arithmetic progressions for weakly holomorphic modular forms.
Advances in Mathematics,
Vol. 294,
Issue. ,
p.
489.
Saikia, Nipen
and
Chetry, Jubaraj
2018.
Infinite families of congruences modulo 7 for Ramanujan’s general partition function.
Annales mathématiques du Québec,
Vol. 42,
Issue. 1,
p.
127.