Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-18T18:42:32.006Z Has data issue: false hasContentIssue false
  • English
  • Français

A Class of Abstract Linear Representations for Convolution Function Algebras overHomogeneous Spaces of Compact Groups

Published online by Cambridge University Press:  20 November 2018

Arash Ghaani Farashahi
Arash Ghaani Farashahi*
Affiliation:
Numerical Harmonic Analysis Group (NuHAG), Faculty of Mathematics, University of Vienna e-mail: [email protected]@hotmail.com
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

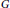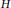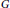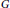This paper introduces a class of abstract linear representations on Banach convolution function algebras over homogeneous spaces of compact groups. Let $G$ be a compact group and $H$ a closed subgroup of $G$. Let $\mu $ be the normalized $G$-invariant measure over the compact homogeneous space $G/H$ associated with Weil's formula and 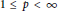$1\,\le \,p\,<\,\infty $. We then present a structured class of abstract linear representations of the Banach convolution function algebras 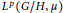${{L}^{p}}\left( G/H,\,\mu \right)$.

Keywords

43A8547A6720G05homogeneous spacelinear representationcontinuous unitary representationconvolution function algebracompact groupconvolutioninvolution
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 70 , Issue 1 , 01 February 2018 , pp. 97 - 116
Copyright
Copyright © Canadian Mathematical Society 2018

References

[1] Derighetti, A., Á propos des convoluteurs d'un groupe quotient. Bull. Sci. Math. (2) 107(1983), no. 1, 3–23.Google Scholar
[2] Derighetti, A., Convolution operators on groups. Lecture Notes of the Unione Matematica Italiana, 11.Springer, Heidelberg, 2011.http://dx.doi.org/10.1007/978-3-642-20656-6 Google Scholar
[3] Dixmier, J., C* -algebras. North-Holland Mathematical Library, 15. North-Holland, Amsterdam, 1977.Google Scholar
[4] Feichtinger, H. G., On a class of convolution algebras of functions. Ann. Inst. Fourier (Grenoble) 27(1977), no. 3, 135–162.Google Scholar
[5] Feichtinger, H. G., Banach convolution algebras of functions. II. Monatsh. Math. 87(1979), no. 3,181–207. http://dx.doi.Org/10.1007/BF01303075 Google Scholar
[6] Feichtinger, H. G., On a new Segal algebra. Monatsh. Math. 92(1981), no. 4, 269–289. http://dx.doi.Org/10.1007/BF01320058 Google Scholar
[7] Folland, G. B., A course in abstract harmonic analysis. Studies in Advanced Mathematics. CRC Press, Boca Raton, FL, 1995.Google Scholar
[8] Ghaani Farashahi, A., Abstract non-commutative harmonic analysis of coherent state transforms. Ph.D. thesis, Ferdowsi University of Mashhad, 2012.Google Scholar
[9] Ghaani Farashahi, A., Convolution and involution on function spaces of homogeneous spaces. Bull. Malays. Math. Sci. Soc. (2) 36(2013) no. 4,1109–1122.Google Scholar
[10] Ghaani Farashahi, A., Abstract harmonic analysis of relative convolutions over canonical homogeneous spaces of semidirect product groups. J. Aust. Math. Soc. 101(2016), no. 2,171–187, http://dx.doi.Org/10.1017/S1446788715000798 Google Scholar
[11] Ghaani Farashahi, A., Abstract harmonic analysis of wave-packet transforms over locally compact abelian groups. Banach J. Math. Anal. 11(2017), no. 1, 50–71.http://dx.doi.org/10.1215/17358787-3721281 Google Scholar
[12] Ghaani Farashahi, A., Abstract operator-valued Fourier transforms over homogeneous spaces of compact groups. Groups, Geometry, Dynamics, to appear. Google Scholar
[13] Ghaani Farashahi, A., Abstract convolution function algebras over homogeneous spaces of compact groups. Illinois J. Math., to appear.Google Scholar
[14] Ghaani Farashahi, A., Abstract Plancherel (trace) formulas over homogeneous spaces of compact groups. Can. Math. Bull, to appear. http://dx.doi.Org/10.4153/CMB-2O16-037-6 Google Scholar
[15] Hewitt, E. and Ross, K. A., Abstract harmonic analysis. Vol. 1-2,1963,1970.Google Scholar
[16] Kisil, V., Relative convolutions. I. Properties and applications. Adv. Math. 147(1999), no. 1, 35–73. http://dx.doi.Org/10.1006/aima.1 999.1 833 Google Scholar
[17] Kisil, V., Erlangen program at large: an overview. Trends Math., Birkhäuser/Springer, Basel, 2012, pp. 1–94. http://dx.doi.Org/10.1007/978-3-0348-0417-2_1 Google Scholar
[18] Kisil, V., Geometry of Mobius transformations. Elliptic, parabolic and hyperbolic actions of SL2(). Imperial College Press, London, 2012.http://dx.doi.org/=10.1142/p835 Google Scholar
[19] Kisil, V., Operator covariant transform and local principle. J. Phys. A 45(2012), no. 24, pp. 244022, 10.http://dx.doi.org/10.1088/1751-8113/45/24/244022 Google Scholar
[20] Kisil, V., Calculus of operators: covariant transform and relative convolutions. Banach J. Math. Anal. 8(2014), no. 2, 156–184.http://dx.doi.org/10.15352/bjma/1396640061 Google Scholar
[21] Murphy, G. J., C* -algebras and operator theory. Academic Press, Boston, MA, 1990.Google Scholar
[22] Reiter, H. and Stegeman, J. D., Classical harmonic analysis and locally compact groups. Second edition. London Mathematical Society Monographs, 22. Oxford University Press, New York, 2000.Google Scholar