Article contents
Characteristic Cycles in Hermitian Symmetric Spaces
Published online by Cambridge University Press: 20 November 2018
Abstract
We give explicit combinatorial expresssions for the characteristic cycles associated to certain canonical sheaves on Schubert varieties X in the classical Hermitian symmetric spaces: namely the intersection homology sheaves IHX and the constant sheaves ℂX. The three main cases of interest are the Hermitian symmetric spaces for groups of type An (the standard Grassmannian), Cn (the Lagrangian Grassmannian) and Dn. In particular we find that CC(IHX) is irreducible for all Schubert varieties X if and only if the associated Dynkin diagramis simply laced. The result for Schubert varieties in the standard Grassmannian had been established earlier by Bressler, Finkelberg and Lunts, while the computations in the Cn and Dn cases are new.
Our approach is to compute CC(ℂX) by a direct geometric method, then to use the combinatorics of the Kazhdan-Lusztig polynomials (simplified for Hermitian symmetric spaces) to compute CC(IHX). The geometric method is based on the fundamental formula 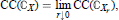 where the Xr ↓ X constitute a family of tubes around the variety X. This formula leads at once to an expression for the coefficients of CC(ℂX) as the degrees of certain singular maps between spheres.
where the Xr ↓ X constitute a family of tubes around the variety X. This formula leads at once to an expression for the coefficients of CC(ℂX) as the degrees of certain singular maps between spheres.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1997
References
- 9
- Cited by


