Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-30T19:47:05.522Z Has data issue: false hasContentIssue false
  • English
  • Français

Chains of Varieties

Published online by Cambridge University Press:  20 November 2018

Narain Gupta ,
Frank Levin  and
Akbar Rhemtulla
Narain Gupta
Affiliation:
University of Manitoba, Winnipeg, Manitoba
Frank Levin
Affiliation:
Rutgers, The State University, New Brunswick, New Jersey
Akbar Rhemtulla
Affiliation:
University of Alberta, Edmonton, Alberta
Rights & Permissions [Opens in a new window]

Summary

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

If is a variety of groups that can be denned by n-variable laws and (m) is the variety all of whose m-generator groups are in then there corresponds the chain: (1) ≧ (2) ≧ . . . ≧ (n) = . In this paper such chains are investigated to determine which of the inclusions are proper for certain varieties . In particular the inclusions are shown to be all proper for the varieties 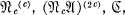where 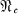is the variety of nilpotent-of-class-c groups, 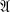is the abelian variety and 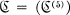is the variety of centre-bymetabelian groups. For 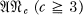the inclusions are likewise proper but for 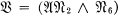the corresponding chain is: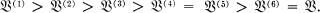

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 26 , Issue 1 , February 1974 , pp. 190 - 206
Copyright
Copyright © Canadian Mathematical Society 1974

References

1. Gruenberg, K. W., Residual properties of infinite soluble groups, Proc. London Math. Soc. 7 (1957), 29–62.Google Scholar
2. Gupta, C. K., On 2-metabelian groups, Arch. Math. 19 (1968), 584–587.Google Scholar
3. Gupta, C. K., Gupta, N. D., and Newman, M. F., Some finite nilpotent p-groups, J. Austral. Math. Soc. 9 (1969), 287–288.Google Scholar
4. Gupta, N. D., Certain locally metanilpotent varieties of groups, Arch. Math. 22 (1969), 481–484.Google Scholar
5. Heineken, H., Ueber ein Levisches Nilpotenzkriterium, Arch. Math. 12 (1961), 176–178.Google Scholar
6. Kappe, W., Die A-Norm einer Gruppe, Illinois J. Math. 5 (1961), 187–197.Google Scholar
7. Levi, F. W., Groups in which the commutator operation satisfies certain algebraic conditions, J. Indian Math. Soc. (N.S.) 6 (1942), 87–97.Google Scholar
8. Levi, F. W. and Van, B. L. der Waerden, Ueber eine besondere Klasse von Gruppen, Abh. Math. Sem. Univ. Hamburg 9 (1932), 154–158.Google Scholar
9. Levin, Frank, On some varieties of soluble groups, I. Math. Z. 85 (1964), 369–372.Google Scholar
10. Macdonald, I. D., On certain varieties of groups, Math. Z. 76 (1961), 270–282.Google Scholar
11. Macdonald, I. D., On certain varieties of groups, II. Math. Z. 78 (1962), 175–188.Google Scholar
12. Macdonald, I. D. and Neumann, B. H., A Third-Engel h-group, J. Austral. Math. Soc. 7 (1967), 555–569.Google Scholar
13. Magnus, W., Karass, A., and Solitar, D., Combinatorial group theory (Interscience, New York-London, 1966).Google Scholar
14. Neumann, B. H., On a conjecture of Hanna Neumann, Glasgow Math. J. 8 (1957), 13–17.Google Scholar
15. Neumann, Hanna, Varieties of groups (Springer-Verlag, New York, 1967).Google Scholar