No CrossRef data available.
Article contents
Bounds on Betti Numbers
Published online by Cambridge University Press: 20 November 2018
Extract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
The Betti numbers βn(k) of the residue class field k = R/m of a commutative local ring (R, m) have been studied for about 20 years, primarily as the coefficients of the Poincaré series of E . Several authors have obtained results about the growth of the sequence {βn(k)}.For example, Gulliksen [3] showed that when R is non-regular, the sequence is non-decreasing. More recently, Avramov [1] studied asymptotic properties of {βn(k)} and found that under certain conditions the growth is exponential, i.e., there is a natural number p such that for all n, βpn(k) ≧ 2n.
In this paper, we examine the sequence {βn(M)} for arbitrary finitely generated non-free modules M over any commutative local artin ring R. We establish the following bounds:
1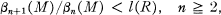
2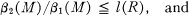
3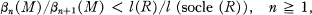
where l(X) is the length of X.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1982
References
1.
Avramov, L., Sur la croissance des nombres de Betti d'un anneau local,
C. R. Acad. Se. Paris t.
289 (1979), 369–372.Google Scholar
2.
Cartan, H. and Eilenberg, S., Homologuai algebra (Princeton Univ. Press, Princeton, N.J., 1956.Google Scholar
3.
Gulliksen, T., A proof of the existence of minimal R- Igebra resolutions,
Acta Math.
120 (1968), 53–58.Google Scholar
4.
Ramras, M., Betti numbers and reflexive modules, in Ring theory (Academic Press, 1972.Google Scholar
6.
Ramras, M. and Gover, E., Increasing sequences of Betti numbers,
Pacific J. Math.
87 (1980), 65–68.Google Scholar
You have
Access