Article contents
Boundedness of Differential Transforms for Heat Semigroups Generated by Schrödinger Operators
Published online by Cambridge University Press: 12 February 2020
Abstract
In this paper we analyze the convergence of the following type of series  $$\begin{eqnarray}T_{N}^{{\mathcal{L}}}f(x)=\mathop{\sum }_{j=N_{1}}^{N_{2}}v_{j}\big(e^{-a_{j+1}{\mathcal{L}}}f(x)-e^{-a_{j}{\mathcal{L}}}f(x)\big),\quad x\in \mathbb{R}^{n},\end{eqnarray}$$
$$\begin{eqnarray}T_{N}^{{\mathcal{L}}}f(x)=\mathop{\sum }_{j=N_{1}}^{N_{2}}v_{j}\big(e^{-a_{j+1}{\mathcal{L}}}f(x)-e^{-a_{j}{\mathcal{L}}}f(x)\big),\quad x\in \mathbb{R}^{n},\end{eqnarray}$$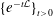 ${\{e^{-t{\mathcal{L}}}\}}_{t>0}$ is the heat semigroup of the operator
${\{e^{-t{\mathcal{L}}}\}}_{t>0}$ is the heat semigroup of the operator 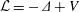 ${\mathcal{L}}=-\unicode[STIX]{x1D6E5}+V$ with
${\mathcal{L}}=-\unicode[STIX]{x1D6E5}+V$ with  $\unicode[STIX]{x1D6E5}$ being the classical laplacian, the nonnegative potential
$\unicode[STIX]{x1D6E5}$ being the classical laplacian, the nonnegative potential 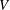 $V$ belonging to the reverse Hölder class
$V$ belonging to the reverse Hölder class 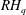 $RH_{q}$ with
$RH_{q}$ with 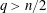 $q>n/2$ and
$q>n/2$ and 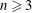 $n\geqslant 3$,
$n\geqslant 3$, 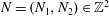 $N=(N_{1},N_{2})\in \mathbb{Z}^{2}$ with
$N=(N_{1},N_{2})\in \mathbb{Z}^{2}$ with  $N_{1}<N_{2}$,
$N_{1}<N_{2}$, 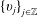 ${\{v_{j}\}}_{j\in \mathbb{Z}}$ is a bounded real sequences, and
${\{v_{j}\}}_{j\in \mathbb{Z}}$ is a bounded real sequences, and 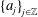 ${\{a_{j}\}}_{j\in \mathbb{Z}}$ is an increasing real sequence.
${\{a_{j}\}}_{j\in \mathbb{Z}}$ is an increasing real sequence.
Our analysis will consist in the boundedness, in  $L^{p}(\mathbb{R}^{n})$ and in
$L^{p}(\mathbb{R}^{n})$ and in 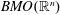 $BMO(\mathbb{R}^{n})$, of the operators
$BMO(\mathbb{R}^{n})$, of the operators 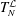 $T_{N}^{{\mathcal{L}}}$ and its maximal operator
$T_{N}^{{\mathcal{L}}}$ and its maximal operator 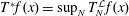 $T^{\ast }f(x)=\sup _{N}T_{N}^{{\mathcal{L}}}f(x)$.
$T^{\ast }f(x)=\sup _{N}T_{N}^{{\mathcal{L}}}f(x)$.
It is also shown that the local size of the maximal differential transform operators (with 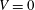 $V=0$) is the same with the order of a singular integral for functions
$V=0$) is the same with the order of a singular integral for functions  $f$ having local support. Moreover, if
$f$ having local support. Moreover, if 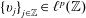 ${\{v_{j}\}}_{j\in \mathbb{Z}}\in \ell ^{p}(\mathbb{Z})$, we get an intermediate size between the local size of singular integrals and Hardy–Littlewood maximal operator.
${\{v_{j}\}}_{j\in \mathbb{Z}}\in \ell ^{p}(\mathbb{Z})$, we get an intermediate size between the local size of singular integrals and Hardy–Littlewood maximal operator.
MSC classification
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2020
Footnotes
The first author was supported by the National Natural Science Foundation of China (Grant Nos. 11971431, 11401525), the Natural Science Foundation of Zhejiang Province (Grant No. LY18A010006), the first Class Discipline of Zhejiang-A (Zhejiang Gongshang University-Statistics) and the State Scholarship Fund (No. 201808330097). The second author was supported by grant PGC2018-099124-B-I00 (MINECO/FEDER) from Government of Spain.
References
- 14
- Cited by



