Article contents
A Basically Disconnected Normal Space Φ With |βΦ – Φ| = 1
Published online by Cambridge University Press: 20 November 2018
Extract
All spaces considered are completely regular. C*(X) denotes the set of bounded continuous real-valued functions in X. A subspace S of X is called C*-embedded in X if for every f ∈ C*(S) there is ϕ ∈ C*(X) with ϕ⥤S = f.
A space X is called almost compact if |βX – X| ≦ 1; basically disconnected if every cozero-set has open closure; extremally disconnected if every open set has open closure; an F-space if every cozero-set is C*-embedded; small if |C*(X)| = 2ω; and weakly Lindelöf if every open cover has a subfamily 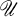 with
with 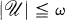 and ⋃
and ⋃  dense. A point p of a space X is called a P-point of X if every Gδ-set in X which contains p is a neighborhood of p.
dense. A point p of a space X is called a P-point of X if every Gδ-set in X which contains p is a neighborhood of p.
ω(X) denotes the weight of X.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1979
References
- 8
- Cited by


