Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-25T06:10:58.109Z Has data issue: false hasContentIssue false
  • English
  • Français

Asymptotic Continuous Orbit Equivalence of Smale Spaces and Ruelle Algebras

Part of: Dynamical systems with hyperbolic behavior Selfadjoint operator algebras

Published online by Cambridge University Press:  09 January 2019

Kengo Matsumoto
Kengo Matsumoto*
Affiliation:
Department of Mathematics, Joetsu University of Education, Joetsu 943-8512, Japan Email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

In the first part of the paper, we introduce notions of asymptotic continuous orbit equivalence and asymptotic conjugacy in Smale spaces and characterize them in terms of their asymptotic Ruelle algebras with their dual actions. In the second part, we introduce a groupoid 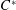$C^{\ast }$-algebra that is an extended version of the asymptotic Ruelle algebra from a Smale space and study the extended Ruelle algebras from the view points of Cuntz–Krieger algebras. As a result, the asymptotic Ruelle algebra is realized as a fixed point algebra of the extended Ruelle algebra under certain circle action.

Keywords

hyperbolic dynamicsSmale spaceRuelle algebragroupoidzeta functioncontinuous orbit equivalenceshifts of finite typeCuntz-Krieger algebra

MSC classification

Primary: 37D20: Uniformly hyperbolic systems (expanding, Anosov, Axiom A, etc.)
Secondary: 46L35: Classifications of $C^*$-algebras
Type
Article
Information
Canadian Journal of Mathematics , Volume 71 , Issue 5 , October 2019 , pp. 1243 - 1296
Copyright
© Canadian Mathematical Society 2018 

Footnotes

This work was supported by JSPS KAKENHI Grant Number 15K04896.

References

Anantharaman-Delaroche, C. and Renault, J., Amenable groupoids . Monographies de L’Enseignement Mathématique, 36, L’Enseignement Mathématique, Genéve, 2000.Google Scholar
Bowen, R., Equilibrium states and the ergodic theory of Anosov diffeomorphisms . Lecture Notes in Mathematics, 470, Springer, Berlin, 1975.Google Scholar
Bowen, R., On Axiom A diffeomorphisms . Regional Conference Series in Mathematics, 35, American Mathematical Society, Providence, RI, 1978.Google Scholar
Bratteli, O., Kishimoto, A., Rørdam, M., and Størmer, E., The crossed product of a UHF algebra by a shift . Ergodic Theory Dynam. Systems 13(1993), 615–626. https://doi.org/10.1017/S0143385700007574.Google Scholar
Carlsen, T. M. and Rout, J., Diagonal-preserving gauge invariant isomorphisms of graph C ∗-algebras . J. Funct. Anal. 273(2017), 2981–2993. https://doi.org/10.1016/j.jfa.2017.06.018.Google Scholar
Carlsen, T. M., Ruiz, E., and Sims, A., Equivalence and stable isomorphism of groupoids, and diagonal-preserving stable isomorphisms of graph C ∗-algebras and Leavitt path algebras . Proc. Amer. Math. Soc. 145(2017), 1581–1592.Google Scholar
Cuntz, J. and Krieger, W., A class of C ∗-algebras and topological Markov chains . Invent. Math. 56(1980), 251–268. https://doi.org/10.1007/BF01390048.Google Scholar
Holton, C. G., The Rohlin property for shifts of finite type . J. Funct. Anal. 229(2005), 277–299. https://doi.org/10.1016/j.jfa.2005.05.002.Google Scholar
Kaminker, J. and Putnam, I. F., K-theoretic duality for shifts of finite type . Comm. Math. Phys. 187(1997), 509–522. https://doi.org/10.1007/s002200050147.Google Scholar
Kaminker, J., Putnam, I. F., and Spielberg, J., Operator algebras and hyperbolic dynamics. Operator algebras and quantum field theory (Rome, 1996), Int. Press, Cambridge, MA, 1997, pp. 525–532.Google Scholar
Killough, D. B. and Putnam, I. F., Ring and module structures on dimension groups associated with a shift of finite type . Ergodic Theory Dynam. Systems 32(2012), 1370–1399. https://doi.org/10.1017/S0143385712000272.Google Scholar
Kishimoto, A., The Rohlin property for automorphisms of UHF algebras . J. Reine Angew. Math. 465(1995), 183–196. https://doi.org/10.1515/crll.1995.465.183.Google Scholar
Lind, D. and Marcus, B., An introduction to symbolic dynamics and coding . Cambridge University Press, Cambridge, 1995. https://doi.org/10.1017/CBO9780511626302.Google Scholar
Matsumoto, K., Orbit equivalence of topological Markov shifts and Cuntz–Krieger algebras . Pacific J. Math. 246(2010), 199–225. https://doi.org/10.2140/pjm.2010.246.199.Google Scholar
Matsumoto, K., Strongly continuous orbit equivalence of one-sided topological Markov shifts . J. Operator Theory 74(2015), 457–483. https://doi.org/10.7900/jot.2014aug19.2063.Google Scholar
Matsumoto, K., Uniformly continuous orbit equivalence of Markov shifts and gauge actions on Cuntz–Krieger algebras . Proc. Amer. Math. Soc. 145(2017), 1131–1140. https://doi.org/10.1090/proc/13387.Google Scholar
Matsumoto, K., Continuous orbit equivalence, flow equivalence of Markov shifts and circle actions on Cuntz–Krieger algebras . Math. Z. 285(2017), 121–141. https://doi.org/10.1007/s00209-016-1700-3.Google Scholar
Matsumoto, K., Topological conjugacy of topological Markov shifts and Ruelle algebras. 2018. arxiv:1706.07155.Google Scholar
Matsumoto, K. and Matui, H., Continuous orbit equivalence of topological Markov shifts and Cuntz–Krieger algebras . Kyoto J. Math. 54(2014), 863–878. https://doi.org/10.1215/21562261-2801849.Google Scholar
Matsumoto, K. and Matui, H., Continuous orbit equivalence of topological Markov shifts and dynamical zeta functions . Ergodic Theory Dynam. Systems 36(2016), 1557–1581. https://doi.org/10.1017/etds.2014.128.Google Scholar
Matui, H., Homology and topological full groups of étale groupoids on totally disconnected spaces . Proc. Lond. Math. Soc. 104(2012), 27–56. https://doi.org/10.1112/plms/pdr029.Google Scholar
Matui, H., Topological full groups of one-sided shifts of finite type . J. Reine Angew. Math. 705(2015), 35–84. https://doi.org/10.1515/crelle-2013-0041.Google Scholar
Muhly, P. S., Renault, J., and Williams, D. P., Equivalence and isomorphism for groupoid C ∗-algebras . J. Operator Theory 17(1987), 3–22.Google Scholar
Parry, W. and Pollicott, M., Zeta functions and the periodic orbit structure of hyperbolic dynamics . Astérisque 187–188(1990).Google Scholar
Putnam, I. F., C ∗-algebras from Smale spaces . Canad. J. Math. 48(1996), 175–195. https://doi.org/10.4153/CJM-1996-008-2.Google Scholar
Putnam, I. F., Hyperbolic systems and generalized Cuntz–Krieger algebras. Lecture Notes, Summer School in Operator Algebras, Odense, August 1996, Odense University, Odense, Denmark.Google Scholar
Putnam, I. F., Functoriality of the C ∗-algebras associated with hyperbolic dynamical systems . J. London Math. Soc. 62(2000), 873–884. https://doi.org/10.1112/S002461070000140X.Google Scholar
Putnam, I. F., A homology theory for Smale spaces . Mem. Amer. Math. Soc. 232(2014), no. 1094. https://doi.org/10.1090/memo/1094.Google Scholar
Putnam, I. F. and Spielberg, J., The structure of C ∗-algebras associated with hyperbolic dynamical systems . J. Funct. Anal. 163(1999), 279–299. https://doi.org/10.1006/jfan.1998.3379.Google Scholar
Renault, J., A groupoid approach to C ∗-algebras . Lecture Notes in Mathematics, 793, Springer, Berlin, 1980.Google Scholar
Renault, J., Cartan subalgebras in C ∗-algebras . Irish Math. Soc. Bull. 61(2008), 29–63.Google Scholar
Renault, J., Examples of masas in C ∗-algebras. In: Operator structures and dynamical systems, Contemp. Math., 503, American Mathematical Socisty, Providence, RI, 2009. https://doi.org/10.1090/conm/503/09903.Google Scholar
Ruelle, D., Thermodynamic formalism. The mathematical structures of classical equilibrium statistical mechanics . Encyclopedia of Mathematics and its Applications, 5, Addison-Wesley, Reading, Mass, 1978.Google Scholar
Ruelle, D., Non-commutative algebras for hyperbolic diffeomorphisms . Invent. Math. 93(1988), 1–13. https://doi.org/10.1007/BF01393685.Google Scholar
Ruelle, D., Dynamical zeta functions and transfer operators . Notices Amer. Math. Soc. 49(2002), 887–895.Google Scholar
Smale, S., Differentiable dynamical systems . Bull. Amer. Math. Soc. 73(1967), 747–817. https://doi.org/10.1090/S0002-9904-1967-11798-1.Google Scholar
Thomsen, K., C ∗-algebras of homoclinic and heteroclinic structure in expansive dynamics . Mem. Amer. Math. Soc. 206(2010), no. 970. https://doi.org/10.1090/S0065-9266-10-00581-8.Google Scholar
Williams, R. F., Classification of subshifts of finite type . Ann. of Math. 98(1973), 120–153; errata: Ann. Math. 99(1974), 380–381. https://doi.org/10.2307/1970908.Google Scholar