Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-24T08:06:29.890Z Has data issue: false hasContentIssue false
  • English
  • Français

Application of the Strong Artin Conjecture to the Class Number Problem

Published online by Cambridge University Press:  20 November 2018

Peter J. Cho  and
Henry H. Kim
Peter J. Cho
Affiliation:
Department of Mathematics, University of Toronto, TorontoON M5S 2E4, e-mail: [email protected]
Henry H. Kim
Affiliation:
Department of Mathematics, University of Toronto, TorontoON M5S 2E4 andKorea Institute for Advanced Study, Seoul, Korea, e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We construct unconditionally several families of number fields with the largest possible class numbers. They are number fields of degree 4 and 5 whose Galois closures have the Galois group 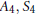${{A}_{4}},\,{{S}_{4}}$, and 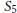${{S}_{5}}$. We first construct families of number fields with smallest regulators, and by using the strong Artin conjecture and applying the zero density result of Kowalski–Michel, we choose subfamilies of $L$-functions that are zero-free close to 1. For these subfamilies, the $L$-functions have the extremal value at 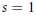$s\,=\,1$, and by the class number formula, we obtain the extreme class numbers.

Keywords

11R2911M41class numberstrong Artin conjecture
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 65 , Issue 6 , 01 December 2013 , pp. 1201 - 1216
Copyright
Copyright © Canadian Mathematical Society 2013

References

[1] Ankeny, N. C., Brauer, R., and Chowla, S., A note on the class-numbers of algebraic number fields. Amer. J. Math. 78(1956), 51–61. http://dx.doi.org/10.2307/2372483 Google Scholar
[2] Cho, P. J., The strong Artin conjecture and number fields with large class numbers. Q. J. Math., to appear.Google Scholar
[3] Cho, P. J. and H. H. Kim, Dihedral and cyclic extensions with large class numbers. J. Th. Nombres Bordeaux, to appear.Google Scholar
[4] F. Calegari, The Artin Conjecture for some S5-extensions. arxiv:1112.1152v1 Google Scholar
[5] Cohen, H., A course in computational algebraic number theory. Graduate Texts in Mathematics, 138, Springer-Verlag, Berlin, 1993.Google Scholar
[6] Duke, W., Extreme values of Artin L-functions and class numbers. Compositio Math. 136(2003), no. 1, 103–115. http://dx.doi.org/10.1023/A:1022695505997 Google Scholar
[7] Daileda, R. C., Non-abelian number fields with very large class numbers. Acta Arith. 125(2006), no. 3, 215–255. http://dx.doi.org/10.4064/aa125-3-2 Google Scholar
[8] Hooley, C., Applications of sieve methods to the theory of numbers. Cambridge Tracts in Mathematics, 70, Cambridge University Press, Cambridge, 1976.Google Scholar
[9] Heath-Brown, D. R., Powerfree values of polynomials. arxiv:1103.2028. Google Scholar
[10] Khare, C. and P.Wintenberger, J., Serre's modularity conjecture. I. Invent. Math. 178(2009), no. 3, 485–504. http://dx.doi.org/10.1007/s00222-009-0205-7 Google Scholar
[11] Kim, H. H., An example of non-normal quintic automorphic induction and modularity of symmetric powers of cusp forms of icosahedral type. Invent. Math. 156(2004), no. 3, 495–502. http://dx.doi.org/10.1007/s00222-003-0340-5 Google Scholar
[12] Klingen, N., Arithmetical similarities. Oxford Mathematical Monographs, Oxford Science Publications, The Clarendon Press, Oxford University Press, New York, 1998.Google Scholar
[13] Kowalski, E. and Michel, P., Zeros of families of automorphic L-functions close to 1. Pacific J. Math. 207(2002), no. 2, 411–431. http://dx.doi.org/10.2140/pjm.2002.207.411 Google Scholar
[14] Montgomery, H. L. and Weinberger, P. J., Real quadratic fields with large class numbers. Math. Ann. 225(1977), no. 2, 173–176. http://dx.doi.org/10.1007/BF01351721 Google Scholar
[15] Nagell, T., Introduction to number theory. Second ed., Chelsea Publishing Co., New York, 1964.Google Scholar
[16] Narkiewicz, W., Elementary and analytic theory of algebraic numbers. Second ed., Springer-Verlag, Berlin, PWN-Polish Scientific Publisher,Warsaw, 1990.Google Scholar
[17] Obreschkoff, N., Verteilung und Berechnung der Nullstellen reeller Polynome. Deutscher Verlag der Wissenschaften, Berlin, 1963.Google Scholar
[18] Ramakrishnan, D., Modularity of the Rankin-Selberg L-series, and multiplicity one for SL(2). Ann. of Math. 152(2000), no. 1, 45–111. http://dx.doi.org/10.2307/2661379 Google Scholar
[19] Sasaki, S., On Artin representations and nearly ordinary Hecke algebras over totally real fields. I. http://www.cantabgold.net/users/s.sasaki.03/Google Scholar
[20] Sándor, J., MitrinoviĆ, D., and Crstici, B., Handbook of number theory. I. Springer, Dordrecht, 2006.Google Scholar
[21] Schäpp, A. M., Fundamental units in a parametric family of not totally real quintic number fields. J. Théor. Nombres Bordeaux 18(2006), no. 3, 693–706. http://dx.doi.org/10.5802/jtnb.567 Google Scholar
[22] Serre, J.-P., Topics in Galois theory, Research Notes in Mathematics, 1, A K Peters, Ltd.,Wellesley, MA, 2008.Google Scholar
[23] Silverman, J. H., An inequality relating the regulator and the discriminant of a number field. J. Number Theory 19(1984), no. 3, 437–442. http://dx.doi.org/10.1016/0022-314X(84)90082-9 Google Scholar
[24] Zhang, Y., L-functions in number theory. Ph.D. Thesis, University of Toronto, 2009, ProQuest LLC, Ann Arbor, MI, 2010.Google Scholar