Article contents
Actions That Fiber and Vector Semigroups
Published online by Cambridge University Press: 20 November 2018
Extract
From [2], we can derive a criterion for determining when an action of a Lie group on a locally compact space leads to a fiber bundle. Here, we present an equivalent criterion which can be stated purely in the language of actions of groups on spaces. This is Theorem I. Using this result, we are able to give a version of a result of Home [1] for dimensions greater than one. This is done in Theorem IV and Corollary IVA. In Theorem II, we show that if a vector semigroup 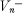 acts on a space X, then whenever the map t ↦ tx is 1 — 1 from
acts on a space X, then whenever the map t ↦ tx is 1 — 1 from 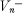 onto
onto 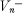 x, it is in fact a homeomorphism. Also,
x, it is in fact a homeomorphism. Also, 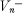 is a closed subset of X. This is also a version of a result in [1].
is a closed subset of X. This is also a version of a result in [1].
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1972
References
- 2
- Cited by


