Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-28T06:37:03.098Z Has data issue: false hasContentIssue false
  • English
  • Français

On the structure of Kac–Moody algebras

Part of: Lie algebras and Lie superalgebras

Published online by Cambridge University Press:  20 May 2020

Timothée Marquis
Timothée Marquis*
Affiliation:
Université catholique de Louvain, IRMP, Chemin du Cyclotron 2, bte L7.01.02, 1348 Louvain-la-Neuve, Belgique
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

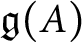Let A be a symmetrisable generalised Cartan matrix, and let $\mathfrak {g}(A)$ be the corresponding Kac–Moody algebra. In this paper, we address the following fundamental question on the structure of $\mathfrak {g}(A)$ : given two homogeneous elements 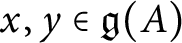$x,y\in \mathfrak {g}(A)$ , when is their bracket $[x,y]$ a nonzero element? As an application of our results, we give a description of the solvable and nilpotent graded subalgebras of $\mathfrak {g}(A)$ .

Keywords

Kac-Moody algebrassolvable subalgebrasnilpotent subalgebras

MSC classification

Primary: 17B67: Kac-Moody (super)algebras; extended affine Lie algebras; toroidal Lie algebras
Secondary: 17B30: Solvable, nilpotent (super)algebras
Type
Article
Information
Canadian Journal of Mathematics , Volume 73 , Issue 4 , August 2021 , pp. 1124 - 1152
Check for updates
Copyright
© Canadian Mathematical Society 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

F.R.S.-FNRS post-doctoral researcher.

References

Berman, S. and Moody, R. V., Lie algebra multiplicities. Proc. Amer. Math. Soc. 76(1979), no. 2, 223–228.CrossRefGoogle Scholar
Billig, Y. and Pianzola, A., Root strings with two consecutive real roots. Tohoku Math. J. 47(1995), no. 3, 391–403.CrossRefGoogle Scholar
Caprace, P.-E., A uniform bound on the nilpotency degree of certain subalgebras of Kac-Moody algebras. J. Algebra 317(2007), no. 2, 867–876.CrossRefGoogle Scholar
Caprace, P.-E., Non-discrete simple locally compact groups. In: European Congress of Mathematics, Eur. Math. Soc., Zürich, 2018, pp. 333–354.CrossRefGoogle Scholar
Caprace, P.-E. and Marquis, T., Closed sets of real roots in Kac–Moody root systems. Preprint, 2018. arxiv.org/abs/1810.05114.Google Scholar
Carbone, L., Freyn, W., and Lee, K.-H., Dimensions of imaginary root spaces of hyperbolic Kac-Moody algebras. In: Recent advances in representation theory, quantum groups, algebraic geometry, and related topics, Contemporary Mathematics, 623, Amer. Math. Soc., Providence, RI, 2014, pp. 23–40.Google Scholar
Damour, T., Henneaux, M., and Nicolai, H., E 10 and a small tension expansion of M theory. Phys. Rev. Lett. 89(2002), no. 22, 221601.CrossRefGoogle Scholar
Damour, T. and Nicolai, H., Higher-order M-theory corrections and the Kac-Moody algebra E 10. Classic. Quant. Gravity 22(2005), no. 14, 2849–2879.CrossRefGoogle Scholar
Feingold, A. J. and Frenkel, I. B., A hyperbolic Kac-Moody algebra and the theory of Siegel modular forms of genus 2. Math. Ann. 263(1983), no. 1, 87–144.CrossRefGoogle Scholar
Fleig, P., Gustafsson, H. P. A., Kleinschmidt, A., and Persson, D., Eisenstein series and automorphic representations. Cambridge Studies in Advanced Mathematics, 176, Cambridge University Press, Cambridge, UK, 2018.CrossRefGoogle Scholar
Gaberdiel, M. R., Olive, D. I., and West, P. C., A class of Lorentzian Kac-Moody algebras. Nuclear Phys. B. 645(2002), no. 3, 403–437.CrossRefGoogle Scholar
Kac, V. G., Simple graded Lie algebras of finite height. Funkcional Anal. i Priložen 1(1967), no. 4, 82–83.Google Scholar
Kac, V. G., Infinite-dimensional Lie algebras. 3rd ed., Cambridge University Press, Cambridge, UK, 1990.CrossRefGoogle Scholar
Kac, V. G. and Peterson, D. H., On geometric invariant theory for infinite-dimensional groups . Algebraic Groups Utrecht 1986, Lecture Notes in Mathematics, 1271, Springer, Berlin, 1987, pp. 109–142.CrossRefGoogle Scholar
Kang, S.-J., Root multiplicities of Kac-Moody algebras . Duke Math. J. 74(1994), no. 3, 635–666.CrossRefGoogle Scholar
Kang, S.-J. and Melville, D. J., Rank $\;2\;$ symmetric hyperbolic Kac-Moody algebras . Nagoya Math. J. 140(1995), 41–75.CrossRefGoogle Scholar
Kumar, S., Kac–Moody groups, their flag varieties and representation theory . Progress in Mathematics, 204, Birkhäuser Boston Inc., Boston, MA, 2002.Google Scholar
Marquis, T., An introduction to Kac–Moody groups over fields . EMS Textbooks in Mathematics, Eur. Math. Soc., Zürich, 2018.Google Scholar
Moody, R. V., Lie algebras associated with generalized Cartan matrices . Bull. Amer. Math. Soc. 73(1967), 217–221.CrossRefGoogle Scholar
Moody, R. V. and Pianzola, A., Lie algebras with triangular decompositions . Canadian Mathematical Society Series of Monographs and Advanced Texts, John Wiley & Sons Inc., New York, NY, 1995.Google Scholar
Serre, J.-P., Algèbres de Lie semi-simples complexes. W. A. Benjamin Inc. New York, NY–Amsterdam, Netherlands, 1966.Google Scholar
West, P., E 11 and M theory . Classic. Quant. Gravity 18(2001), no. 21, 4443–4460.CrossRefGoogle Scholar