Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-27T23:36:37.719Z Has data issue: false hasContentIssue false
  • English
  • Français

UNIMODALITY AND COLOURED HOOK FACTORISATION

Part of: Combinatorics

Published online by Cambridge University Press:  11 November 2015

ZHICONG LIN
ZHICONG LIN*
Affiliation:
School of Science, Jimei University, Xiamen 361021, PR China CAMP, National Institute for Mathematical Sciences, Daejeon 305-811, Republic of Korea email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We prove the unimodality of some coloured 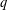$q$-Eulerian polynomials, which involve the flag excedances, the major index and the fixed points on coloured permutation groups, via two recurrence formulas. In particular, we confirm a recent conjecture of Mongelli about the unimodality of the flag excedances over type B derangements. Furthermore, we find the coloured version of Gessel’s hook factorisation, which enables us to interpret these two recurrences combinatorially. We also provide a combinatorial proof of a symmetric and unimodal expansion for the coloured derangement polynomial, which was first established by Shin and Zeng using continued fractions.

Keywords

coloured permutationsderangementsEulerian polynomialsunimodality𝛾-positivityhook factorisation

MSC classification

Primary: 05A05: Permutations, words, matrices
Secondary: 05A15: Exact enumeration problems, generating functions 05A19: Combinatorial identities, bijective combinatorics
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 93 , Issue 1 , February 2016 , pp. 1 - 12
Copyright
© 2015 Australian Mathematical Publishing Association Inc. 

References

Bagno, E. and Garber, D., ‘On the excedance numbers of coloured permutation groups’, Sém. Lothar. Combin. 53 (2006), Art. B53f, 17 pp.Google Scholar
Chow, C.-O. and Mansour, T., ‘Counting derangements, involutions and unimodal elements in the wreath product C r ≀§n’, Israel J. Math. 179 (2010), 425–448.CrossRefGoogle Scholar
Faliharimalala, H. L. M. and Zeng, J., ‘Fix–Euler–Mahonian statistics on wreath products’, Adv. Appl. Math. 46 (2011), 275–295.CrossRefGoogle Scholar
Foata, D., ‘Eulerian polynomials: from Euler’s time to the present’, in: The Legacy of Alladi Ramakrishnan in the Mathematical Sciences (eds. Alladi, K., Klauder, J. R. and Rao, C. R.) (Springer, New York, 2010), 253–273.CrossRefGoogle Scholar
Foata, D. and Han, G.-N., ‘Fix-Mahonian calculus III: a quadruple distribution’, Monatsh. Math. 154 (2008), 177–197.CrossRefGoogle Scholar
Foata, D. and Han, G.-N., ‘The q-tangent and q-secant numbers via basic Eulerian polynomials’, Proc. Amer. Math. Soc. 138 (2009), 385–393.CrossRefGoogle Scholar
Foata, D. and Han, G.-N., ‘The decrease value theorem with an application to permutation statistics’, Adv. Appl. Math. 46 (2011), 296–311.CrossRefGoogle Scholar
Gessel, I. M., ‘A colouring problem’, Amer. Math. Monthly 98 (1991), 530–533.CrossRefGoogle Scholar
Han, G.-N., Lin, Z. and Zeng, J., ‘A symmetrical q-Eulerian identity’, Sém. Lothar. Combin. 67 (2012), Art. B67c, 11 pp.Google Scholar
Hyatt, M., ‘Eulerian quasisymmetric functions for the type B Coxeter group and other wreath product groups’, Adv. Appl. Math. 48 (2012), 465–505.CrossRefGoogle Scholar
Lin, Z., ‘On some generalized q-Eulerian polynomials’, Electron. J. Combin. 20(1) (2013), Art. P55.CrossRefGoogle Scholar
Mongelli, P., ‘Excedances in classical and affine Weyl groups’, J. Combin. Theory Ser. A 120 (2013), 1216–1234.CrossRefGoogle Scholar
Shareshian, J. and Wachs, M. L., ‘Eulerian quasisymmetric functions’, Adv. Math. 225 (2011), 2921–2966.CrossRefGoogle Scholar
Shin, H. and Zeng, J., ‘The symmetric and unimodal expansion of Eulerian polynomials via continued fractions’, European J. Combin. 33 (2012), 111–127.CrossRefGoogle Scholar
Shin, H. and Zeng, J., ‘Symmetric unimodal expansions of excedances in colored permutations’, arXiv:1411.6184v2.Google Scholar
Stanley, R. P., ‘Unimodal and log-concave sequences in algebra, combinatorics, and geometry’, in: Graph Theory and its Applications: East and West, Annals of the New York Academy of Sciences, 576 (1989), 500–535.Google Scholar
Steingrímsson, E., ‘Permutation statistics of indexed permutations’, European J. Combin. 15 (1994), 187–205.CrossRefGoogle Scholar
Sun, H. and Wang, Y., ‘A group action on derangements’, Electron. J. Combin. 21(1) (2014), Art. P1.67.CrossRefGoogle Scholar
Wachs, M. L., ‘On the (co)homology of the partition lattice and the free Lie algebra’, Discrete Math. 193 (1998), 287–319.CrossRefGoogle Scholar
Zhang, X., ‘On q-derangement polynomials’, in: Combinatorics and Graph Theory ’95, Vol. 1 (Hefei) (World Scientific, River Edge, NJ, 1995), 462–465.Google Scholar