Hostname: page-component-78c5997874-8bhkd Total loading time: 0 Render date: 2024-11-14T01:12:45.630Z Has data issue: false hasContentIssue false
  • English
  • Français

TWO-SIDED ESTIMATES FOR POSITIVE SOLUTIONS OF SUPERLINEAR ELLIPTIC BOUNDARY VALUE PROBLEMS

Part of: Elliptic equations and systems Partial differential equations

Published online by Cambridge University Press:  30 August 2018

KENTARO HIRATA
KENTARO HIRATA*
Affiliation:
Department of Mathematics, Graduate School of Science, Hiroshima University, Higashi-Hiroshima 739-8526, Japan email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We give two-sided estimates for positive solutions of the superlinear elliptic problem $-\unicode[STIX]{x1D6E5}u=a(x)|u|^{p-1}u$ with zero Dirichlet boundary condition in a bounded Lipschitz domain. Our result improves the well-known a priori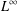$L^{\infty }$-estimate and provides information about the boundary decay rate of solutions.

Keywords

a priori estimatesemilinear elliptic equation

MSC classification

Primary: 35B45: A priori estimates
Secondary: 35J91: Semilinear elliptic equations with Laplacian, bi-Laplacian or poly-Laplacian
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 98 , Issue 3 , December 2018 , pp. 465 - 473
Copyright
© 2018 Australian Mathematical Publishing Association Inc. 

Footnotes

This work was supported in part by JSPS KAKENHI Grant Number JP18K03333.

References

Armitage, D. H. and Gardiner, S. J., Classical Potential Theory (Springer, London, 2001).Google Scholar
Bidaut-Véron, M. F. and Vivier, L., ‘An elliptic semilinear equation with source term involving boundary measures: the subcritical case’, Rev. Mat. Iberoam. 16(3) (2000), 477–513.Google Scholar
Bogdan, K., ‘Sharp estimates for the Green function in Lipschitz domains’, J. Math. Anal. Appl. 243(2) (2000), 326–337.Google Scholar
Brézis, H. and Turner, R. E. L., ‘On a class of superlinear elliptic problems’, Comm. Partial Differential Equations 2(6) (1977), 601–614.Google Scholar
de Figueiredo, D. G., Lions, P.-L. and Nussbaum, R. D., ‘A priori estimates and existence of positive solutions of semilinear elliptic equations’, J. Math. Pures Appl. (9) 61(1) (1982), 41–63.Google Scholar
Gidas, B. and Spruck, J., ‘A priori bounds for positive solutions of nonlinear elliptic equations’, Comm. Partial Differential Equations 6(8) (1981), 883–901.Google Scholar
Gilbarg, D. and Trudinger, N. S., Elliptic Partial Differential Equations of Second Order (Springer, Berlin, 2001).Google Scholar
Hirata, K., ‘Global estimates for non-symmetric Green type functions with applications to the p-Laplace equation’, Potential Anal. 29(3) (2008), 221–239.Google Scholar
Hirata, K., ‘Existence and nonexistence of a positive solution of the Lane–Emden equation having a boundary singularity: the subcritical case’, Monatsh. Math. 186(4) (2018), 635–652.Google Scholar
Jerison, D. S. and Kenig, C. E., ‘Boundary behavior of harmonic functions in nontangentially accessible domains’, Adv. Math. 46(1) (1982), 80–147.Google Scholar
Maeda, F. Y. and Suzuki, N., ‘The integrability of superharmonic functions on Lipschitz domains’, Bull. Lond. Math. Soc. 21(3) (1989), 270–278.Google Scholar
McKenna, P. J. and Reichel, W., ‘A priori bounds for semilinear equations and a new class of critical exponents for Lipschitz domains’, J. Funct. Anal. 244(1) (2007), 220–246.Google Scholar
Quittner, P. and Souplet, P., Superlinear Parabolic Problems (Birkhäuser, Basel, 2007).Google Scholar