Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-26T12:18:49.240Z Has data issue: false hasContentIssue false
  • English
  • Français

SQUARES OF DEGREES OF BRAUER CHARACTERS AND MONOMIAL BRAUER CHARACTERS

Part of: Representation theory of groups

Published online by Cambridge University Press:  29 January 2019

XIAOYOU CHEN  and
MARK L. LEWIS Open the ORCID record for MARK L. LEWIS [Opens in a new window]
XIAOYOU CHEN
Affiliation:
College of Science, Henan University of Technology, Zhengzhou 450001, China email [email protected]
MARK L. LEWIS*
Affiliation:
Department of Mathematical Sciences, Kent State University, Kent, OH 44242, USA email [email protected]
*
✉[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

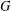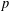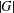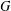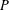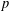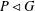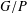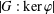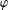Let $G$ be a finite group and let $p$ be a prime factor of $|G|$. Suppose that $G$ is solvable and $P$ is a Sylow $p$-subgroup of $G$. In this note, we prove that $P{\vartriangleleft}G$ and $G/P$ is nilpotent if and only if $\unicode[STIX]{x1D711}(1)^{2}$ divides $|G:\ker \unicode[STIX]{x1D711}|$ for all irreducible monomial $p$-Brauer characters $\unicode[STIX]{x1D711}$ of 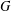$G$.

Keywords

solvable groupnilpotent groupmonomial Brauer character

MSC classification

Primary: 20C20: Modular representations and characters
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 100 , Issue 1 , August 2019 , pp. 58 - 60
Copyright
© 2019 Australian Mathematical Publishing Association Inc. 

Footnotes

The first author acknowledges the hospitality of the Department of Mathematical Sciences of Kent State University and the support of the China Scholarship Council, the Program for Young Key Teachers of Henan University of Technology, the Project of Foreign Experts Affairs of Henan Province, Funds of Henan University of Technology (2014JCYJ14, 26510009), Project of Department of Education of Henan Province (17A110004), Fund of Henan Province (162300410066) and the NSFC (11571129, 11771356, 11601121, 11701149).

References

Chen, X., Cossey, J. P., Lewis, M. L. and Tong-Viet, H. P., ‘Blocks of small defect in alternating groups and squares of Brauer character degrees’, J. Group Theory 20(6) (2017), 1155–1173.Google Scholar
Gagola, S. M. Jr. and Lewis, M. L., ‘A character theoretic condition characterizing nilpotent groups’, Comm. Algebra 27 (1999), 1053–1056.Google Scholar
Gallagher, P. X., ‘Group characters and normal Hall subgroups’, Nagoya Math. J. 21 (1962), 223–230.Google Scholar
Isaacs, I. M., ‘Large orbits in actions of nilpotent groups’, Proc. Amer. Math. Soc. 127 (1999), 45–50.Google Scholar
Lu, J., ‘On a theorem of Gagola and Lewis’, J. Algebra Appl. 16 1750158 (2017), 3 pages.Google Scholar
Navarro, G., Characters and Blocks of Finite Groups (Cambridge University Press, Cambridge, 1998).Google Scholar