Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-30T18:28:43.575Z Has data issue: false hasContentIssue false
  • English
  • Français

Slice convergence of parametrised sums of convex functions in non-reflexive spaces

Published online by Cambridge University Press:  17 April 2009

Robert Wenczel  and
Andrew Eberhard
Robert Wenczel
Affiliation:
Department of MathematicsRoyal Melbourne University of TechnologyMelbourne Vic 3001Australia e-mail: [email protected]
Andrew Eberhard
Affiliation:
Department of MathematicsRoyal Melbourne University of TechnologyMelbourne Vic 3001Australia e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The objectives of this study of slice convergence are two-fold. The first is to derive results regarding the passage of certain semi–convergences through Young–Fenchel conjugation. These semi–convergences arise from the splitting of the usual slice topology in the primal and dual spaces into (non-Hausdorff) topologies: the upper slice topology 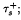; a topology generating a convergence closely resembling the bounded–weak* upper Kuratowski convergence; along with the respective primal and dual lower Kuratowski topologies. This gives rise to topological convergences not reliant on sequentially–based definitions found in many such studies, and associated topological continuity results for conjugation (in normed spaces), in contrast to the usual sequential continuity exhibited by analogues of Mosco convergence. The second objective is to study the passage of slice convergence through addition. Such sum theorems have been derived in other works and we establish previous theorems from a unified framework as well as obtaining a new result.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 60 , Issue 3 , December 1999 , pp. 429 - 458
Copyright
Copyright © Australian Mathematical Society 1999

References

[1]Attouch, H., Variational convergence for functions and operators, Applicable Mathematics Series (Pitman, London, 1984).Google Scholar
[2]Attouch, H. and Wets, R.J.–B., ‘Quantitative stability of variational systems: III. ε–approximate solutions’, Math. Programming 61 (1993), 197–214.Google Scholar
[3]Attouch, H. and Brézis, H., ‘Duality for the sum of convex functions in general Banach spaces’, in Aspects of Mathematics and its Applications, (Barroso, J., Editor) (Elsevier Science. Publishers, 1986), pp. 125–133.Google Scholar
[4]Aubin, J.–P. and Frankowska, H., Set–valued analysis, Systems and Control: Foundations and Applications 2 (Birkhäuser, Boston, MA, 1990).Google Scholar
[5]Azé, D. and Penot, J.–P., ‘Operations on convergent families of sets and functions’, Optimization 21 (1990), 521–534.CrossRefGoogle Scholar
[6]Beer, C., ‘The slice topology: A viable alternative to Mosco convergence in non–reflexive spaces’, Nonlinear Anal. 19 (1992), 271–290.CrossRefGoogle Scholar
[7]Beer, C., Topologies on closed and closed convex sets, Mathematics and its Applications 268 (Kluwer Acad. Publ., Dordrecht, 1993).Google Scholar
[8]Beer, G. and Borwein, M., ‘Mosco convergence and reflexivity’, Proc. Amer. Math. Soc. 109 (1990), 427–436.Google Scholar
[9]Beer, G. and Lucchetti, R., ‘The Epi–distance topology: Continuity and stability results with applications to convex optimization problems’, Math. Oper. Res. 17 (1992), 715–726.CrossRefGoogle Scholar
[10]Borwein, J.M., ‘Epi–Lipschitz-like sets in Banach space: Theorems and examples’, Nonlinear Anal. 11 (1987), 1207–1217.Google Scholar
[11]Borwein, J.M. and Lewis, A.S., ‘Partially–finite convex programming’, Math. Programming 57 (1992), 15–83.Google Scholar
[12]Dahleh, M.A. and Diaz-Bobillo, I.J., Control of uncertain systems: A linear programming approach (Prentice–Hall, New Jersey, 1995).Google Scholar
[13]Desoer, C.A. and Vidyasagar, M., Feedback systems: Input–output properties (Academic Press, New York, London, 1975).Google Scholar
[14]Dunford, N. and Schwartz, J.T., Linear operators I, General theory, Wiley–Interscience (J. Wiley and Sons, New York, 1957).Google Scholar
[15]Elia, N. and Dahleh, M. A., ‘Controller design with multiple objectives’, IEEE Trans. Automat. Control AC-42 (1997), 596–613.CrossRefGoogle Scholar
[16]Holmes, R.B., Geometric functional analysis and its applications, Graduate Texts in Mathematics 24 (Springer-Verlag, Berlin, Heidelberg, New York, 1975).Google Scholar
[17]Jourani, A., ‘Compactly Epi–Lipschitzian sets and A–subdifferentials in WT–spaces’, optimization 34 (1995), 1–17.CrossRefGoogle Scholar
[18]Loewen, P.D., ‘Limits of Fréchet normals in nonsmooth analysis’, in Optimization and Nonsmooth Analysis, (Ioffe, , Marcus, , Reich, , Editors), Research Notes in Mathematics Series 244 (Longman Sci. and Tech., Harlow 1990), pp. 177–188.Google Scholar
[19]Penot, J.–P., ‘Preservation of persistence and stability under intersection and operations’, J. Optim. Theory Appl. 79 (1993), 525–561.CrossRefGoogle Scholar
[20]Rockafellar, R.T. and Wets, J.–B., ‘Variational systems, an introduction’, in Multi-functions and integrands, (Salinetti, G., Editor), Lecture Notes in Mathematics 1091 (Springer-Verlag, Berlin, Heidelberg, New York, 1984), pp. 1–54.Google Scholar
[21]Rockafellar, R.T., ‘Level–sets and continuity of conjugate convex functions’, Trans. Amer. Math. Soc. 123 (1966), 46–61.CrossRefGoogle Scholar
[22]Strömberg, T., ‘The operation of infimal convolution’, Dissertationes Math. 352 (1996), 4–58.Google Scholar
[23]Vidyasagar, M., Control system synthesis (MIT Press, Cambridge, 1985).Google Scholar
[24]Zabell, S.L., ‘Mosco convergence in locally convex spaces’, J. Funct. Anal. 110 (1992), 226–246.CrossRefGoogle Scholar