Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-26T01:00:01.958Z Has data issue: false hasContentIssue false
  • English
  • Français

SINGULARITY OF ORIENTED GRAPHS FROM SEVERAL CLASSES

Part of: Graph theory

Published online by Cambridge University Press:  21 November 2019

XIAOXUAN CHEN Open the ORCID record for XIAOXUAN CHEN [Opens in a new window] ,
JING YANG Open the ORCID record for JING YANG [Opens in a new window] ,
XIANYA GENG Open the ORCID record for XIANYA GENG [Opens in a new window]  and
LONG WANG Open the ORCID record for LONG WANG [Opens in a new window]
XIAOXUAN CHEN
Affiliation:
School of Mathematics and Big Data, Anhui University of Science and Technology, Huainan, China email [email protected]
JING YANG*
Affiliation:
School of Mathematics and Big Data, Anhui University of Science and Technology, Huainan, China email [email protected]
XIANYA GENG
Affiliation:
School of Mathematics and Big Data, Anhui University of Science and Technology, Huainan, China email [email protected]
LONG WANG
Affiliation:
School of Mathematics and Big Data, Anhui University of Science and Technology, Huainan, China email [email protected]
*
✉[email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

A digraph is called oriented if there is at most one arc between two distinct vertices. An oriented graph 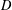$D$ is nonsingular if its adjacency matrix 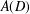$A(D)$ is nonsingular. We characterise all nonsingular oriented graphs from three classes: graphs in which cycles are vertex disjoint, graphs in which all cycles share exactly one common vertex and graphs formed by cycles sharing a common path. As a straightforward corollary, the singularity of oriented bicyclic graphs is determined.

Keywords

oriented graphsingularityrank

MSC classification

Primary: 05C50: Graphs and linear algebra (matrices, eigenvalues, etc.)
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 102 , Issue 1 , August 2020 , pp. 7 - 14
Check for updates
Copyright
© 2019 Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Supported by National Natural Science Foundation of China (61702008, 11701008), Natural Science Foundation of Anhui Province (1808085MF193, 1808085QA04, 1908085QA31), Educational Commission of Anhui Province of China (KJ2018A0081) and Research Program of Outstanding Young Backbone Talents in Colleges and Universities of Anhui Province (GXGWFX2019015).

References

Cheng, B. and Liu, B., ‘On the nullity of tricyclic graphs’, Linear Algebra Appl. 434(2011) 1799–1810.10.1016/j.laa.2011.01.006CrossRefGoogle Scholar
Collatz, L. and Sinogowitz, U., ‘Spektren endlicher Grafen’, Abh. Math. Semin. Univ. Hambg. 21(1957) 63–77.CrossRefGoogle Scholar
Cvetković, D., Doob, M. and Sachs, H., Spectra of Graphs: Theory and Application (Academic Press, New York, 1980).Google Scholar
Cvetković, D. and Gutman, I., ‘The algebraic multiplicity of the number zero in the spectrum of a bipartite graph’, Mat. Vesnik, Beograd 9(1972) 141–150.Google Scholar
Cvetković, D., Gutman, I. and Trinajstić, N., ‘Graph theory and molecular orbitals II’, Croat. Chem. Acta 44(1972) 365–374.Google Scholar
Fan, Y. and Qian, K., ‘On the nullity of bipartite graphs’, Linear Algebra Appl. 430(2009) 2943–2949.CrossRefGoogle Scholar
Guo, J., Yan, W. and Yeh, Y.-N., ‘On the nullity and the matching number of unicyclic graphs’, Linear Algebra Appl. 431(2009) 1293–1301.10.1016/j.laa.2009.04.026CrossRefGoogle Scholar
Gutman, I. and Borovćanin, B., ‘Nullity of graphs: an updated survey’, in: Selected Topics on Applications of Graph Spectra (eds. Cvetković, D. and Gutman, I.) (Math. Inst., Belgrade, 2011), 137–154.Google Scholar
Gutman, I. and Sciriha, I., ‘On the nullity of line graphs of trees’, Discrete Math. 232(2001) 35–45.10.1016/S0012-365X(00)00187-4CrossRefGoogle Scholar
Hu, S., Tan, X. and Liu, B., ‘On the nullity of bicyclic graphs’, Linear Algebra Appl. 429(2008) 1387–1391.10.1016/j.laa.2007.12.007CrossRefGoogle Scholar
Hückel, E., ‘Quantentheoretische Beiträge zum Benzolproblem’, Z. Phys. 70(1931) 204–286.10.1007/BF01339530CrossRefGoogle Scholar
Li, H., Fan, Y. and Su, L., ‘On the nullity of the line graph of unicyclic graph with depth one’, Linear Algebra Appl. 437(2012) 2038–2055.CrossRefGoogle Scholar
Monsalve, J. and Rada, J., ‘Oriented bipartite graphs with minimal trace norm’, Linear Multilinear 67(6) (2019), 1121–1131.10.1080/03081087.2018.1448051CrossRefGoogle Scholar
Zhang, Y., Xu, F. and Wong, D., ‘Characterization of oriented graphs of rank 2’, Linear Algebra Appl. 579(2019) 136–147.CrossRefGoogle Scholar