Hostname: page-component-586b7cd67f-t7czq Total loading time: 0 Render date: 2024-11-27T23:54:57.875Z Has data issue: false hasContentIssue false
  • English
  • Français

A SHORT NOTE ON THE FRAME SET OF ODD FUNCTIONS

Part of: Nontrigonometric harmonic analysis General quantum mechanics and problems of quantization

Published online by Cambridge University Press:  15 August 2018

MARKUS FAULHUBER Open the ORCID record for MARKUS FAULHUBER [Opens in a new window]
MARKUS FAULHUBER*
Affiliation:
Analysis Group, Department of Mathematical Sciences, NTNU Trondheim, Sentralbygg 2, Gløshaugen, Trondheim, Norway email [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We give a simple argument which shows that Gabor systems consisting of odd functions of $d$ variables and symplectic lattices of density 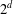$2^{d}$ cannot constitute a Gabor frame. In the one-dimensional, separable case, this follows from a more general result of Lyubarskii and Nes [‘Gabor frames with rational density’, Appl. Comput. Harmon. Anal.34(3) (2013), 488–494]. We use a different approach exploiting the algebraic relation between the ambiguity function and the Wigner distribution as well as their relation given by the (symplectic) Fourier transform. Also, we do not need the assumption that the lattice is separable and, hence, new restrictions are added to the full frame set of odd functions.

Keywords

ambiguity functionFeichtinger’s algebraframe setGabor frameWigner distribution

MSC classification

Primary: 42C15: General harmonic expansions, frames
Secondary: 81S30: Phase-space methods including Wigner distributions, etc.
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 98 , Issue 3 , December 2018 , pp. 481 - 493
Copyright
© 2018 Australian Mathematical Publishing Association Inc. 

Footnotes

The author was supported by the Erwin Schrödinger program of the Austrian Science Fund (FWF): J4100-N32.

References

Christensen, O., An Introduction to Frames and Riesz Bases, 2nd edn, Applied and Numerical Harmonic Analysis (Birkhäuser, Basel, 2016).Google Scholar
Faulhuber, M., ‘Minimal frame operator norms via minimal theta functions’, J. Fourier Anal. Appl. 24(2) (2018), 545–559.Google Scholar
Faulhuber, M. and Steinerberger, S., ‘Optimal Gabor frame bounds for separable lattices and estimates for Jacobi theta functions’, J. Math. Anal. Appl. 445(1) (2017), 407–422.Google Scholar
Feichtinger, H. G., ‘On a new Segal algebra’, Monatsh. Math. 92(4) (1981), 269–289.Google Scholar
Folland, G. B., Harmonic Analysis in Phase Space, Annals of Mathematics Studies, 122 (Princeton University Press, Princeton, NJ, 1989).Google Scholar
de Gosson, M. A., Symplectic Methods in Harmonic Analysis and in Mathematical Physics, Pseudo-Differential Operators. Theory and Applications, 7 (Birkhäuser/Springer, Basel, 2011).Google Scholar
de Gosson, M. A., The Wigner Transform (World Scientific, Singapore, 2017).Google Scholar
Gröchenig, K., ‘An uncertainty principle related to the Poisson summation formula’, Studia Math. 121(1) (1996), 87–104.Google Scholar
Gröchenig, K., Foundations of Time–Frequency Analysis, Applied and Numerical Harmonic Analysis (Birkhäuser, Boston, MA, 2001).Google Scholar
Gröchenig, K., ‘The mystery of Gabor frames’, J. Fourier Anal. Appl. 20(4) (2014), 865–895.Google Scholar
Jakobsen, M. S., ‘On a (no longer) new Segal algebra: a review of the Feichtinger algebra’, J. Fourier Anal. Appl. (2018), 1–82; published online https://doi.org/10.1007/s00041-018-9596-4.Google Scholar
Janssen, A. J. E. M., ‘Duality and biorthogonality for Weyl–Heisenberg frames’, J. Fourier Anal. Appl. 1(4) (1995), 403–436.Google Scholar
Janssen, A. J. E. M., ‘Some Weyl–Heisenberg frame bound calculations’, Indag. Math. (N.S.) 7(2) (1996), 165–183.Google Scholar
Lanzara, F. and Maz’ya, V., ‘Note on a nonstandard eigenfunction of the planar Fourier transform’, J. Math. Sci. 224(5) (2017), 694–698.Google Scholar
Lemvig, J., ‘On some Hermite series identities and their applications to Gabor analysis’, Monatsh. Math. 182(4) (2017), 889–912.Google Scholar
Lyubarskii, Y. and Nes, P. G., ‘Gabor frames with rational density’, Appl. Comput. Harmon. Anal. 34(3) (2013), 488–494.Google Scholar
Pei, S.-C. and Liu, C.-L., ‘A general form of 2D Fourier transform eigenfunctions’, in: 2012 IEEE Int. Conf. Acoustics, Speech and Signal Processing (ICASSP) (IEEE, New York, 2012), 3701–3704.Google Scholar
Tolimieri, R. and Orr, R. S., ‘Poisson summation, the ambiguity function, and the theory of Weyl–Heisenberg frames’, J. Fourier Anal. Appl. 1(3) (1995), 233–247.Google Scholar