THE PROBABILITY THAT 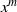$\lowercase {X}^{\lowercase {M}}$ AND $\lowercase {Y}^{\lowercase {N}}$ COMMUTE IN A COMPACT GROUP

Abstract

In a recent article [K. H. Hofmann and F. G. Russo, ‘The probability that $x$ and 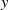$y$ commute in a compact group’, Math. Proc. Cambridge Phil Soc., to appear] we calculated for a compact group 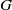$G$ the probability 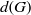$d(G)$ that two randomly selected elements 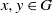$x, y\in G$ satisfy $xy=yx$, and we discussed the remarkable consequences on the structure of 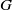$G$ which follow from the assumption that 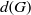$d(G)$ is positive. In this note we consider two natural numbers $m$ and $n$ and the probability 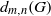$d_{m,n}(G)$ that for two randomly selected elements $x, y\in G$ the relation $x^my^n=y^nx^m$ holds. The situation is more complicated whenever 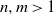$n,m\gt 1$. If 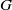$G$ is a compact Lie group and if its identity component 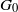$G_0$ is abelian, then it follows readily that 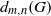$d_{m,n}(G)$ is positive. 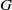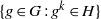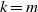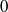We show here that the following condition suffices for the converse to hold in an arbitrary compact group $G$: for any nonopen closed subgroup $H$ of $G$, the sets $\{g\in G: g^k\in H\}$ for both $k=m$ and $k=n$ have Haar measure $0$. Indeed, we show that if a compact group $G$ satisfies this condition and if 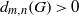$d_{m,n}(G)\gt 0$, then the identity component of 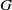$G$is abelian.