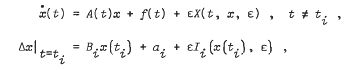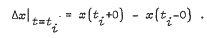Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Hu, Shouchuan
and
Lakshmikantham, V.
1989.
Periodic boundary value problems for second order impulsive differential systems.
Nonlinear Analysis: Theory, Methods & Applications,
Vol. 13,
Issue. 1,
p.
75.
Liz, E.
and
Nieto, Juan J.
1991.
Periodic solutions of discontinuous impulsive differential systems.
Journal of Mathematical Analysis and Applications,
Vol. 161,
Issue. 2,
p.
388.
Simeonov, P.S
and
Bainov, D.D
1991.
On the asymptotic properties of the solutions of systems of differential equations with impulse effect.
Journal of Mathematical Analysis and Applications,
Vol. 158,
Issue. 1,
p.
1.
Rachůnková, Irena
and
Tomeček, Jan
2015.
State-Dependent Impulses.
Vol. 6,
Issue. ,
p.
1.
Peng, Yunfei
Wu, Kuilin
Qin, Shulin
and
Kang, Ying
2017.
Properties of solution of linear controlled systems with impulses at variable times.
p.
811.
Fen, Mehmet Onur
and
Tokmak Fen, Fatma
2023.
Unpredictability in Quasilinear Non-Autonomous Systems with Regular Moments of Impulses.
Mediterranean Journal of Mathematics,
Vol. 20,
Issue. 4,