Hostname: page-component-6bf8c574d5-b4m5d Total loading time: 0 Render date: 2025-02-25T22:57:44.744Z Has data issue: false hasContentIssue false
  • English
  • Français

ON THE PRIMES IN FLOOR FUNCTION SETS

Part of: Multiplicative number theory Exponential sums and character sums

Published online by Cambridge University Press:  23 November 2022

RONG MA Open the ORCID record for RONG MA [Opens in a new window]  and
JIE WU Open the ORCID record for JIE WU [Opens in a new window]
RONG MA
Affiliation:
School of Mathematics and Statistics, Northwestern Polytechnical University, Xi’an, Shaanxi 710072, PR China e-mail: [email protected]
JIE WU*
Affiliation:
CNRS UMR 8050, Laboratoire d’Analyse et de Mathématiques Appliquées, Université Paris-Est Créteil, 94010 Créteil cedex, France
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Let $[t]$ be the integral part of the real number t and let 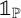$\mathbb {1}_{{\mathbb P}}$ be the characteristic function of the primes. Denote by 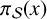$\pi _{\mathcal {S}}(x)$ the number of primes in the floor function set 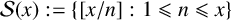$\mathcal {S}(x) := \{[{x}/{n}] : 1\leqslant n\leqslant x\}$ and by 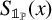$S_{\mathbb {1}_{{\mathbb P}}}(x)$ the number of primes in the sequence 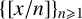$\{[{x}/{n}]\}_{n\geqslant 1}$. Improving a result of Heyman [‘Primes in floor function sets’, Integers 22 (2022), Article no. A59], we show

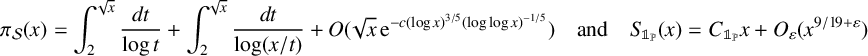$$ \begin{align*} \pi_{\mathcal{S}}(x) = \int_2^{\sqrt{x}} \frac{d t}{\log t} + \int_2^{\sqrt{x}} \frac{d t}{\log(x/t)} + O(\sqrt{x}\,\mathrm{e}^{-c(\log x)^{3/5}(\log\log x)^{-1/5}}) \quad\mbox{and}\quad S_{\mathbb{1}_{{\mathbb P}}}(x) = C_{\mathbb{1}_{{\mathbb P}}} x + O_{\varepsilon}(x^{9/19+\varepsilon}) \end{align*} $$

for 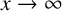$x\to \infty $, where 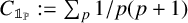$C_{\mathbb {1}_{{\mathbb P}}} := \sum _{p} {1}/{p(p+1)}$, 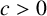$c>0$ is a positive constant and $\varepsilon $ is an arbitrarily small positive number.

Keywords

the prime number theoremexponential sumsexponent pairvon Mangoldt function

MSC classification

Primary: 11N37: Asymptotic results on arithmetic functions
Secondary: 11L07: Estimates on exponential sums
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 108 , Issue 2 , October 2023 , pp. 236 - 243
Check for updates
Copyright
© The Author(s), 2022. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

This work is supported in part by the National Natural Science Foundation of China (Grant Nos. 11971370 and 12071375).

References

Bordellès, O., ‘On certain sums of number theory’, Int. J. Number Theory 18(99) (2022), 2053–2074.10.1142/S1793042122501056CrossRefGoogle Scholar
Bordellès, O., Dai, L., Heyman, R., Pan, H. and Shparlinski, I. E., ‘On a sum involving the Euler function’, J. Number Theory 202 (2019), 278–297.10.1016/j.jnt.2019.01.006CrossRefGoogle Scholar
Davenport, H., Multiplicative Number Theory, 3rd edn, Graduate Texts in Mathematics, 74 (Springer-Verlag, New York, 2000), revised and with a preface by H. L. Montgomery.Google Scholar
Friedlander, J. and Iwaniec, H., ‘The polynomial ${X}^2+{Y}^4$ captures its primes’, Ann. of Math. (2) 148(3) (1998), 945–1040.10.2307/121034CrossRefGoogle Scholar
Heath-Brown, D. R., ‘Primes represented by ${x}^3+2{y}^3$ ’, Acta Math. 186(1) (2001), 1–84.10.1007/BF02392715CrossRefGoogle Scholar
Heyman, R., ‘Cardinality of a floor function set’, Integers 19 (2019), Article no. A67.Google Scholar
Heyman, R., ‘Primes in floor function sets’, Integers 22 (2022), Article no. A59.Google Scholar
Huxley, M. N., ‘On the difference between consecutive primes’, Invent. Math. 15 (1972), 164–170.10.1007/BF01418933CrossRefGoogle Scholar
Liu, K., Wu, J. and Yang, Z.-S., ‘On some sums involving the integral part function’, Preprint, 2021, arXiv:2109.01382v1.Google Scholar
Liu, K., Wu, J. and Yang, Z.-S., ‘A variant of the prime number theorem’, Indag. Math. (N.S.) 33 (2022), 388–396.10.1016/j.indag.2021.09.005CrossRefGoogle Scholar
Ma, J. and Wu, J., ‘On a sum involving the von Mangoldt function’, Period. Math. Hungar. 83(1) (2021), 39–48.10.1007/s10998-020-00359-6CrossRefGoogle Scholar
Rivat, J. and Sargos, P., ‘Nombres premiers de la forme $\left[{n}^c\right]$ ’, Canad. J. Math. 53(2) (2001), 414–433 (in French). English summary.10.4153/CJM-2001-017-0CrossRefGoogle Scholar
Wu, J., ‘Note on a paper by Bordellès, Dai, Heyman, Pan and Shparlinski’, Period. Math. Hungar. 80 (2020), 95–102.10.1007/s10998-019-00300-6CrossRefGoogle Scholar
Yu, Y. and Wu, J., ‘Distribution of elements of a floor function set in arithmetical progression’, Bull. Aust. Math. Soc. 106(3) (2022), 419–424.10.1017/S000497272200017XCrossRefGoogle Scholar
Zhai, W., ‘On a sum involving the Euler function’, J. Number Theory 211 (2020), 199–219.10.1016/j.jnt.2019.10.003CrossRefGoogle Scholar