Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-29T07:36:08.507Z Has data issue: false hasContentIssue false
  • English
  • Français

ON THE INCREASING PARTIAL QUOTIENTS OF CONTINUED FRACTIONS OF POINTS IN THE PLANE

Part of: Classical measure theory Probabilistic theory: distribution modulo $1$; metric theory of algorithms

Published online by Cambridge University Press:  17 December 2021

MEIYING LÜ Open the ORCID record for MEIYING LÜ [Opens in a new window]  and
ZHENLIANG ZHANG Open the ORCID record for ZHENLIANG ZHANG [Opens in a new window]
MEIYING LÜ
Affiliation:
School of Mathematical Sciences, Chongqing Normal University, Chongqing 401331, PR China e-mail: [email protected]
ZHENLIANG ZHANG*
Affiliation:
School of Mathematical Sciences, Chongqing Normal University, Chongqing 401331, PR China
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

For any x in 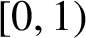$[0,1)$ , let 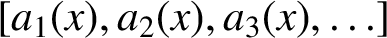$[a_1(x),a_2(x),a_3(x),\ldots ]$ be its continued fraction. Let 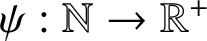$\psi :\mathbb {N}\to \mathbb {R}^+$ be such that 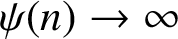$\psi (n) \to \infty $ as 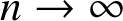$n\to \infty $ . For any positive integers s and t, we study the set

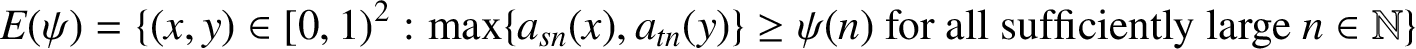$$ \begin{align*}E(\psi)=\{(x,y)\in [0,1)^2: \max\{a_{sn}(x), a_{tn}(y)\}\ge \psi(n) \ {\text{for all sufficiently large}}\ n\in \mathbb{N}\} \end{align*} $$

and determine its Hausdorff dimension.

Keywords

continued fractionpartial quotientHausdorff dimension

MSC classification

Primary: 11K55: Metric theory of other algorithms and expansions; measure and Hausdorff dimension
Secondary: 28A80: Fractals
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 105 , Issue 3 , June 2022 , pp. 404 - 411
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

This work was supported by the Program of Chongqing Municipal Education Commission (Nos. KJQN202100528 and KJQN202000531), Projects from Chongqing Municipal Science and Technology Commission (No. cstc2018jcyjAX0277) and the Foundation of Chongqing Normal University (No. 20XLB030).

References

Falconer, K. J., Fractal Geometry: Mathematical Foundations and Applications (Wiley, Hoboken, NJ, 1990).Google Scholar
Feng, D. J., Wu, J., Liang, J. C. and Tseng, S., ‘A simple proof of the lower bound on the fractional dimension of sets of continued fractions’, Mathematika 44(1) (1997), 54–55, Appendix to the paper by T. Łuczak.Google Scholar
Good, I. J., ‘The fractional dimensional theory of continued fractions’, Math. Proc. Cambridge Philos. Soc. 37 (1941), 199–228.CrossRefGoogle Scholar
Hirst, K. E., ‘A problem in the fractional dimension theory of continued fractions’, Q. J. Math. 21(1) (1970), 29–35.CrossRefGoogle Scholar
Khintchine, A. Y., Continued Fractions (University of Chicago Press, Chicago, IL, 1964).Google Scholar
Łuczak, T., ‘On the fractional dimension of sets of continued fractions’, Mathematika 44(1) (1997), 50–53.CrossRefGoogle Scholar
Moorthy, C. G., ‘A problem of Good on Hausdorff dimension’, Mathematika 39(2) (1992), 244–246.CrossRefGoogle Scholar
Wang, B. W. and Wu, J., ‘Hausdorff dimension of certain sets arising in continued fraction expansions’, Adv. Math. 218(5) (2008), 1319–1339.CrossRefGoogle Scholar
Wu, J. and Xu, J., ‘The distribution of the largest digit in continued fraction expansions’, Math. Proc. Cambridge Philos. Soc. 146(1) (2009), 207–212.CrossRefGoogle Scholar