Hostname: page-component-f554764f5-68cz6 Total loading time: 0 Render date: 2025-04-20T16:33:17.750Z Has data issue: false hasContentIssue false
  • English
  • Français

ON THE EXPECTED UNIFORM ERROR OF BROWNIAN MOTION APPROXIMATED BY THE LÉVY–CIESIELSKI CONSTRUCTION

Part of: Markov processes

Published online by Cambridge University Press:  24 August 2023

BRUCE BROWN Open the ORCID record for BRUCE BROWN [Opens in a new window] ,
MICHAEL GRIEBEL Open the ORCID record for MICHAEL GRIEBEL [Opens in a new window] ,
FRANCES Y. KUO Open the ORCID record for FRANCES Y. KUO [Opens in a new window]  and
IAN H. SLOAN
BRUCE BROWN
Affiliation:
School of Mathematics and Statistics, University of New South Wales, Sydney 2052, Australia e-mail: [email protected]
MICHAEL GRIEBEL
Affiliation:
Institut für Numerische Simulation, Universität Bonn, Bonn, Germany and Fraunhofer Institute SCAI, Schloss Birlinghoven, Sankt Augustin, Germany e-mail: [email protected]
FRANCES Y. KUO
Affiliation:
School of Mathematics and Statistics, University of New South Wales, Sydney 2052, Australia e-mail: [email protected]
IAN H. SLOAN*
Affiliation:
School of Mathematics and Statistics, University of New South Wales, Sydney 2052, Australia
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The Brownian bridge or Lévy–Ciesielski construction of Brownian paths almost surely converges uniformly to the true Brownian path. We focus on the uniform error. In particular, we show constructively that at level N, at which there are $d=2^N$ points evaluated on the Brownian path, the uniform error and its square, and the uniform error of geometric Brownian motion, have upper bounds of order 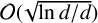$\mathcal {O}(\sqrt {\ln d/d})$, matching the known orders. We apply the results to an option pricing example.

Keywords

Brownian motionBrownian bridgeLévy–Ciesielski constructionexpected uniform error

MSC classification

Primary: 60J65: Brownian motion
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 109 , Issue 3 , June 2024 , pp. 581 - 593
Check for updates
Copyright
© The Author(s), 2023. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

Footnotes

The authors acknowledge support of the Australian Research Council under project DP210100831. Michael Griebel acknowledges support from the Sydney Mathematical Research Institute.

References

Bogachev, V. I., Gaussian Measures (American Mathematical Society, Providence, RI, 1998).CrossRefGoogle Scholar
Ciesielski, Z., ‘Hölder conditions for realizations of Gaussian processes’, Trans. Amer. Math. Soc. 99 (1961), 403–413.Google Scholar
Griebel, M., Kuo, F. Y. and Sloan, I. H., ‘The ANOVA decomposition of a non-smooth function of infinitely many variables can have every term smooth’, Math. Comp. 86 (2017), 1855–1876.CrossRefGoogle Scholar
Lévy, P., Processus stochastiques et mouvement brownien, Suivi d’une note de M. Loève (Gauthier-Villars, Paris, 1948) (in French).Google Scholar
Müller-Gronbach, T., ‘The optimal uniform approximation of systems of stochastic differential equations’, Ann. Appl. Probab. 12 (2002), 664–690.CrossRefGoogle Scholar
Ritter, K., ‘Approximation and optimization on the Wiener space’, J. Complexity 6 (1990), 337–364.CrossRefGoogle Scholar
Small, C. G., Expansions and Asymptotics for Statistics (CRC Press, Boca Raton, FL, 2010).CrossRefGoogle Scholar
Steele, J. M., Stochastic Calculus and Financial Applications, Applications of Mathematics, 45 (Springer-Verlag, New York, 2001).CrossRefGoogle Scholar
Triebel, H., Bases in Function Spaces, Sampling, Discrepancy, Numerical Integration, EMS Tracts in Mathematics, 11 (European Mathematical Society, Zurich, 2010).CrossRefGoogle Scholar
Triebel, H., ‘Numerical integration and discrepancy, a new approach’, Math. Nachr. 283 (2010), 139–159.CrossRefGoogle Scholar