No CrossRef data available.
Article contents
ON THE EVANS CHAIN COMPLEX
Part of:
Selfadjoint operator algebras
Published online by Cambridge University Press: 14 October 2024
Abstract
We elaborate on the construction of the Evans chain complex for higher-rank graph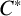 
$C^*$-algebras. Specifically, we introduce a block matrix presentation of the differential maps. These block matrices are then used to identify a wide family of higher-rank graph 
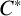$C^*$-algebras with trivial K-theory. Additionally, in the specialised case where the higher-rank graph consists of one vertex, we are able to use the Künneth theorem to explicitly compute the homology groups of the Evans chain complex.
MSC classification
Secondary:
46L37: Subfactors and their classification
- Type
- Research Article
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press on behalf of Australian Mathematical Publishing Association Inc.
References
Barlak, S., Omland, T. and Stammeier, N., ‘On the
$K$
-theory of
${C}^{\ast }$
-algebras arising from integral dynamics’, Ergodic Theory Dynam. Systems 38(3) (2018), 832–862.CrossRefGoogle Scholar
$K$
-theory of
${C}^{\ast }$
-algebras arising from integral dynamics’, Ergodic Theory Dynam. Systems 38(3) (2018), 832–862.CrossRefGoogle ScholarElliott, G. A., Gong, G., Lin, H. and Niu, Z., ‘The classification of simple separable KK-contractible
$\mathrm{C}^{\ast }$
-algebras with finite nuclear dimension’, J. Geom. Phys. 158 (2020), Article no. 103861, 51 pages.CrossRefGoogle Scholar
$\mathrm{C}^{\ast }$
-algebras with finite nuclear dimension’, J. Geom. Phys. 158 (2020), Article no. 103861, 51 pages.CrossRefGoogle ScholarEvans, D. G., ‘On the
$K$
-theory of higher rank graph
${C}^{\ast }$
-algebras,’ New York J. Math. 14 (2008), 1–31.Google Scholar
$K$
-theory of higher rank graph
${C}^{\ast }$
-algebras,’ New York J. Math. 14 (2008), 1–31.Google ScholarGong, G., Lin, H. and Niu, Z., ‘A classification of finite simple amenable
$Z$
-stable
${C}^{\ast }$
-algebras, I:
${C}^{\ast }$
-algebras with generalized tracial rank one’, C. R. Math. Acad. Sci. Soc. R. Can. 42(3) (2020), 63–450.Google Scholar
$Z$
-stable
${C}^{\ast }$
-algebras, I:
${C}^{\ast }$
-algebras with generalized tracial rank one’, C. R. Math. Acad. Sci. Soc. R. Can. 42(3) (2020), 63–450.Google ScholarGong, G., Lin, H. and Niu, Z., ‘A classification of finite simple amenable
$Z$
-stable
${C}^{\ast }$
-algebras, II:
${C}^{\ast }$
-algebras with rational generalized tracial rank one’, C. R. Math. Acad. Sci. Soc. R. Can. 42(4) (2020), 451–539.Google Scholar
$Z$
-stable
${C}^{\ast }$
-algebras, II:
${C}^{\ast }$
-algebras with rational generalized tracial rank one’, C. R. Math. Acad. Sci. Soc. R. Can. 42(4) (2020), 451–539.Google ScholarHajac, P. M., Nest, R., Pask, D., Sims, A. and Zieliński, B., ‘The
$K$
-theory of twisted multipullback quantum odd spheres and complex projective spaces’, J. Noncommut. Geom. 12(3) (2018), 823–863.CrossRefGoogle Scholar
$K$
-theory of twisted multipullback quantum odd spheres and complex projective spaces’, J. Noncommut. Geom. 12(3) (2018), 823–863.CrossRefGoogle ScholarKasparov, G. G., ‘Equivariant KK-theory and the Novikov conjecture’, Invent. Math. 91(1) (1988), 147–201.CrossRefGoogle Scholar
Kirchberg, E., ‘Exact
${C}^{\ast }$
-algebras, tensor products, and the classification of purely infinite algebras’, in: Proceedings of the International Congress of Mathematicians (Zürich, 1994), Vols. 1, 2 (ed. S. D. Chatterji) (Birkhäuser, Basel, 1995), 943–954.CrossRefGoogle Scholar
${C}^{\ast }$
-algebras, tensor products, and the classification of purely infinite algebras’, in: Proceedings of the International Congress of Mathematicians (Zürich, 1994), Vols. 1, 2 (ed. S. D. Chatterji) (Birkhäuser, Basel, 1995), 943–954.CrossRefGoogle ScholarKirchberg, E. and Phillips, N. C., ‘Embedding of exact
${C}^{\ast }$
-algebras in the Cuntz algebra
${O}_2$
’, J. reine angew. Math. 525 (2000), 17–53.CrossRefGoogle Scholar
${C}^{\ast }$
-algebras in the Cuntz algebra
${O}_2$
’, J. reine angew. Math. 525 (2000), 17–53.CrossRefGoogle ScholarKumjian, A. and Pask, D., ‘Higher rank graph
${C}^{\ast }$
-algebras’, New York J. Math. 6 (2000), 1–20.Google Scholar
${C}^{\ast }$
-algebras’, New York J. Math. 6 (2000), 1–20.Google ScholarMassey, W. S., Singular Homology Theory, Graduate Texts in Mathematics, 70 (Springer-Verlag, New York–Berlin, 1980).CrossRefGoogle Scholar
Robertson, G. and Steger, T., ‘Affine buildings, tiling systems and higher rank Cuntz–Krieger algebras’, J. reine angew. Math. 513 (1999), 115–144.CrossRefGoogle Scholar
Robertson, G. and Steger, T., ‘Asymptotic
$K$
-theory for groups acting on
$\widetilde{A}_2$
buildings’, Canad. J. Math. 53(4) (2001), 809–833.CrossRefGoogle Scholar
$K$
-theory for groups acting on
$\widetilde{A}_2$
buildings’, Canad. J. Math. 53(4) (2001), 809–833.CrossRefGoogle ScholarRuiz, E., Sims, A. and Sørensen, A. P. W., ‘UCT-Kirchberg algebras have nuclear dimension one’, Adv. Math. 279 (2015), 1–28.CrossRefGoogle Scholar
Schochet, C., ‘Topological methods for
${C}^{\ast }$
-algebras. I. Spectral sequences’, Pacific J. Math. 96(1) (1981), 193–211.CrossRefGoogle Scholar
${C}^{\ast }$
-algebras. I. Spectral sequences’, Pacific J. Math. 96(1) (1981), 193–211.CrossRefGoogle ScholarVdovina, A., ‘Drinfeld–Manin solutions of the Yang–Baxter equation coming from cube complexes’, Internat. J. Algebra Comput. 31(4) (2021), 775–788.CrossRefGoogle Scholar
Weibel, C. A., An Introduction to Homological Algebra, Cambridge Studies in Advanced Mathematics, 38 (Cambridge University Press, Cambridge, 1994).CrossRefGoogle Scholar
Yang, D.. ‘The interplay between
$k$
-graphs and the Yang–Baxter equation’, J. Algebra 451 (2016), 494–525.CrossRefGoogle Scholar
$k$
-graphs and the Yang–Baxter equation’, J. Algebra 451 (2016), 494–525.CrossRefGoogle Scholar