Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-28T19:00:05.748Z Has data issue: false hasContentIssue false
  • English
  • Français

ON THE DISTRIBUTION OF TORSION POINTS MODULO PRIMES

Part of: Multiplicative number theory Algebraic number theory: global fields Arithmetic algebraic geometry

Published online by Cambridge University Press:  16 February 2012

YEN-MEI J. CHEN  and
YEN-LIANG KUAN
YEN-MEI J. CHEN*
Affiliation:
Department of Mathematics, National Central University, Jhongli City, Taoyuan County 32001, Taiwan (email: [email protected])
YEN-LIANG KUAN
Affiliation:
Department of Mathematics, National Central University, Jhongli City, Taoyuan County 32001, Taiwan (email: [email protected])
*
✉For correspondence; e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let be a commutative algebraic group defined over a number field K. For a prime ℘ in K where has good reduction, let N℘,n be the number of n-torsion points of the reduction of modulo ℘ where n is a positive integer. When is of dimension one and n is relatively prime to a fixed finite set of primes depending on 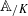, we determine the average values of N℘,n as the prime ℘ varies. This average value as a function of n always agrees with a divisor function.

Keywords

number fieldsalgebraic groupstorsion pointselliptic curvescomplex multiplication

MSC classification

Secondary: 11N13: Primes in progressions 11R18: Cyclotomic extensions 11G05: Elliptic curves over global fields
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 86 , Issue 2 , October 2012 , pp. 339 - 347
Copyright
Copyright © Australian Mathematical Publishing Association Inc. 2012

Footnotes

Research partially supported by National Science Council, Republic of China.

References

[1]Coates, J. and Wiles, A., ‘On the conjecture of Birch and Swinnerton-Dyer’, Invent. Math. 39 (1977), 223–251.CrossRefGoogle Scholar
[2]Cojocaru, A. C., ‘On the surjectivity of the Galois representations associated to non-CM elliptic curves’, Canad. Math. Bull. 48(1) (2005), 16–31.CrossRefGoogle Scholar
[3]Gupta, R., ‘Ramification in the Coates-Wiles tower’, Invent. Math. 81 (1985), 59–69.Google Scholar
[4]Lang, S., Algebraic Number Theory (Springer, Berlin, 1994).Google Scholar
[5]Murty, M. R., ‘On the supersingular reduction of elliptic curves’, Proc. Indian Acad. Sci. Math. Sci. 97 (1987), 247–250.CrossRefGoogle Scholar
[6]Serre, J.-P., ‘Propriétés galoisiennes des points d’ordre fini des courbes elliptiques’, Invent. Math. 15 (1972), 259–331.CrossRefGoogle Scholar
[7]Serre, J.-P., ‘On a theorem of Jordan’, Bull. Amer. Math. Soc. (N.S) 40(4) (2003), 429–440.CrossRefGoogle Scholar
[8]Silverman, J. H., Advanced Topics in the Arithmetic of Elliptic Curves (Springer, Berlin, 1994).CrossRefGoogle Scholar