Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-03T00:12:04.345Z Has data issue: false hasContentIssue false
  • English
  • Français

On the critical points of a polynomial

Published online by Cambridge University Press:  17 April 2009

Abdul Aziz  and
B.A. Zargar
Abdul Aziz
Affiliation:
Postgraduate Department of MathematicsUniversity of KashmirHazratbalSrinigar 190006India
B.A. Zargar
Affiliation:
Postgraduate Department of MathematicsUniversity of KashmirHazratbalSrinigar 190006India
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let p be a complex polynomial, of the form , where |zk| ≥ 1 when 1 ≤ k ≤ n − 1. Then p′(z) ≠ 0 if |z| /n.

Let B(z, r) denote the open ball in with centre z and radius r, and denote its closure. The Gauss-Lucas theorem states that every critical point of a complex polynomial p of degree at least 2 lies in the convex hull of its zeros. This theorem has been further investigated and developed. B. Sendov conjectured that, if all the zeros of p lie in 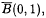then, for any zero ζ of p, the disc contains at least one zero of p′; see [3, Problem 4.1]. This conjecture has attracted much attention-see, for example, [1], and the papers cited there. In connection with this conjecture, Brown [2] posed the following problem.

Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 57 , Issue 1 , February 1998 , pp. 173 - 174
Copyright
Copyright © Australian Mathematical Society 1998

References

[1]Borcea, Iulius, ‘On the Sendov conjecture for polynomials with at most six distinct roots’, J. Math. Anal. Appl. 200 (1996), 182–206.CrossRefGoogle Scholar
[2]Brown, J.E., ‘On the Ilief-Sendov conjecture’, Pacific J. Math. 135 (1988), 223–232.CrossRefGoogle Scholar
[3]Hayman, W.K., Research problems in function theory (Athlone Press, London, 1967).Google Scholar