Hostname: page-component-586b7cd67f-rdxmf Total loading time: 0 Render date: 2024-11-27T15:01:18.996Z Has data issue: false hasContentIssue false
  • English
  • Français

ON THE CLASS NUMBER AND THE FUNDAMENTAL UNIT OF THE REAL QUADRATIC FIELD

Part of: Algebraic number theory: global fields

Published online by Cambridge University Press:  25 January 2012

JAE MOON KIM  and
JADO RYU
JAE MOON KIM
Affiliation:
Department of Mathematics, Inha University, Incheon 402-751, Korea (email: [email protected])
JADO RYU*
Affiliation:
Department of Mathematics, Inha University, Incheon 402-751, Korea (email: [email protected])
*
✉For correspondence; e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

For a real quadratic field 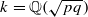, let tk be the exact power of 2 dividing the class number hk of k and ηk the fundamental unit of k. The aim of this paper is to study tk and the value of Nk/ℚ(ηk). Various methods have been successfully applied to obtain results related to this topic. The idea of our work is to select a special circular unit ℰk of k and investigate C(k)=〈±ℰk 〉. We examine the indices [E(k):C(k)] and [C(k):CS (k)] , where E(k) is the group of units of k, and CS (k) is that of circular units of k defined by Sinnott. Then by using the Sinnott’s index formula [E(k):CS (k)]=hk, we obtain as much information about tk and Nk/ℚ (ηk) as possible.

Keywords

class numberfundamental unitcircular unitunit indexindex formula

MSC classification

Secondary: 11R11: Quadratic extensions
Type
Research Article
Information
Bulletin of the Australian Mathematical Society , Volume 85 , Issue 3 , June 2012 , pp. 359 - 370
Copyright
Copyright © Australian Mathematical Publishing Association Inc. 2012

Footnotes

This work was supported by INHA UNIVERSITY Research Grant.

References

[1]Brown, E., ‘The class number and fundamental units of , for p≡1 (mod) 16 a prime’, J. Number Theory 16 (1983), 95–99.CrossRefGoogle Scholar
[2]Conner, P. E. and Hurrelbrink, J., Class Number Parity, Series in Pure Mathematics, 8 (World Science, Singapore, 1988).CrossRefGoogle Scholar
[3]Dohmae, K., ‘On bases of groups of circular units of some imaginary abelian number fields’, J. Number Theory 61 (1996), 343–364.CrossRefGoogle Scholar
[4]Dohmae, K., ‘A note on Sinnott’s index formula’, Acta Arith. 82(1) (1997), 57–67.CrossRefGoogle Scholar
[5]Hirabayashi, M. and Yoshino, K., ‘Remarks on unit indices of imaginary abelian fields’, Manuscripta Math. 60 (1988), 423–436.CrossRefGoogle Scholar
[6]Kim, J. and Ryu, J., ‘Construction of a certain circular unit and its applications’, J. Number Theory 131(4) (2011), 737–744.CrossRefGoogle Scholar
[7]Kučera, R., ‘On the parity of the class number of a biquadratic field’, J. Number Theory 52 (1995), 43–52.CrossRefGoogle Scholar
[8]Sinnott, W., ‘On the Stickelberger ideal and the circular units of an abelian field’, Invent. Math. 62 (1980), 181–234.CrossRefGoogle Scholar